The displacement of a particle of a string carrying a travelling wave is given by y=(3.0cm)sin6.28(0.50x−50t), where x is in centimetre and t in second Find (a) the amplitude, (b) the wavelength, (c ) the frequency and (d) the speed of the wave.
To solve the problem step by step, we will analyze the given wave equation and find the required parameters.
Given wave equation:
y=(3.0cm)sin(6.28(0.50x−50t))
Step 1: Identify the amplitude
The amplitude A of the wave is the coefficient in front of the sine function.
Solution:
From the equation, we see that:
A=3.0cm
Step 2: Find the wave number k and calculate the wavelength λ
The wave number k is given in the equation in the form kx. We can extract k from the equation.
Solution:
From the equation:
k=6.28×0.50=3.14cm−1
The wavelength λ is related to the wave number by the formula:
λ=2πk
Substituting the value of k:
λ=2π3.14≈2.0cm
Step 3: Find the angular frequency ω and calculate the frequency f
The angular frequency ω can be extracted from the equation as well.
Solution:
From the equation:
ω=6.28×50=314rad/s
The frequency f is related to the angular frequency by the formula:
f=ω2π
Substituting the value of ω:
f=3142π≈50Hz
Step 4: Calculate the speed of the wave v
The speed v of the wave can be calculated using the formula:
v=f⋅λ
Solution:
Substituting the values of f and λ:
v=50Hz×2.0cm=100cm/s
Summary of Results:
(a) Amplitude A=3.0cm
(b) Wavelength λ=2.0cm
(c) Frequency f=50Hz
(d) Speed v=100cm/s
---
y=(3.0cm)sin(6.28(0.50x−50t))
The amplitude A of the wave is the coefficient in front of the sine function.
From the equation, we see that:
A=3.0cm
The wave number k is given in the equation in the form kx. We can extract k from the equation.
From the equation:
k=6.28×0.50=3.14cm−1
λ=2πk
λ=2π3.14≈2.0cm
The angular frequency ω can be extracted from the equation as well.
From the equation:
ω=6.28×50=314rad/s
f=ω2π
f=3142π≈50Hz
The speed v of the wave can be calculated using the formula:
v=f⋅λ
Substituting the values of f and λ:
v=50Hz×2.0cm=100cm/s
(a) Amplitude A=3.0cm
(b) Wavelength λ=2.0cm
(c) Frequency f=50Hz
(d) Speed v=100cm/s
Topper's Solved these Questions
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Objective -1
20 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Objective -2
9 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer
7 Videos
- THE FORCESBOOK - HC VERMA ENGLISHCHAPTER - THE FORCESEXERCISE - Questions for short Answer
9 Videos
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer
16 Videos
Similar Questions
Figure shows a plot of the transverse displacements of the particles of a string at t = 0 through which a travelling wave is passing in the positive x-direction. The wave speed is 20cms−1. Find (a) the amplitude, (b) the wavelength, (c) the wave number and (d) the frequency of the wave.
 .
.
View SolutionThe displacement wave in a string is y=(3cm)sin6.28(0.5x−50t) where x is in centimetres and t in seconds. The velocity and wavelength of the wave is :-
View SolutionFigure shows a plot of the transverse displacement of the particle of a string at t=0 through which a travelling wave is passing in the positive in the positive x-direction. The wave speed is 20cm/s. Find (a) the amplitude (b) the wavelength (c) the wave number and (d) the frequency of the wave.
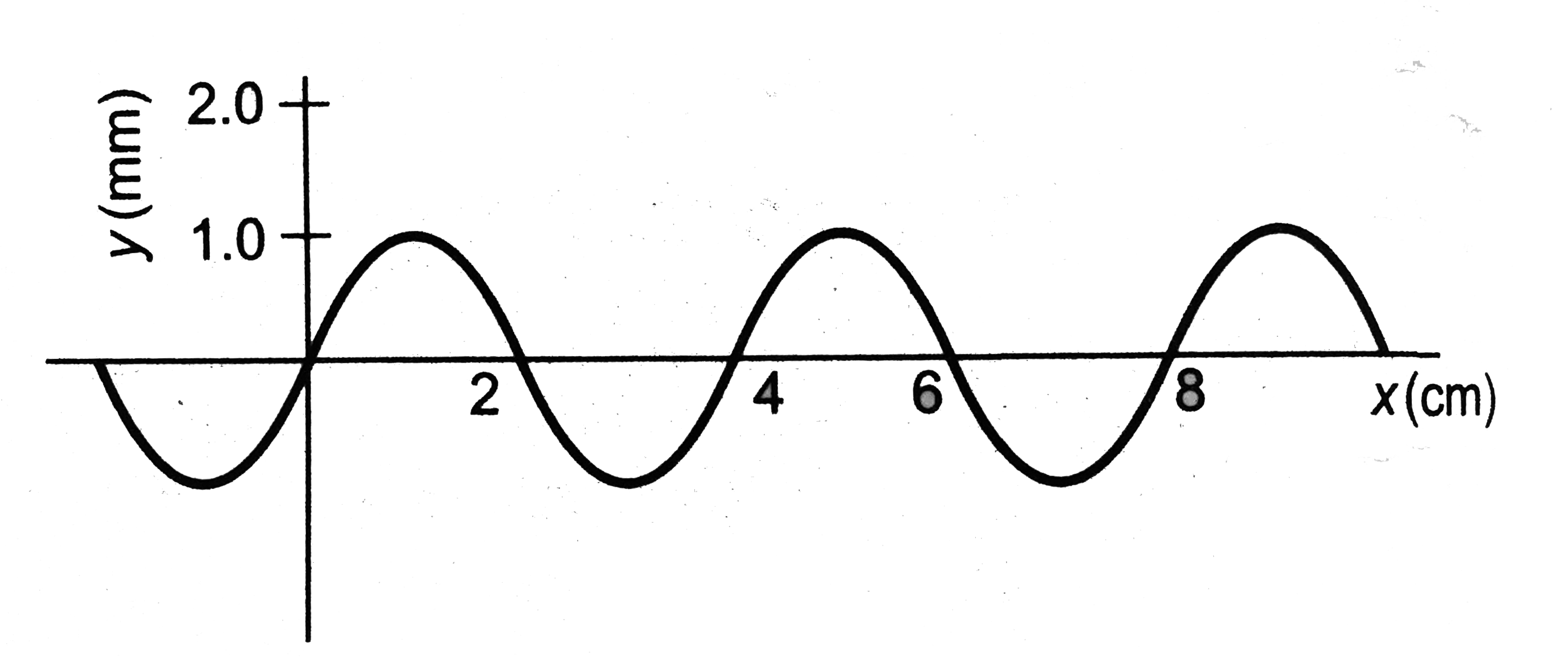
View SolutionThe equation of a transverse wave travelling along x-axis is given by y = 10 sin π= (0.01 x- 2.0t) where y and x are represented in centimeters and, in seconds. Find the amplitude, frequency velocity and wavelength of the wave.
View SolutionThe equation of a transverse wave is given by
y=10sinπ(0.01x−2t)
where x and y are in cm and t is in second. Its frequency is
View SolutionThe wave described by y =0.25sin(10πx−2πt) where x and y are in meters and t in seconds , is a wave travelling along the
View SolutionThe equation of a transverse wave travelling along +x axis is given by y=0.15 sin (1.57x-31.4t) where y and x are represented in m and t in s. Find the amplitude, frequency velocity and wavelength of the wave.
View SolutionThe wave described by y=0.25sin(10πx−2πt) , where x and y are in metres and t in seconds , is a wave travelling along the:
View SolutionTwo sinusoidal waves travelling in opposite directions interfere to produce a standing wave described by the equation
y=(1.5m)sin(0.400x)cos(200t)
where, x is in meters and t is in seconds. Determine the wavelength, frequency and speed of the interfering waves.
View SolutionTwo sinusoidal waves travelling in opposite directions interfere to produce a standing wave described by the equation y=(1.5)msin(0.200x)cos(100t), where x is in metres and t is in seconds. Determine the wavelength, frequency and speed of the interfering waves.
View Solution
