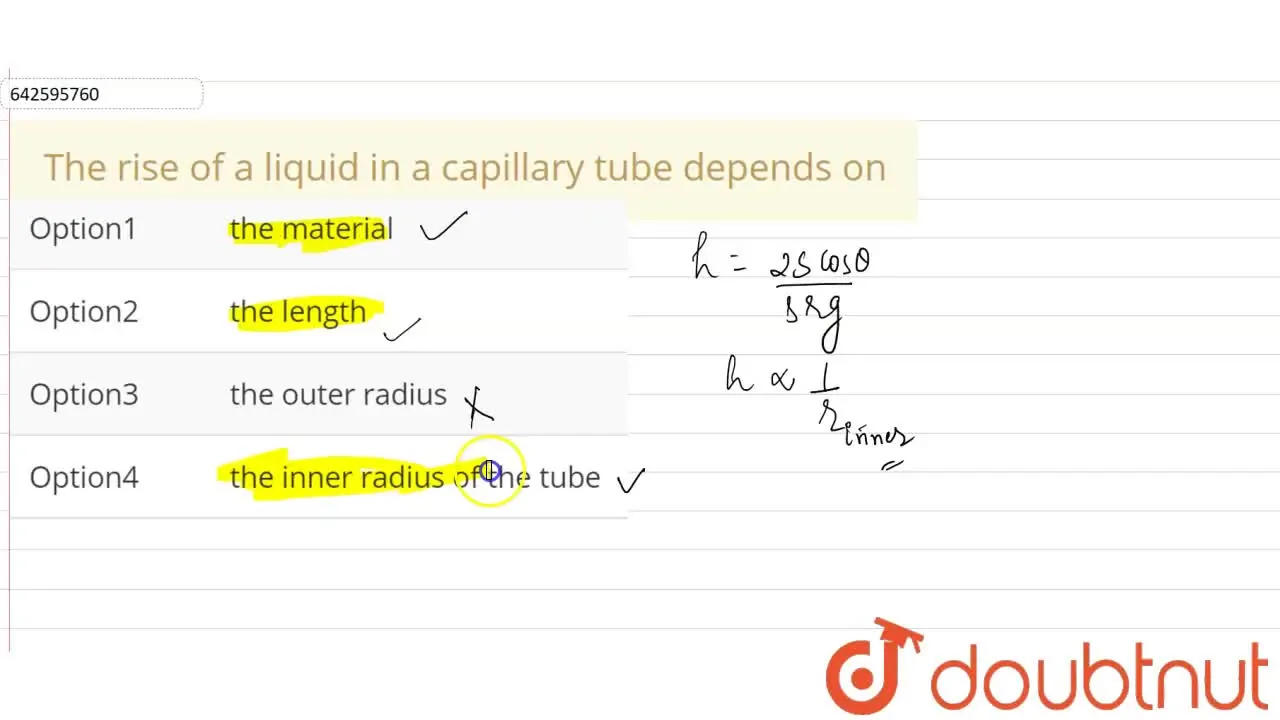
The rise of a liquid in a capillary tube depends on
the material
the length
the outer radius
the inner radius of the tube
Step by step video & image solution for The rise of a liquid in a capillary tube depends on by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-129videos
SOME MECHANICAL PROPERTIES OF MATTER
- BOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer15videos
SIMPLE HARMONIC MOTION
- BOOK - HC VERMA ENGLISHCHAPTER - SOUND WAVESEXERCISE - All Questions133videos
SOUND WAVES
Similar Questions
The rise of a liquid in a capillary tube is due to :
View SolutionWhat do you mean by capillarity. Derive relation for the rise of liquid in a capillary tube on the basis of balancing force and explain the rise of liquid in a tube of insufficient length.
View Solution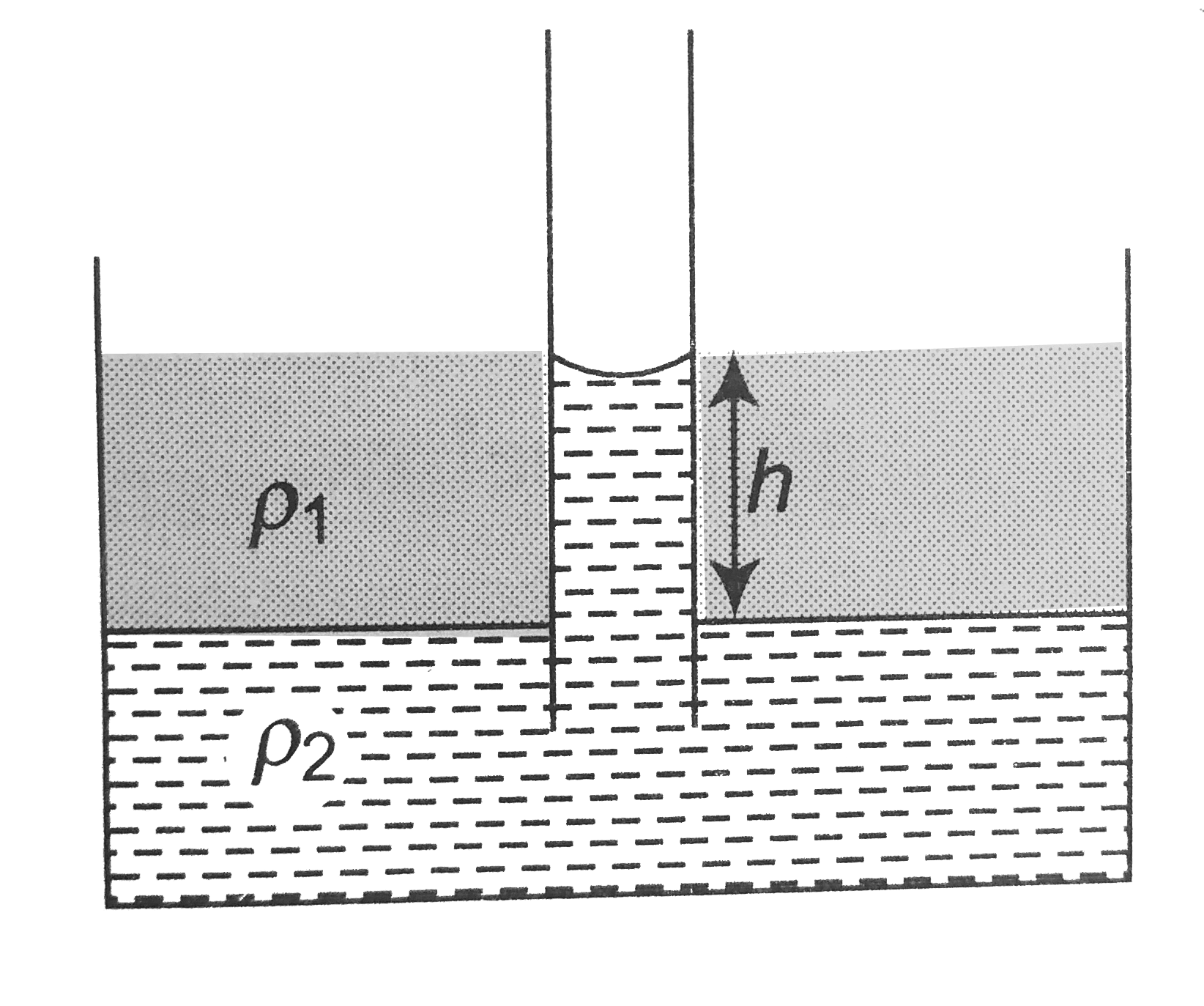
A container is partially filled with a liquid of density ρ2 A capillary tube of radius r is vertically inserted in this liquid. Now another liquid of density ρ1(ρ1<ρ2) is slowly poured in the container to a height h as shown. There is only denser liquid in the capillary tube. The rise of denser liquid in the capillary tube is also h. Assuming zero contact angle, the surface tension of heavier liquid isView SolutionThe correct curve between the height or depression h of liquid in a capillary tube and its radius is
View SolutionA liquid of density ρ and surface tension σ rises in a capillary tube of diameter d. Angle of contact between the tube and liquid is zero. The weight of the liquid in the capillary tube is:
View SolutionThe angle of contact of a liquid in a capillary tube is 60∘. The shape of the meniscus is
View SolutionWater rises in a glass capillary tube, but descends if the bore of the capillary tube is coated with paraffin wax. Why ?
View SolutionThe rate of flow of liquid through a capillary tube in an experiment to determine the viscosity of the liquid increases
View SolutionA thread of liquid is in a uniform capillary tube of length L. As measured by a ruler. The temperature of the tube and thread of liquid is raised by ΔT. If γ be the coefficient of volume expansion of the liquid and α be the coefficient of linear expansion of the material of the tube, then the increase ΔL in the length of the thread, again measured by the ruler will be
View SolutionWater rises up to a height h in a capillary tube of certain diameter. This capillary tube is replaced by a similar tube of half the diameter. Now the water will rise to the height of
View Solution