Question

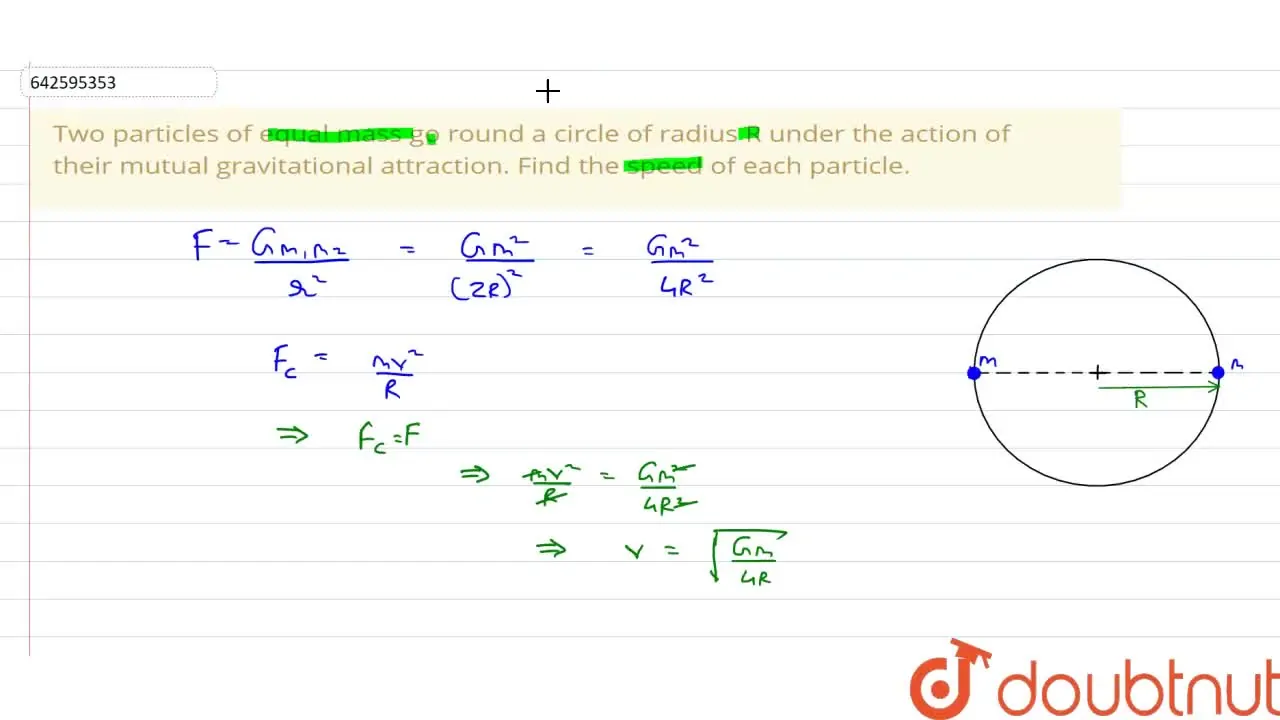
Two particles of equal mass go round a circle of radius R under the action of their mutual gravitational attraction. Find the speed of each particle.

Explanation

To find the speed of each particle in a system where two particles of equal mass are moving in a circle of radius $$R$$ under the influence of their mutual gravitational attraction, we can follow these steps:

1. Understanding the System:
We have two particles, each with mass \( m \), revolving around a common center of mass in a circular path. The distance between the two particles is \( 2R \) since each particle is at a distance \( R \) from the center of the circle.

2. Gravitational Force:
The gravitational force \( F \) between the two particles can be expressed using Newton's law of gravitation:
\( F = \frac{G m^2}{(2R)^2} \)
where \( G \) is the gravitational constant.

3. Simplifying the Gravitational Force:
Simplifying the expression for gravitational force:
\( F = \frac{G m^2}{4R^2} \)

4. Centripetal Force Requirement:
For circular motion, the centripetal force required to keep each particle in circular motion is given by:
\( F_c = \frac{m v^2}{R} \)
where \( v \) is the speed of each particle.

5. Equating Forces:
Since the gravitational force provides the necessary centripetal force, we can set these two forces equal:
\( \frac{m v^2}{R} = \frac{G m^2}{4R^2} \)

6. Cancelling Common Terms:
We can cancel \( m \) from both sides (assuming \( m \neq 0 \)):
\( \frac{v^2}{R} = \frac{G m}{4R^2} \)

7. Rearranging for Speed:
Multiplying both sides by \( R \):
\( v^2 = \frac{G m}{4R} \)

8. Taking the Square Root:
Finally, taking the square root of both sides to find \( v \):
\( v = \sqrt{\frac{G m}{4R}} \)

Final Answer:
The speed of each particle is:
\( v = \sqrt{\frac{G m}{4R}} \)

---