What minimum horizontal speed should be given to the bob of a simple pendulum of length l so tht it describes a complete circle?
To find the minimum horizontal speed that should be given to the bob of a simple pendulum of length l so that it describes a complete circle, we can follow these steps:
Step 1: Understand the Problem
We need to determine the minimum speed u at the lowest point of the pendulum such that the bob can complete a vertical circular motion. The critical point occurs when the bob is at the top of the circle.
Step 2: Analyze Forces at the Top of the Circle
At the top of the circular path, the forces acting on the bob are:
- The gravitational force mg acting downwards.
- The tension T in the string acting downwards.
For the bob to just complete the circle, the tension T can be zero at the topmost point. Therefore, the centripetal force required to keep the bob in circular motion is provided entirely by the weight of the bob:
mg=mv2r
where v is the speed of the bob at the top of the circle and r is the radius of the circle (which is equal to the length l of the pendulum).
Step 3: Solve for Speed at the Top
From the equation above, we can simplify:
g=v2l
Thus, the speed v at the top of the circle is:
v=√gl
Step 4: Apply Conservation of Energy
Using the conservation of mechanical energy, the total mechanical energy at the lowest point (initial) must equal the total mechanical energy at the highest point (final).
At the lowest point:
- Kinetic Energy (KE) = 12mu2
- Potential Energy (PE) = 0 (taking the lowest point as the reference)
At the highest point:
- Kinetic Energy (KE) = 12mv2
- Potential Energy (PE) = mg(2l) (since the height is 2l)
Setting the initial energy equal to the final energy:
12mu2=12mv2+mg(2l)
Step 5: Substitute for v
We know from Step 3 that v=√gl. Substituting this into the energy equation gives:
12mu2=12m(√gl)2+mg(2l)
12mu2=12mgl+mg(2l)
12mu2=12mgl+2mgl
12mu2=52mgl
Step 6: Solve for u
Cancel m from both sides (assuming m≠0):
12u2=52gl
Multiplying both sides by 2:
u2=5gl
Taking the square root:
u=√5gl
Final Answer
The minimum horizontal speed u that should be given to the bob of a simple pendulum of length l to describe a complete circle is:
u=√5gl
---
We need to determine the minimum speed u at the lowest point of the pendulum such that the bob can complete a vertical circular motion. The critical point occurs when the bob is at the top of the circle.
At the top of the circular path, the forces acting on the bob are:
- The gravitational force mg acting downwards.
- The tension T in the string acting downwards.
mg=mv2r
where v is the speed of the bob at the top of the circle and r is the radius of the circle (which is equal to the length l of the pendulum).
From the equation above, we can simplify:
g=v2l
Thus, the speed v at the top of the circle is:
v=√gl
Using the conservation of mechanical energy, the total mechanical energy at the lowest point (initial) must equal the total mechanical energy at the highest point (final).
- Kinetic Energy (KE) = 12mu2
- Potential Energy (PE) = 0 (taking the lowest point as the reference)
- Kinetic Energy (KE) = 12mv2
- Potential Energy (PE) = mg(2l) (since the height is 2l)
12mu2=12mv2+mg(2l)
We know from Step 3 that v=√gl. Substituting this into the energy equation gives:
12mu2=12m(√gl)2+mg(2l)
12mu2=12mgl+mg(2l)
12mu2=12mgl+2mgl
12mu2=52mgl
Cancel m from both sides (assuming m≠0):
12u2=52gl
Multiplying both sides by 2:
u2=5gl
Taking the square root:
u=√5gl
The minimum horizontal speed u that should be given to the bob of a simple pendulum of length l to describe a complete circle is:
u=√5gl
Topper's Solved these Questions
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Objective -1
10 Videos
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Objective -2
8 Videos
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer
16 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer
7 Videos
Similar Questions
A ball of mass m moving horizontally at a speed v collides with the bob of a simple pendulum at rest. The mass of the bob is also m. If the collision is perfectly elastic and both balls sticks, the height to which the two balls rise after the collision will be given by:
View SolutionA particle is attached with a string of length l which is fixed at point O on an inclined plane what minimum velocity should be given to the particle along the incline so that it may complete a circle on inclined plane ( plane is smooth and initially particle was resting on the inclined plane . )
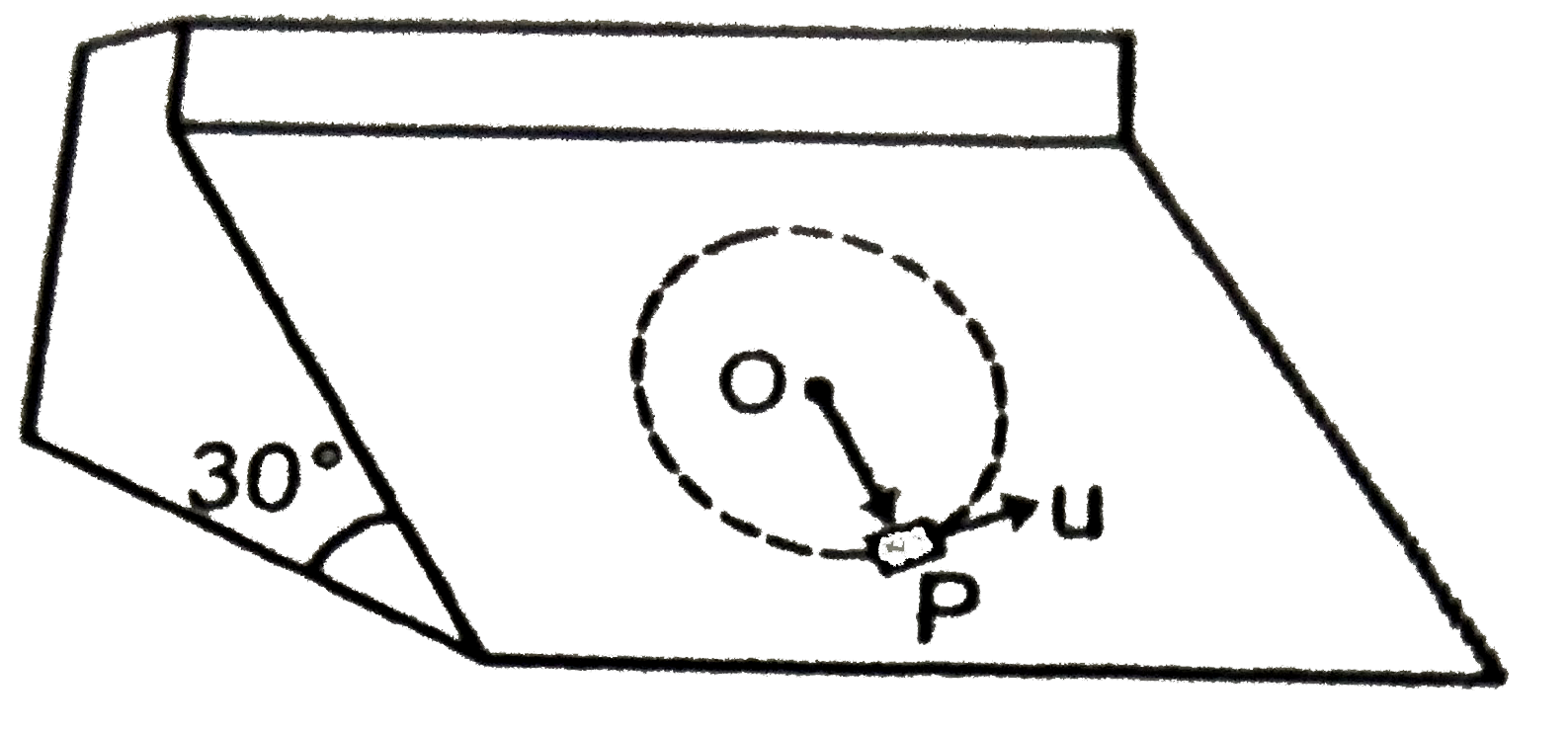
View Solution
Knowledge Check
What is the length of a simple pendulum which ticks second?
A0.5 mB1 mC1.5 mD2 mWhat is the effect on the time period of a simple pendulum if the mass off the bob is doubled?
AHalvedBDoubleCBecomes 8 timesDNo effects
A mass is attached to the end of a string of length l which is tied to a fixed point O. The mass is released from the initial horizontal position of the string. Below the point O at what minimum distance a peg P should be fixed so that the mass turns about P and can describe a complete circle in the vertical plane?
View SolutionA ball of mass m is attached to one end of a light rod of length l, the other end of which is hinged. What minimum velocity v should be imparted to the ball downwards, so that it can complete the circle ?

View SolutionWhat is the torque acting on the bob of mass m of a simple pendulum Of length l, when the string of the pendulum makes an angle θ with the vertical ? When is the torque (i) zero and (ii) maximum.
View SolutionWhat is the length of a simple pendulum which ticks second?
View SolutionWhat is simple peridulum ? Define length of the pendulum.
View SolutionIf the inertial mass m1 of the bob of a simple pendulum of length 'l' is not equal to the gravitationalmassmg, then its period is
View Solution
