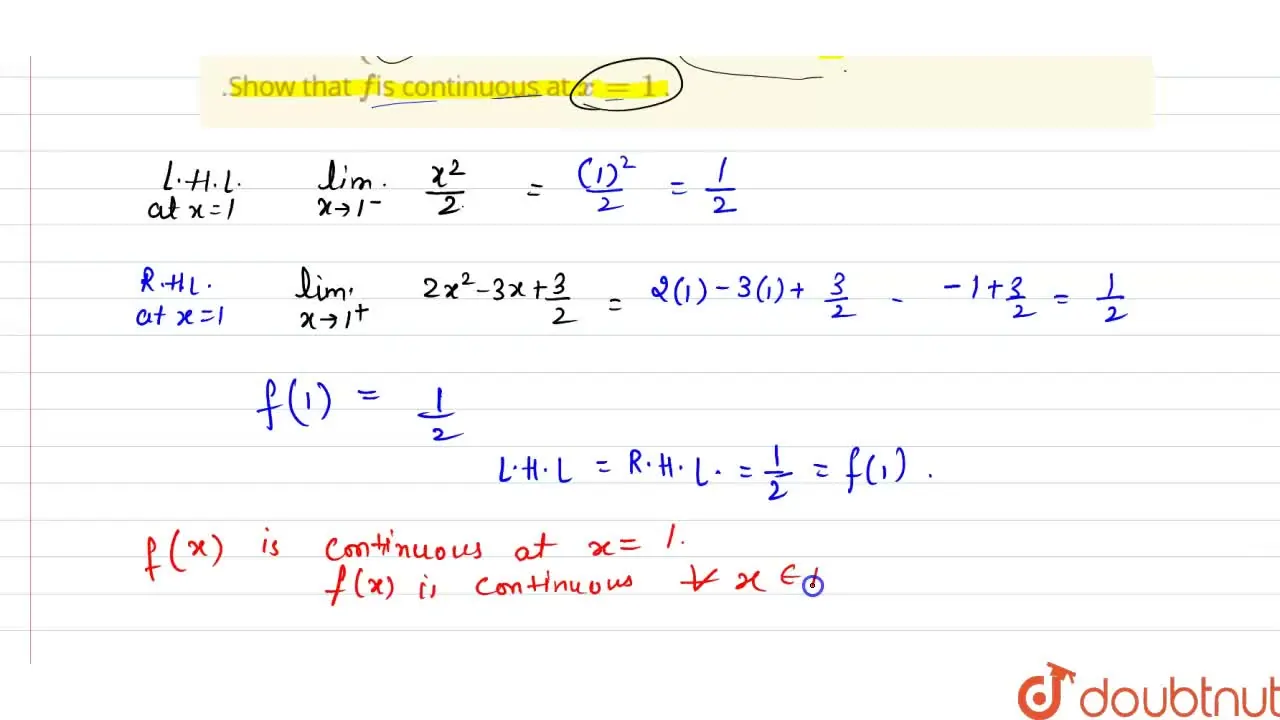
If .Show that is continuous at .
Step by step video & image solution for If f(x)={(x^2)/2, if 0<=x<1, 2x^2-3x+3/2, if1 <=x<=2 .Show that fis continuous at x=1 . by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
Discuss continuity of f(x)=⎧⎨⎩x22if0≤x≤12x2−3x+32if1<x≤2 at x=1
View SolutionCheck the continuity of f(x) = ⎧⎨⎩x22if0≤x≤12x2−3x+32if1<x≤2 at x=1
View SolutionFor x>3,f(x)=3x−2 and for −2≤x≤2,f(x)=x2−2, find f(0)+f(4)
View SolutionThe value of ′c′ in Lagrange's mean value theorem for f(x)=x(x−2)2 in [0,2] is-
View SolutionIf f(x)={x2,x≤02sinx,x>0'∈vestigatethe function at x=0 for maxima/minima
View SolutionDraw the graph of f(x)=[x2],x∈[0,2), where [⋅] denotes the greatest integer function.
View SolutionDetermine the value of the constant m so that the function f(x)={m(x2−2x),ifx<0cosx,ifx≥0 is continuous.
View SolutionFind the value of the constant k so that the given function is continuous at the indicated point: f(x)={k(x2+2), if x≤03x+1, if x>0 at x=0 .
View SolutionFind the value of the constant k so that the given function is continuous at the indicated point: f(x)={k(x2−2x), if x<0cosx, if x≥0 at x=0
View SolutionIf f(x) ={2x2+2x2,0<|x|≤23,x>2then
View Solution