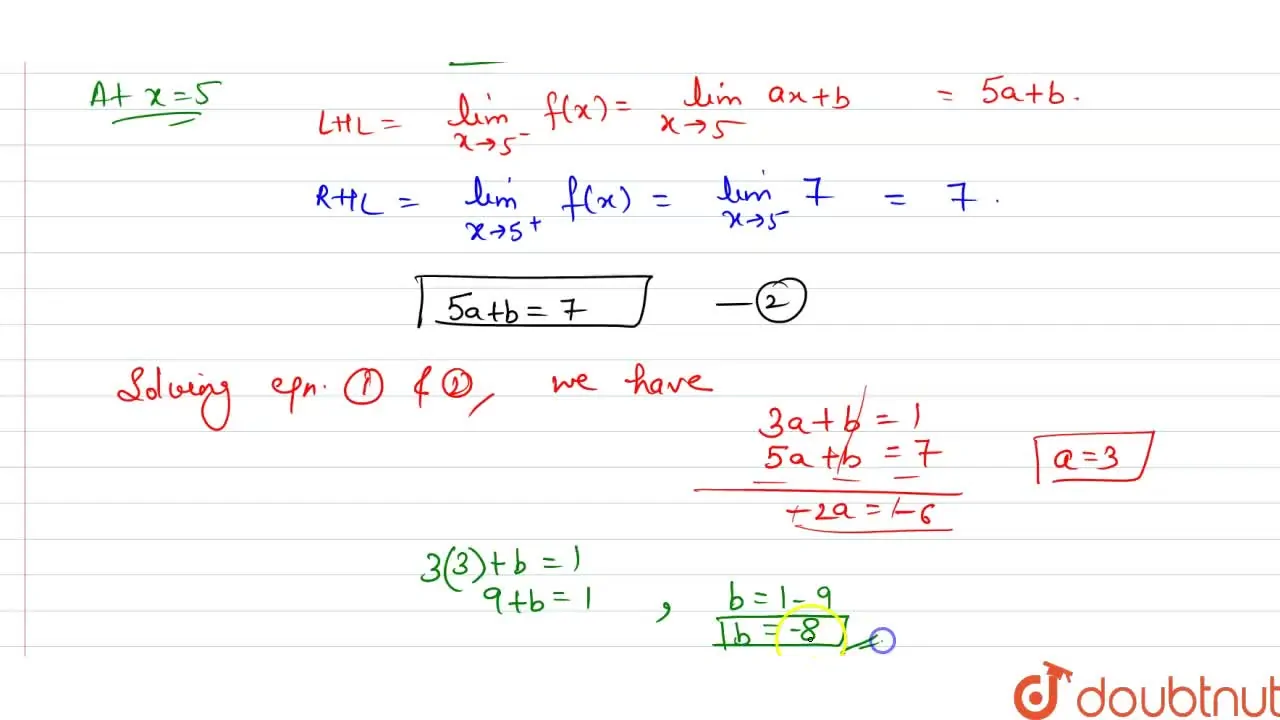
Find the values of a and b so that the function f given by f(x)={1, if x<=3, ax+b , if 3 < x < 5, 7 if x >= 5 is continuous at x=3 and x=5 .
Step by step video & image solution for Find the values of a and b so that the function f given by f(x)={1, if x<=3, ax+b , if 3 < x < 5, 7 if x >= 5 is continuous at x=3 and x=5 . by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
Find the values of a and b so that the function f given by f(x)={ax+b, if 3 < x<5, 1 if x<=3, 7 if x > = 5 is continuous at x=3andx=5.
View SolutionFind the value of 'a' if the function f(x) defined by f(x)={2x−1, x<2 and a, x=2x+1, x>2 is continuous at x=2
View SolutionProve that the function f(x)=5x−3is continuous at x=0, at x=−3and at x=5.
View SolutionFind the relationship between a and b so that the function 'f' defined by f(x)={ax+1,ifx≤3bx+3,ifx<3 is continuous at x=3.
View SolutionFind the value of k, so that the function f(x)={kx2+5ifx≤12ifx>1 is continuous at x=1
View SolutionFind the value of k, so that the function f(x)={kx2+5ifx≤12ifx>1 is continuous at x=1
View SolutionFind the value of a if the function f(x) defined by f(x)={2x−a,,x≤2,x+1,x>2 if function continuous at x=2
View SolutionFind the relationship between a and b so that the function f defined byf(x)={ax+1ifx≤3bx+3ifx>3 is continuous at x=3.
View SolutionFind the value of k for which the function f(x)={3x−8ifx≤52kifx>5 is continuous at x=5
View SolutionFind the value of the constant λ so that the function given below is continuous at x=−1 f(x)={x2−2x−3x+1, x≠−1 λ, x=−1
View Solution