Show that the sum of the three altitudes of a triangle is less than the sum of three sides of the triangle. GIVEN :triangle A B C in which AD⊥BC,BE⊥AC and CF⊥AB. PROVE : AD+BE+CF<AB+BC+AC
Step by step video & image solution for Show that the sum of the three altitudes of a triangle is less than the sum of three sides of the triangle. GIVEN :triangle A B C in which A D_|_B C ,B E_|_A C and C F_|_A Bdot PROVE : A D+B E+C F < A B+B C+A C by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Related Playlists
Similar Questions
Prove that the perimeter of a triangle is greater than the sum of the three medians. GIVEN : A ABC in which AD,BE and CF are its medians. TO PROVE : AB+BC+AC>AD+BE+CF
View SolutionTwo triangles are congruent if two sides and the included angle of one are equal to the corresponding sides and the included angle of the other triangle. GIVEN : Two triangles ABC and DEF such that AB=DE,AC=DF and ∠A=∠D PROVE : ABC≅DEF Figure
View SolutionProve that any two sides of a triangle are together greater than twice the median drawn to the third side. GIVEN : △ABC in which AD is a median. PROVE : AB+AC>2AD CONSTRUCTION : Produce AD to E such that AD=DE. Join EC.
View SolutionTwo triangles are congruent if include two angles and the included side of one triangle are equal to the corresponding two angles and the included side of the other triangle. GIVE : Two △ABC and DEF such that ∠B=∠E,∠C=∠F and BC=EF TO PROVE : △ABC≅△DEF
View SolutionIn Figure, AB⊥BC,FG⊥BC and DE⊥AC.Provetˆ△ A D E~G C F
View SolutionThe sum of any two sides of a triangle is greater than the third side. GIVE : A triangle ABC TO PROVE : AB+AC>BC,AB+BC>AC and BC+AC>AB CONSTRUCTION : Produce side BA to D such that AD=AC. Join CD.
View SolutionIf any two angles and a non-included side of one triangle are equal to the corresponding angles and side of another triangle, then the two triangles are congruent. GIVEN : Two sABC and DEF such that ∠A=∠D,∠B=∠E,BC=EF TO PROVE : ABC≅DEF Figure
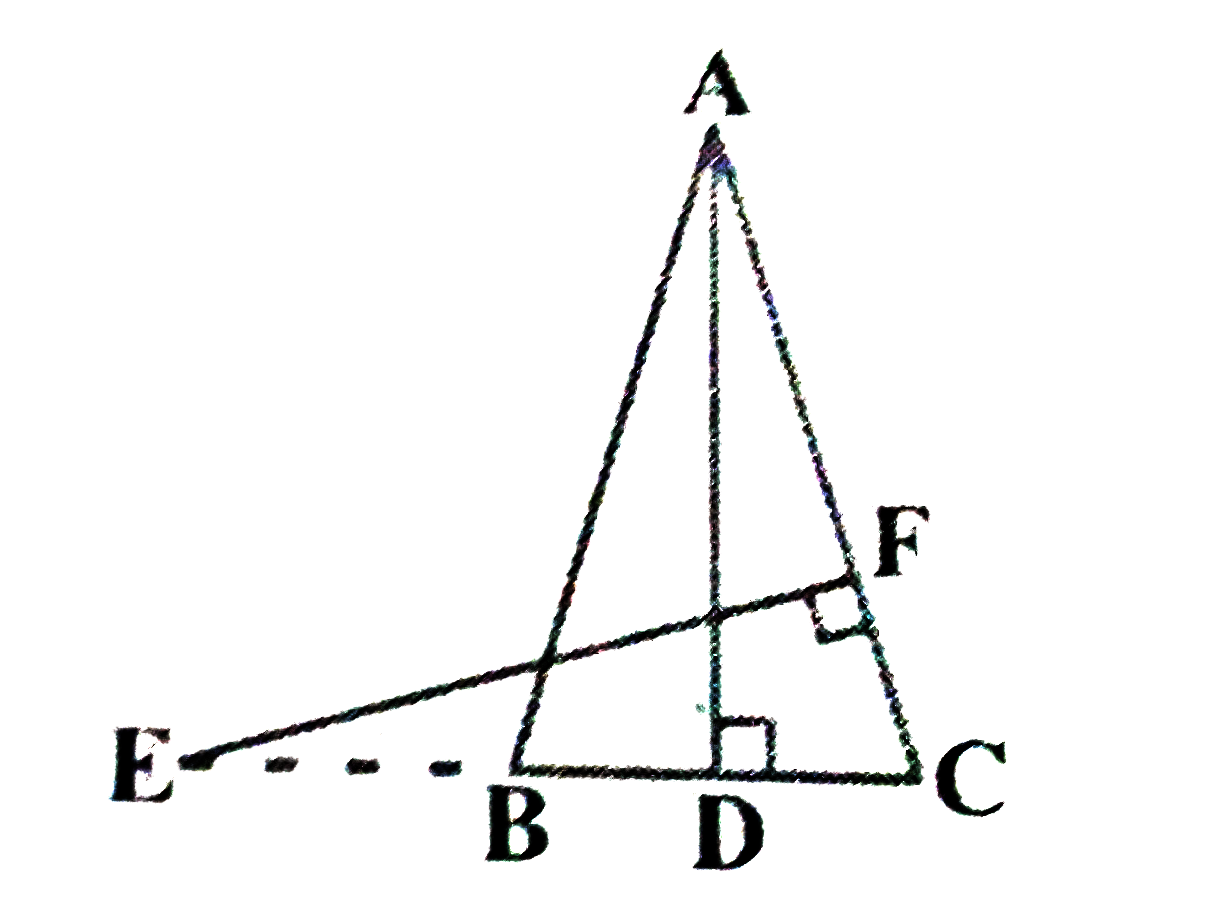
View SolutionIn figureE is a point on side CB produced of an isosceles △ABC with AB=AC. If AD⊥BCandEF⊥AC, prove thatΔABD~ΔECF.
 View Solution
View SolutionAD, BE and CF, the altitudes of ABC are equal. Prove that ABC is an equilateral triangle.
View SolutionAD, BE and CF, the altitudes of ABC are equal. Prove that ABC is an equilateral triangle.
View Solution


