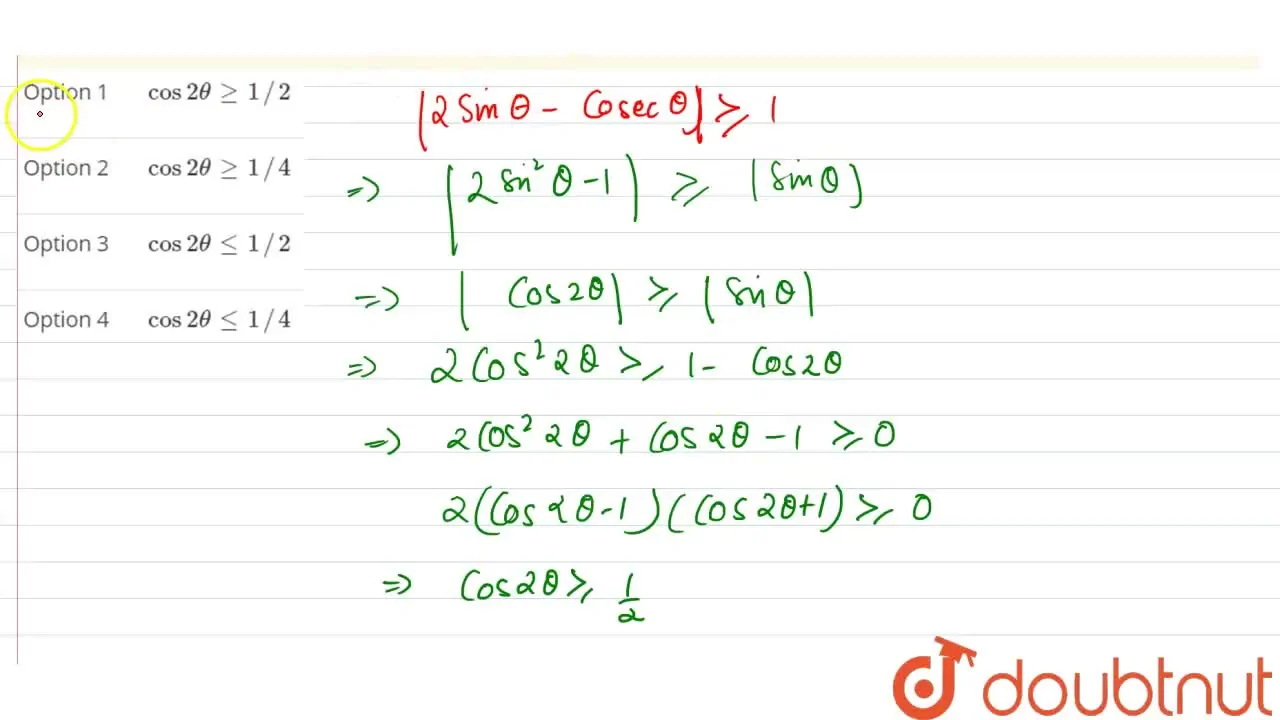
If |2sinθ−cosecθ|≥1 and θ≠nπ2,n∈Z, then
cos2θ≥1/2
cos2θ≥1/4
cos2θ≤1/2
cos2θ≤1/4
The correct Answer is:A
|2sinθ−cosecθ|≥1
or ∣∣2sin2θ−1∣∣≥|sinθ|
or |cos2θ|≥|sinθ|
or 2cos22θ≥1−cos2θ
or 2cos22θ+cos2θ−1≥0
or (2cos2θ−1)(cos2θ+1)≥0
⇒cos2θ≥12 [ ascosθ≠0,i.e.,cos2θ≠−1]
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Exercises (Multiple correct type)31videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Exercises (Linked comprehension type)20videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Concept Application Exercise 4.96videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - ARCHIVES INTEGER TYPE1videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - SINGLE CORRECT ANSWER TYPE38videos
TRIGONOMETRIC FUNCTIONS
Similar Questions
cotθ=sin2θ,θ≠nπ,n∈Z,ifθ equals
View SolutionConsider the equation
secθ+cosecθ=a,θ∈(0,2π)−{π/2,π,3π/2}
If the equation has no real roots, thenView SolutionIf sin 3θ=4sinθ(sin2x−sin2θ),θ≠nπ,n∈Z. Then, the set of values of x, is
View SolutionIf sinθ+cosθ=p and secθ+cosecθ=q; show that q(p2−1)=2p
View SolutionIf sinθ+cosecθ=2, then the value of sin8θ+cosec8θ is equal to
View SolutionIf 4sin2θ=1, then the values of θ are 2nπ±π3, n∈Z b. nπ±π3, n∈Z c. nπ±π6, n∈Z d. 2nπ±π6, n∈Z
View SolutionIf cosθ=−12 and π<θ<3π2 then find the value of 4tan2θ−3cosec2θ
View SolutionStatement I If 2cosθ+sinθ=1(θ≠π2) then the value of 7cosθ+6sinθ is 2.
Statement II If cos2θ−sinθ=12,0<θ<π2, then sinθ+cos6θ=0.View SolutionIf sinθ+cosecθ=2, then find the value of sin20θ+cosec20θ.
View SolutionIf sinθ+cosθ=m and secθ+cosecθ=n then n(m+1)(m−1) equals
View Solution