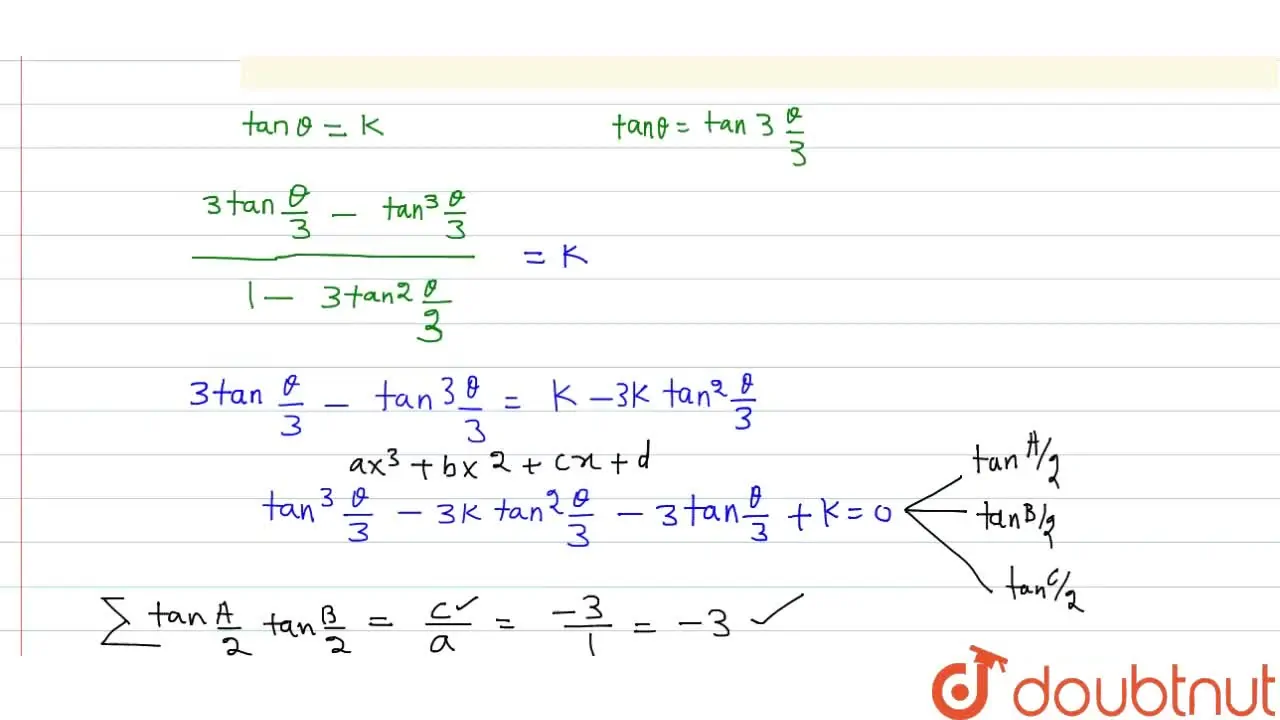
If A,BandC are three values lying in [0,2π] for which tanθ=K then tanA3tanB3+tanB3tanC3+tanC3tanA3 is equal to ___________.
The correct Answer is:-3
tanθ=K
⇒=3tanθ3−tan3θ31−3tan2θ3=K
⇒tan3θ3−3Ktan2θ3−3tanθ3+K=0
Roots of this equation are tanA3,tanB3,tanC3.
∴ Sum of roots taken two at a time = ∑tanA3tanB3=−3.
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Single correct Answer Type (Archives) JEE Main5videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Single correct Answer Type (Archives) JEE Advanced1videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Exercise (Linked Comprehension Type)20videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - SINGLE CORRECT ANSWER TYPE38videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS FOR COMPOUND, MULTIPLE, SUB-MULTIPLE ANGLES, AND TRANSFORMATION FORMULAS EXERCISE - Multiple Correct Answers Type6videos
TRIGONOMETRIC RATIOS FOR COMPOUND, MULTIPLE, SUB-MULTIPLE ANGLES, AND TRANSFORMATION FORMULAS
Similar Questions
tan2π5−tanπ15−√3tan2π5tanπ15 is equal to
View Solutiontan2π5−tanπ15−√3tan2π5tanπ15 is equal to
View SolutionIf tanA=12,tanB=13,thentan(2A+B) is equal to
View SolutionIf A+B+C=π, prove that : tan(A2)tan(B2)+tan(B2)tan(C2)+tan(C2)tan(A2)=1
View Solutiontan3A−tan2A−tanA= is equal to
View SolutionIn ΔABCiftan(A2)tan(B2)+tan(B2)tan(C2)=23 then a+c
View SolutionIf θ1,θ2,θ3 are the three values of θ∈[0,2π] for which tanθ=λ then the value of tan(θ1)3tan(θ2)3+tan(θ2)3tan(θ3)3+tan(θ3)3tan(θ1)3 is equal to
View SolutionIn any ΔABC, prove that : (a−bc)=tan(A2)−tan(B2)tan(A2)+tan(B2)
View SolutionLet θ=tan−1(tan.5π4) and ϕ=tan−1(−tan.2π3)then
View SolutionThe value of tan5A−tan3A−tan2A is equal to
View Solution