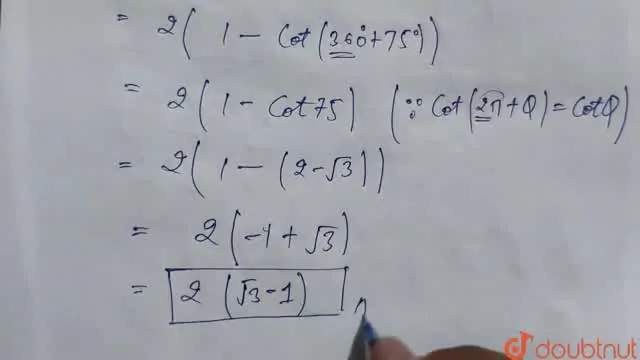The value of is equal to
A
B
C
D
Video Solution
Text Solution
The correct Answer is:C
|
Updated on:21/07/2023
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Archives (Multiple correct Answers Type) (JEE Advanced)2videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Archives (Numerical Value Type)2videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Single correct Answer Type (Archives) JEE Main5videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - SINGLE CORRECT ANSWER TYPE38videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS FOR COMPOUND, MULTIPLE, SUB-MULTIPLE ANGLES, AND TRANSFORMATION FORMULAS EXERCISE - Multiple Correct Answers Type6videos
TRIGONOMETRIC RATIOS FOR COMPOUND, MULTIPLE, SUB-MULTIPLE ANGLES, AND TRANSFORMATION FORMULAS
Similar Questions
Q. The value of is equal 13∑k=1⎛⎜ ⎜⎝1sin(π4+(k−1)π6)sin(π4+kπ6) is equal
View SolutionThe value of
1+14∑k=0{cos((2k+1)π)15−isin((2k+1)π)15}, isView SolutionThe value of
sin6(π49)+cos6(π49)−1+3sin2(π49)cos2(π49) is equal toView Solutionsin−1(cos(5π4)) is equal to
View Solutionsin−1(sin(7π4))
View Solutionsin−1(sin(9π4))
View Solutionsin−1(sin(7π6))
View SolutionThe value of limn→∞n∑r=11sin{(n+r)π4n}.πn is equal to
View Solution"sin^(2)(pi)/(6)+cos^(2)(pi)/(3)-tan^(2)(pi)/(4)=-(1)/(2)"
View SolutionValue of cos−1cos(13π)6 is
View Solution