Question

If the ellipse ${\displaystyle \frac{x^2}{a^2}+\frac{y^2}{b^2}=1}$
is inscribed in a rectangle whose length to breadth ratio is ${\displaystyle 2:1}$
, then the area of the rectangle is
${\displaystyle 4.\frac{a^2+b^2}{7}}$
(b) ${\displaystyle 4.\frac{a^2+b^2}{3}}$

${\displaystyle 12.\frac{a^2+b^2}{5}}$
(d) ${\displaystyle 8.\frac{a^2+b^2}{5}}$

Options

A. `4*(a^(2)+b^(2))/(7)`
B. `4*(a^(2)+b^(2))/(3)`
C. `12*(a^(2)+b^(2))/(5)`
D. `8*(a^(2)+b^(2))/(5)`

Explanation

Since mutually perpendicualar tangents can be drawn from the vartices of the reactangle , all the vertices of the rectangle should lie on the director circle `x^(2)+y^(2)=a^(2)+b^(2)`

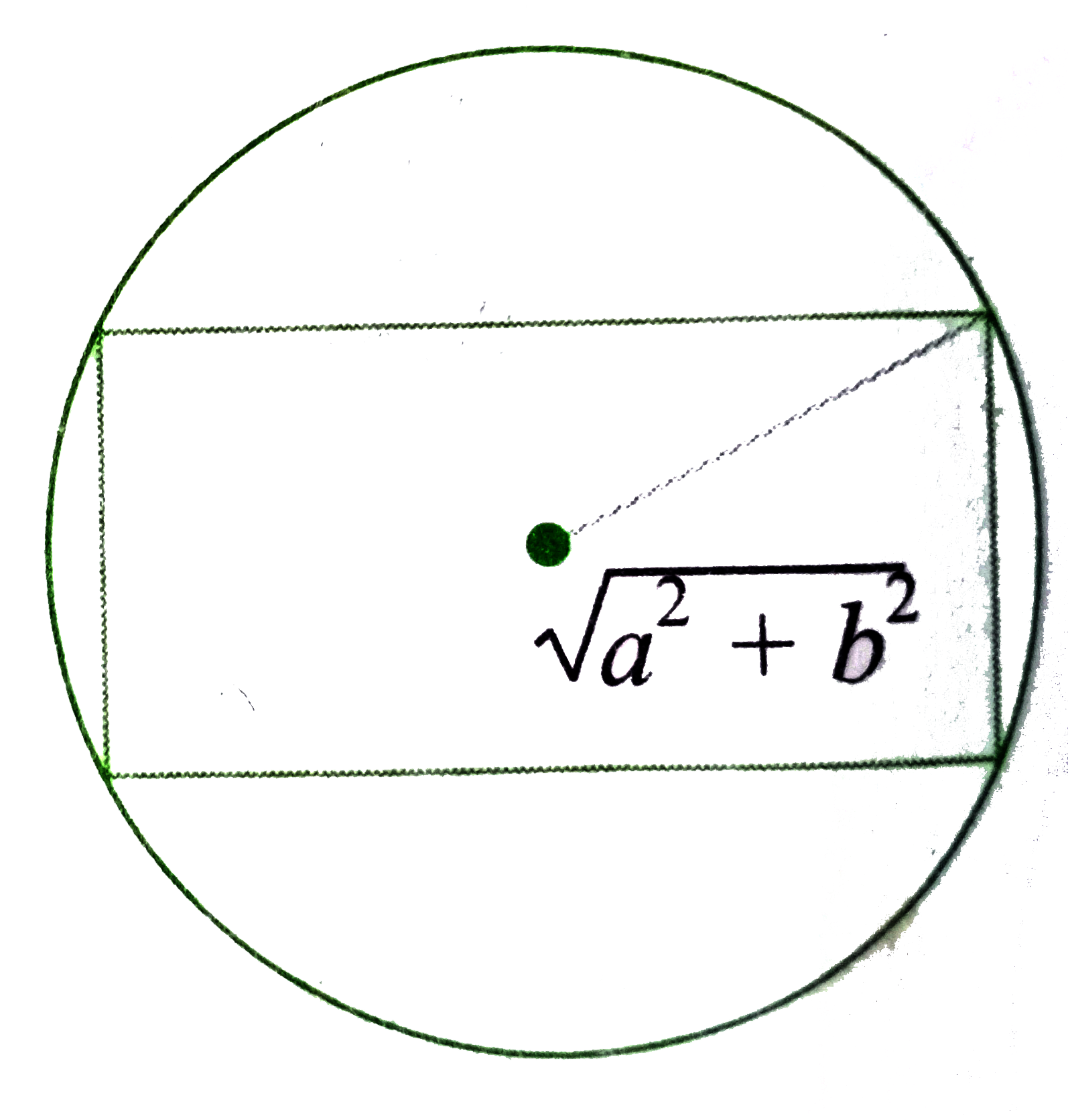
Let breadht =2l and length =4l . then,
`l^(2)+(2l)^(2)=a^(2)+b^(2)`
or `l^(2)=(a^(2)+b^(2))/(5)`
or Area of `4lxx2l=8((a^(2)+b^(2))/(5))`