A circle with center (a,b) passes through the origin. The equation of the tangent to the circle at the origin is (a)ax−by=0 (b) ax+by=0 bx−ay=0 (d) bx+ay=0
ax−by=0
ax+by=0
bx−ay=0
bx+ay=0
The correct Answer is:2
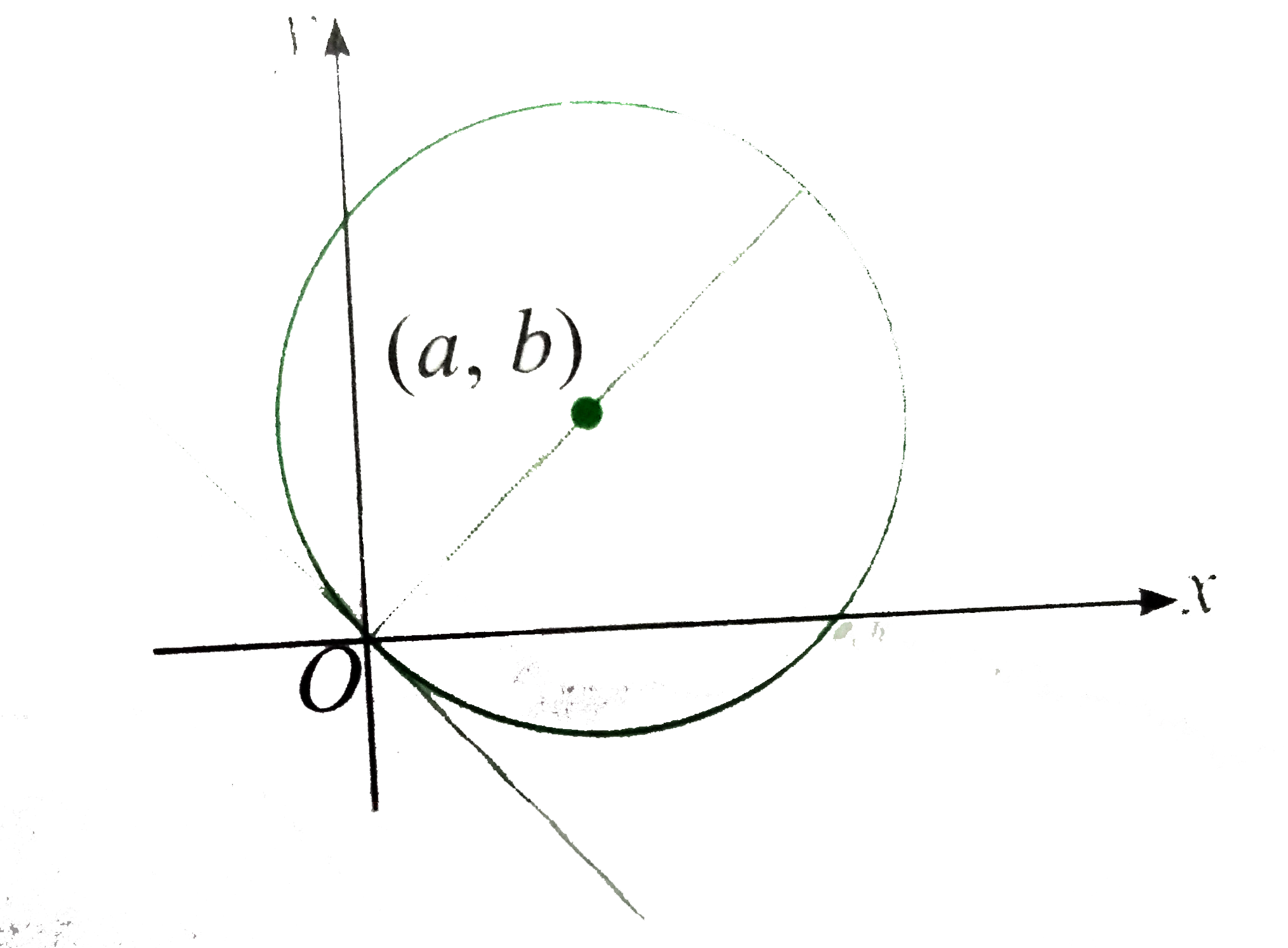
Obviously , the slope of the tangent will be (1b/a),i.e.,−ab.
Hence, the equation of the tangent is y=−abx,i.e., by +ax=0
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - Multiple Correct Anser Type
29 Videos
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - Linked Comprehension Type (For Problem 1-3)
3 Videos
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.20
1 Videos
- BINOMIAL THEOREMBOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix
4 Videos
- CIRCLESBOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
Similar Questions
Knowledge Check
The equation of the diameter of the circle x2+y2−6x+2y=0 which passes through origin is
Ay+3x=0Bx - 3y = 0Cx + 3y = 0Dy - 3x = 0
If x+3y=0 is a tangent to the circle with centre at (-1,2) then show that the other tangent to the circle from the origin is 3x−y=0
View SolutionThe equation to the normal to the curve y=sinx at (0, 0) is
(a)x=0
(b) y=0
(c) x+y=0
(d) x−y=0
View SolutionIf 3x+y=0 is a tangent to a circle whose center is (2,−1) , then find the equation of the other tangent to the circle from the origin.
View SolutionThe equation of common tangent(s) to the parabola y2=16x and the circle x2+y2=8 is/are x+y+4=0 (b) x+y−4=0 x−y−4=0 (d) x−y+4=0
View SolutionEquation of the diameter of the circle x2+y2−2x+4y=0 which passes through the origin is a.x+2y=0 b.x-2y=0 c. 2x+y=0d.2x-y=0.
View SolutionFind the equation of the straight line which passes through
the origin and the point of intersection of the lines ax+by+c=0anda'x+b'y+c'=0.
View SolutionA parabola y=ax2+bx+c crosses the x-axis at (α,0)(β,0) both to the right of the origin. A circle also passes through these two points. The length of a tangent from the origin to the circle is: (a) √bca (b) ac2 (c) b/a (d) √ca
View Solution
