Two equal circles of largest radii have following property:
(i) They intersect each other orthogonally,
(ii) They touch both the curves 4(y+2)=x2 and 4(2−y)=x2 in the region x∈[−2√2,2√2]. Then radius of this circle is
√2
√3
1√3
32
The correct Answer is:A
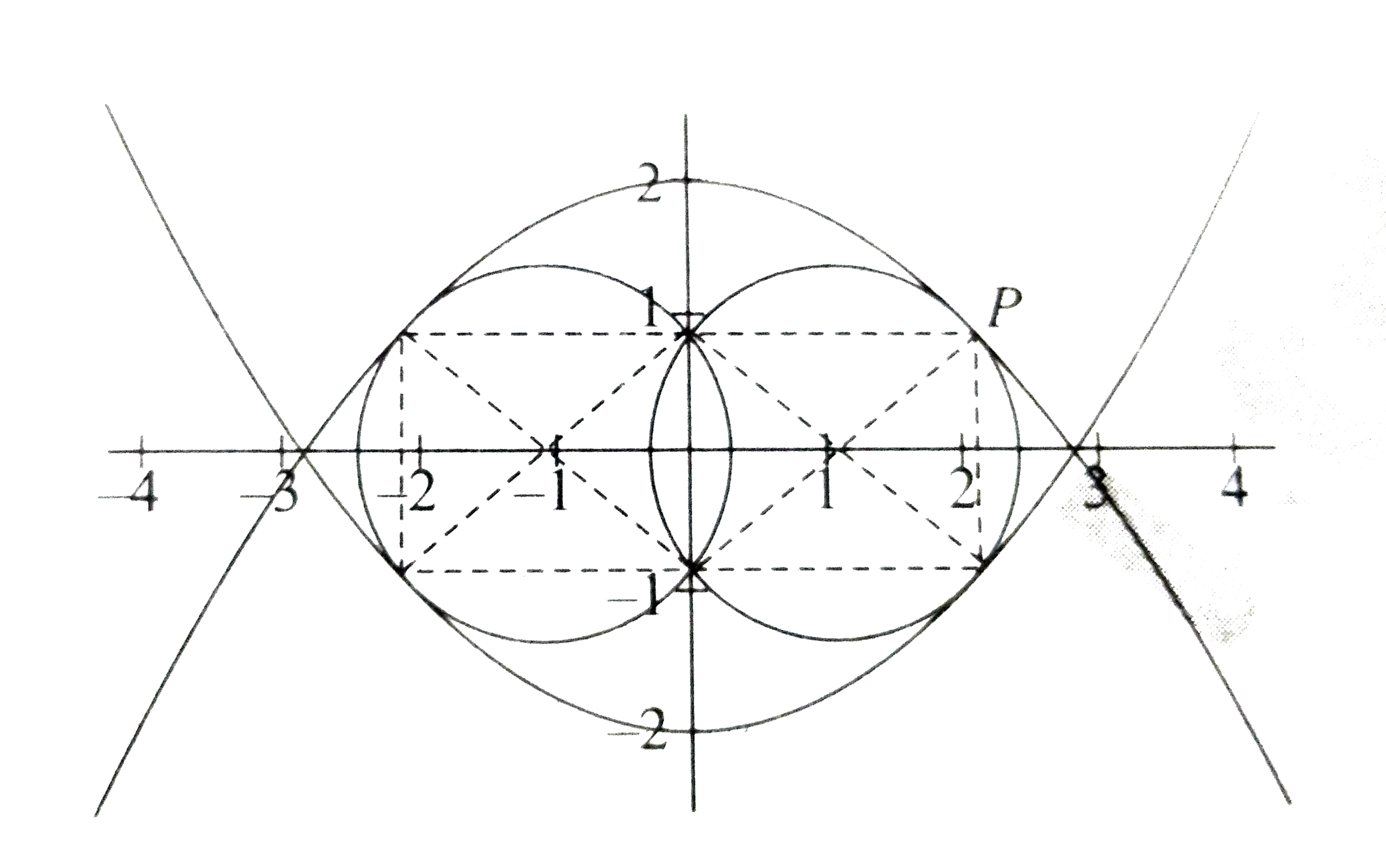
Let the radius of circle is r.
From geometry, P≡(r√2,r√2).
Now, this point lies on the curve 4(2−y)=x2
⇒4(2−r√2)=(r√2)2
⇒r=√2
Topper's Solved these Questions
- PARABOLABook:CENGAGE ENGLISHChapter:PARABOLAExercise:Multiple Correct Answers Type
Explore 10 Videos
- PARABOLABook:CENGAGE ENGLISHChapter:PARABOLAExercise:Comprehension Type
Explore 2 Videos
- PARABOLABook:CENGAGE ENGLISHChapter:PARABOLAExercise:MULTIPLE CORRECT ANSWER TYPE
Explore 7 Videos
- PAIR OF STRAIGHT LINESBook:CENGAGE ENGLISHChapter:PAIR OF STRAIGHT LINESExercise:Numberical Value Type
Explore 5 Videos
- PERMUTATION AND COMBINATIONBook:CENGAGE ENGLISHChapter:PERMUTATION AND COMBINATIONExercise:Comprehension
Explore 8 Videos
Similar Questions
Show that the circles given by the following equations intersect each other orthogonally .
x2+y2+2x−2y=0
x2+y2+2x+2y=0
View SolutionShow that the circles given by the following equation intersect each other orthogonally.
x2+y2−2x+4y+4=0,
x2+y2+3x+4y+1=0.
View SolutionShow that the circles given by the following equations intersect each other orthogonally .
x2+y2+4x−2y−11=0
x2+y2−4x−8y+11=0
View SolutionTwo circles of radii r1andr2, are both touching the coordinate axes and intersecting each other orthogonally. The value of r1r2 (where r1>r2) equals -
View SolutionShow that x2+4y2=8 and x2−2y2=4 intersect orthogonally
View SolutionThe two circles x2+y2−cx=0andx2+y2=4 touch each other if:
View SolutionThe angle of intersection of the circles x2+y2=4 and x2+y2+2x+2y, is
View SolutionProve that the curves xy=4 and x2+y2=8 touch each other.
View SolutionThe are bounded by the curve y2=4x and the circle x2+y2−2x−3=0 is
View SolutionFind the area bounded by the curves x2+y2=4,x2=−√2y and x=y
View Solution