Solve (x−1)(x−2)(1−2x)>0.
Video Solution
Text Solution
We have (x−1)(x−2)(1−2x)>0
or−(x−1)(x−2)(2x−1)>0
or (x−1)(x−2)(2x−1)<0
On the number line, mark x=1/2,1,2
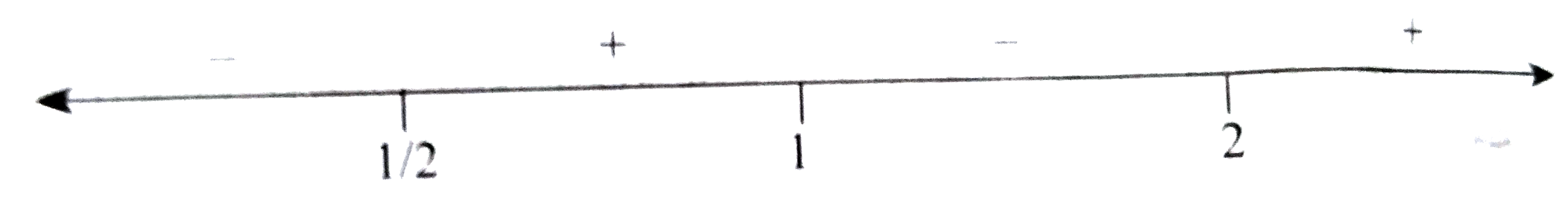
When x>2, all factors (x−1),(2x−1), and (x−2) are passive.
Hence, (x−1)(x−2)(2x−1)>0 for x>2.
Now put positive and negative signs alternatively as shown in the figure.
Hence, solution set of (x−1)(x−2)(1−2x)>0 or (x−1)(x−2)(2x−1)<0 is (−∞,1/2)∪(1,2).
|
Topper's Solved these Questions
- RELATIONS AND FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Solved Examples
15 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - CONCEPT APPLICATION EXERCISE 1.1
15 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (Numerical Value Type)
3 Videos
- SCALER TRIPLE PRODUCTS BOOK - CENGAGE ENGLISHCHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.3
11 Videos
Similar Questions
Solve (x−1)(x−2)(1−2x)>0.
View SolutionSolve : x−1x−2>1
View SolutionSolve 1x−2+1x−1>1x.
View SolutionSolve (2x+1)(x−3)(x+7)<0
View SolutionSolve log2.x−1x−2>0 .
View SolutionSolve (x−1)2(x+4)<0
View SolutionSolve 4x−3.2x+2>0.
View SolutionSolve: 5x−1x+2<0.
View SolutionSolve (2x+1)(x−3)(x+7)<0.
View SolutionSolve |x−1|x+2>1
View Solution
