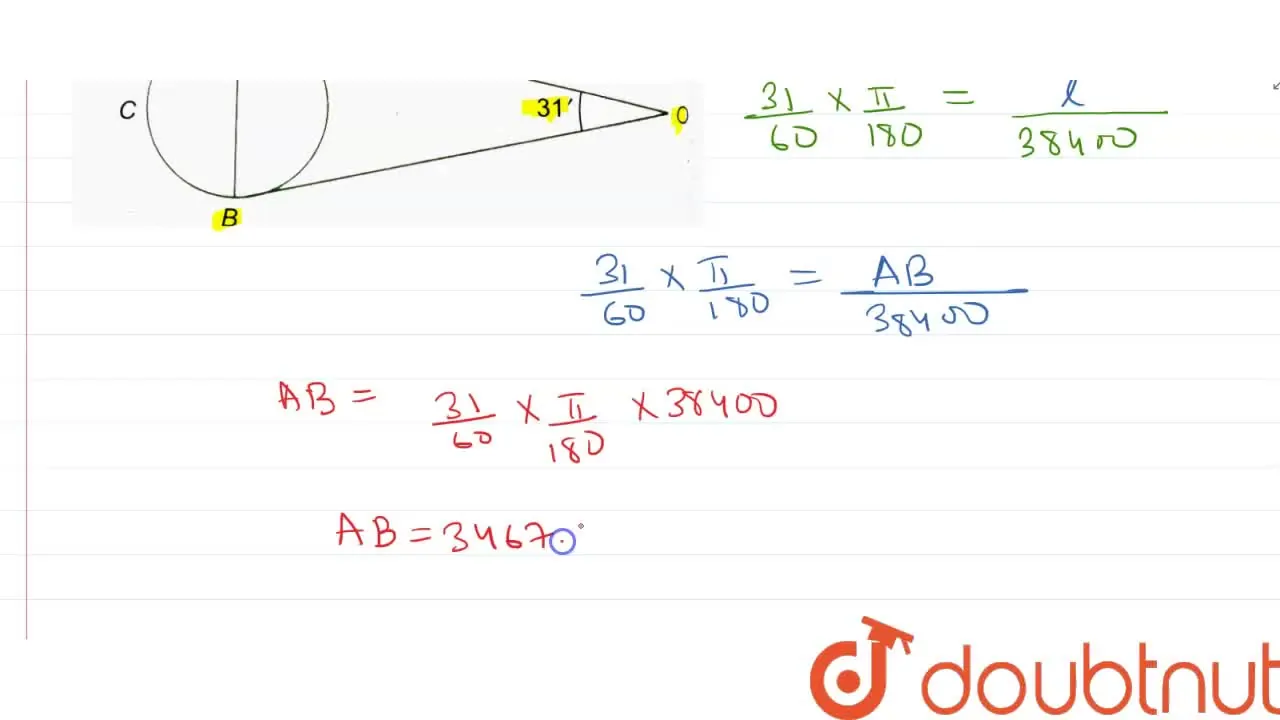
Assuming the distance of the earth from the moon to be 38,400 km and the angle subtended by the moon at the eye of a person on the earth to be 31, find the diameter of the moon.
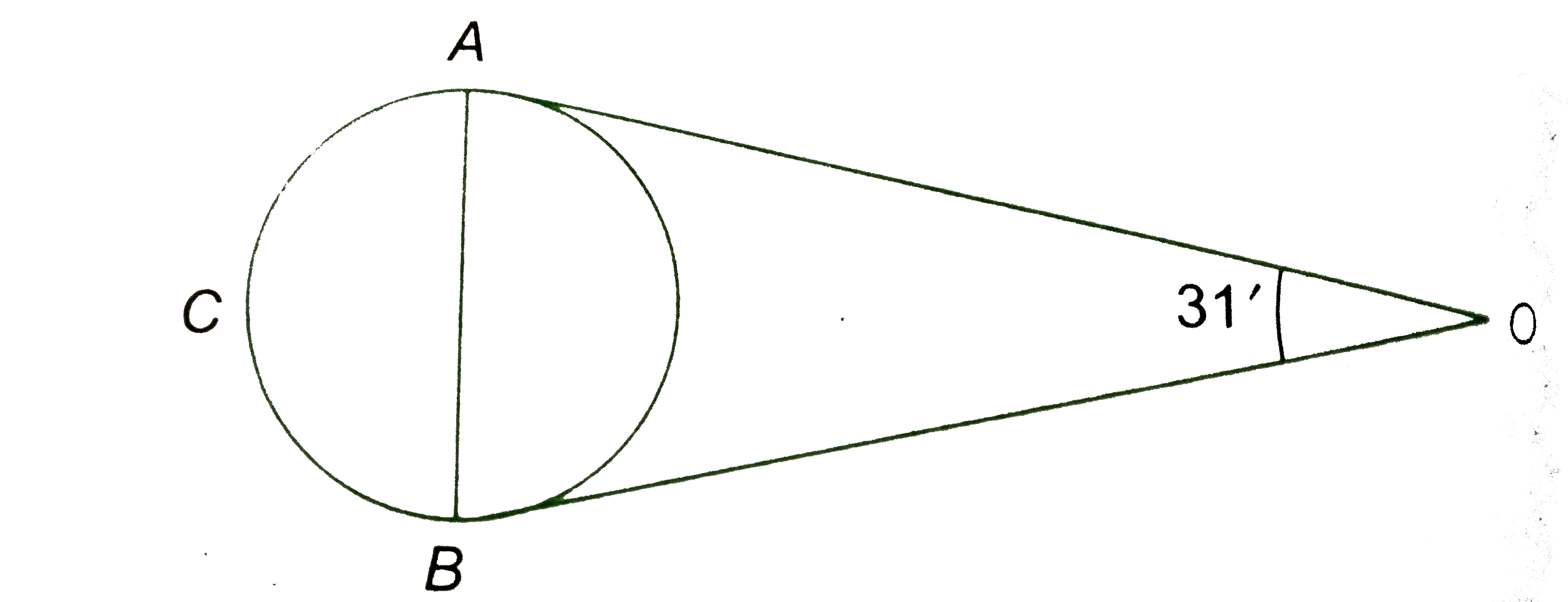
Let AB be the diameter of the moon and O be the observer.
Given, ∠AOB=31'=3160×π180rad
Since the angle subtendedby the moon is very small, itsodiameterwill be approximatelyequal to the small arc of a circle Whose centeris the eye of the observerandthe radius is the distanceof the earthfrom the moon.Also the moon subtendsan angle of 31' at the centerof this circle. Thus,
θ=lr
∴3160×π180=AB384400
or AB=3160×227×180×384400=3467.74km
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - example10videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Concept Application Exercises 2.18videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)1videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type1videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
Similar Questions
Assuming the average distance of the earth from the sun to be 149700000 km and the angle subtended by the sun at the eye of a person on the earth to be 32', find the sun's diameter.
View SolutionThe moon's distance from the earth is 360000 km and its diameter subtends an angle of 31' at the eye of the observer. Find the diameter of the moon.
View SolutionThe moons distance from the earth is 360,000kms and its diameter subtends an angle of 31 at the eye of the observer. Find the diameter of the moon.
View SolutionA big explosion on the moon cannot be heard on the earth because
View SolutionHow is the distance from the moon to the earth determined?
View SolutionIf the moon attracts the earth, why does the earth not move towards the moon?
View SolutionThe moon's distance from the earth is 360000km and its diameter subtends an angle of 42' at the eye of the observer. The diameter of the moon in kilometers is
View SolutionIf a simple pendulum is taken on to the moon from the earth, then it
View SolutionThe mean distance of the moon from the earth is 3.84×105 km and it rotates around the earth in 27.3 days. What is the angular momentum of the moon around the earth ? Mass of the moon = 7.3×1022kg
View SolutionThe moon is observed from two diametrically opposite points A and B on earth. The angle θ substended at the moon by the two directions of observation is 1∘54'. Given the diameter of earth to be about 1.276×107m, calculate the distance of moon from earth.
View Solution