Let AD be a median of the ΔABC. If AE and AF are medians of the triangle ABD and ADC, respectively, and BD=a/2 AD = m1,AE=m2,AF=m3, then a2/8 is equal to
m22+m23−2m21
m21+m22−2m23
m21+m23−2m22
none of these
The correct Answer is:A
To solve the problem, we will use the Apollonius Theorem, which relates the lengths of the sides of a triangle to the length of the median. Let's break down the steps:
Step 1: Understand the given information
We have triangle ABC with median AD. BD is given as a2, where a is the length of side BC. We denote:
- AD=m1
- AE=m2 (median of triangle ABD)
- AF=m3 (median of triangle ADC)
Step 2: Apply the Apollonius Theorem to triangle ABC
According to the Apollonius Theorem:
AB2+AC2=2AD2+2BD2
Substituting the known values:
AB2+AC2=2m21+2(a2)2
Calculating BD2:
BD2=(a2)2=a24
Thus, we have:
AB2+AC2=2m21+2⋅a24=2m21+a22
This is our Equation (1).
Step 3: Apply the Apollonius Theorem to triangle ABD
For triangle ABD, we can write:
AB2+AD2=2AE2+2BD2
Substituting the known values:
AB2+m21=2m22+2(a2)2
This gives us:
AB2+m21=2m22+a24
This is our Equation (2).
Step 4: Apply the Apollonius Theorem to triangle ACD
For triangle ACD, we have:
AC2+AD2=2AF2+2CD2
Since CD=BD=a2:
AC2+m21=2m23+2(a2)2
This gives us:
AC2+m21=2m23+a24
This is our Equation (3).
Step 5: Combine Equations (2) and (3)
Now we add Equations (2) and (3):
(AB2+m21)+(AC2+m21)=(2m22+a24)+(2m23+a24)
This simplifies to:
AB2+AC2+2m21=2m22+2m23+a22
Now, substituting from Equation (1):
2m21+a22=2m22+2m23+a22
Cancelling a22 from both sides, we get:
2m21=2m22+2m23
Dividing by 2:
m21=m22+m23
Step 6: Final result
From the above, we can express:
a28=m22+m23−2m21
Conclusion
Thus, we conclude that:
a28=m22+m23−2m21
We have triangle ABC with median AD. BD is given as a2, where a is the length of side BC. We denote:
- AD=m1
- AE=m2 (median of triangle ABD)
- AF=m3 (median of triangle ADC)
According to the Apollonius Theorem:
AB2+AC2=2AD2+2BD2
Substituting the known values:
AB2+AC2=2m21+2(a2)2
Calculating BD2:
BD2=(a2)2=a24
Thus, we have:
AB2+AC2=2m21+2⋅a24=2m21+a22
This is our Equation (1).
For triangle ABD, we can write:
AB2+AD2=2AE2+2BD2
Substituting the known values:
AB2+m21=2m22+2(a2)2
This gives us:
AB2+m21=2m22+a24
This is our Equation (2).
For triangle ACD, we have:
AC2+AD2=2AF2+2CD2
Since CD=BD=a2:
AC2+m21=2m23+2(a2)2
This gives us:
AC2+m21=2m23+a24
This is our Equation (3).
Now we add Equations (2) and (3):
(AB2+m21)+(AC2+m21)=(2m22+a24)+(2m23+a24)
This simplifies to:
AB2+AC2+2m21=2m22+2m23+a22
Now, substituting from Equation (1):
2m21+a22=2m22+2m23+a22
Cancelling a22 from both sides, we get:
2m21=2m22+2m23
Dividing by 2:
m21=m22+m23
From the above, we can express:
a28=m22+m23−2m21
Thus, we conclude that:
a28=m22+m23−2m21
Topper's Solved these Questions
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Multiple correct answer type
24 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Linked comprehension type
34 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Concept application exercise 5.11
4 Videos
- PROGRESSION AND SERIESBOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (MATRIX MATCH TYPE )
1 Video
- RELATIONS AND FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Archives(Matrix Match Type)
1 Video
Similar Questions
Let AD be a median of the ABC. If AEandAF are medians of the triangle ABDandADC , respectively, and AD=m1,AE=m2,AF=m3, thena28 is equal to (a)m22+m23−2m21 (b) m21+m22−2m23 (c)m21+m23−2m22 (d) none of these
View SolutionBL and CM are medians of a triangle ABC right angled at A. Prove that 4(BL2+CM2)=5BC2
View SolutionThe median AD of the triangle ABC is bisected at E and BE meets AC at F. Find AF:FC.
View SolutionThe median AD of the triangle ABC is bisected at E and BE meets AC at F. Find AF:FC.
View SolutionAD is a median of triangle ABC. Prove that : AB+AC>2AD
View SolutionAD is a median of the ΔABC.Is it trure AB+BC+CA>2AD? Give reason for your answer
View SolutionAD is a median of triangle ABC. Prove that : AB+BC+AC>2AD
View SolutionIn the following figure, BL = CM.
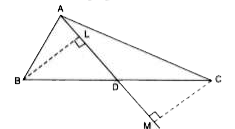
Prove that AD is a median of triangle ABC.
View SolutionIn the given figure, AD = AE and AD2=BD×EC.
Prove that : triangles ABD and CAE are similar.
View SolutionIn triangle ABC,ADandBE are the medians drawn through the angular points A and B respectively. ∠DAB=2∠ABE=36∘ and AD=6 units then circumradius of the triangle is equal to
View Solution
