If the vectors A,B,C of a triangle ABC are (1,2,3),(−1,0,0),(0,1,2), respectively then find ∠ABC.
The vertices of △ABC are given as A(1,2,3) , B(-1,0,0), and C ( 0,1,2)
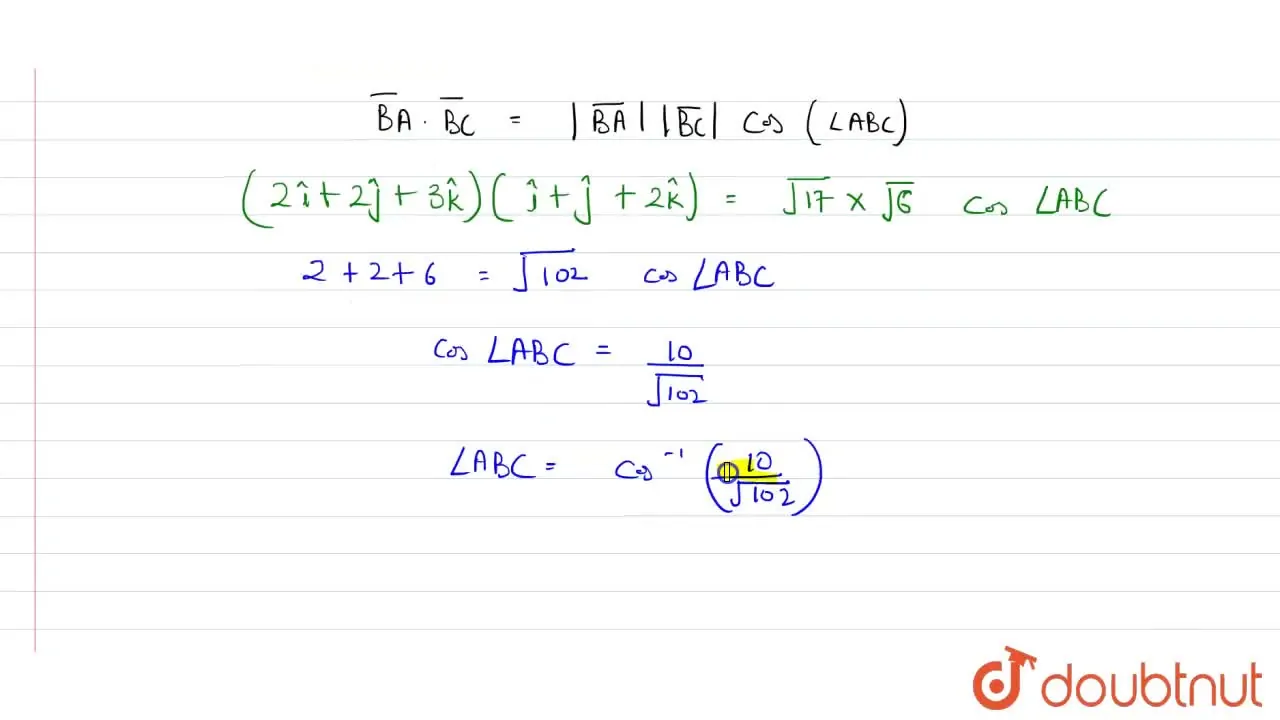
Also, it is given that ∠ABC is the angle between the vectors −−→BAand−−→BC thus
−−→BA={1−(−1)}ˆi+(2−0)ˆj+(3−0)ˆk
2ˆi+2ˆj+3ˆk
−−→BC={0−(−1)}ˆi+(1−0)ˆj+(2−0)ˆk
=ˆi+ˆj+2ˆk
−−→BA.−−→BC=(2ˆi+2ˆj+3ˆk).(ˆi+ˆj+2ˆk)
2×1+2×1+3×2
=2+2+6=10
∣∣∣−−→BA∣∣∣=√22+22+32=√4+4+9=√17
∣∣∣−−→BA∣∣∣=√1+1+22=√6
Now, it is known that :
−−→BA.−−→BC=∣∣∣−−→BA∣∣∣∣∣∣−−→BC∣∣∣cos(∠ABC)
10=√17×√6cos(∠ABC)
orcos(∠ABC)=10√17×√6
or∠ABC=cos−1(10√102)
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Exercise 2.215videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Exercise 2.318videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - DETERMINANTSEXERCISE - All Questions264videos
DETERMINANTS
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Matrix Match Type5videos
DIFFERENTIAL EQUATIONS
Similar Questions
If the vertices A,B,C of a triangle ABC are (1,2,3),(-1,0,0) ,(0,1,2) , respectively, then find ∠ABC.
View SolutionIf the vertices A, B, C of a triangle ABC are (1,2,3),(1,0,0),(0,1,2), respectively, then find ∠ABC. [∠ABCis the angle between the vectors −−→BAand −−→BC.
View SolutionThe equations of the perpendicular bisectors of the sides ABandAC of triangle ABC are x−y+5=0 and x+2y=0 , respectively. If the point A is (1,−2) , then find the equation of the line BC.
View SolutionThe equations of the perpendicular bisectors of the sides ABandAC of triangle ABC are x−y+5=0 and x+2y=0 , respectively. If the point A is (1,−2) , then find the equation of the line BC.
View SolutionABCD is parallelogram. If the coordinates of A,B,C are (−2,−1),(3,0) and (1,−2) respectively, find the coordinates of D.
View SolutionABCD is parallelogram. If the coordinates of A,B,C are (−2,−1),(3,0) and (1,−2) respectively, find the coordinates of D.
View SolutionThe vertices of a triangle ABC are A(0,0),B(2,−1)andC(9,2), find cosB.
View SolutionFind the angles of a triangle whose vertices are A(0,−1,−2),B(3,1,4) and C (5,7,1).
View SolutionIn a triangle ABC,∠A=600andb:c=(√3+1):2, then find the value of (∠B−∠C).
View SolutionIn a triangle ABC, ∠B=1050,∠C=500, Find ∠A
View Solution