ABCD is parallelogram. If the coordinates of A,B,C are (−2,−1),(3,0) and (1,−2) respectively, find the coordinates of D.
To find the coordinates of point D in the parallelogram ABCD, we can use the property that the diagonals of a parallelogram bisect each other. This means that the midpoint of diagonal AC will be the same as the midpoint of diagonal BD.
1. Identify the Coordinates of Points A, B, and C:
- A = (-2, -1)
- B = (3, 0)
- C = (1, -2)
2. Calculate the Midpoint of Diagonal AC:
- The formula for the midpoint M of two points (x1, y1) and (x2, y2) is:
M=(x1+x22,y1+y22)
- For points A and C:
MAC=(−2+12,−1+(−2)2)=(−12,−32)
3. Set Up the Midpoint of Diagonal BD:
- Let the coordinates of point D be (x, y).
- The midpoint of diagonal BD is:
MBD=(3+x2,0+y2)
4. Equate the Midpoints of AC and BD:
- Since the midpoints are equal, we can set up the following equations:
3+x2=−12(1)
0+y2=−32(2)
5. Solve for x from Equation (1):
- Multiply both sides by 2:
3+x=−1
- Subtract 3 from both sides:
x=−1−3=−4
6. Solve for y from Equation (2):
- Multiply both sides by 2:
y=−3
7. Conclusion:
- The coordinates of point D are:
D=(−4,−3)
Final Answer:
The coordinates of point D are (-4, -3).
- A = (-2, -1)
- B = (3, 0)
- C = (1, -2)
- The formula for the midpoint M of two points (x1, y1) and (x2, y2) is:
M=(x1+x22,y1+y22)
- For points A and C:
MAC=(−2+12,−1+(−2)2)=(−12,−32)
- Let the coordinates of point D be (x, y).
- The midpoint of diagonal BD is:
MBD=(3+x2,0+y2)
- Since the midpoints are equal, we can set up the following equations:
3+x2=−12(1)
0+y2=−32(2)
- Multiply both sides by 2:
3+x=−1
- Subtract 3 from both sides:
x=−1−3=−4
- Multiply both sides by 2:
y=−3
- The coordinates of point D are:
D=(−4,−3)
The coordinates of point D are (-4, -3).
Topper's Solved these Questions
Similar Questions
ABCD is a parallelogram. If the coordinates of A, B, C are (2,3), (1,4)and (0,−2) respectively, find the coordinates of D.
View SolutionThe centroid of a triangle ABC is at the point (1,1,1). If the coordinates of A and B are (3,-5,7) and (-1,7,-6) respectively, find the coordinates of the point C.
View Solution
Knowledge Check
In the rectangular coordinate system shown, ABCD is a parallogram. If the coordinates of the points A,B,C and D are (0,2),(a,b),(a,2) and (0,0), respectively, then b =
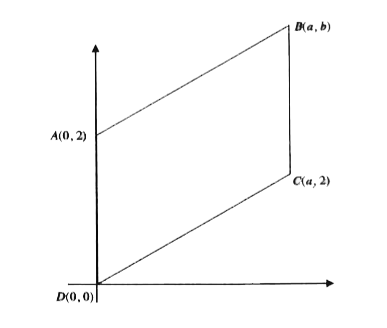 A0B2C3D4
A0B2C3D4
The centroid of a triangle ABC is at the point (1,1,1). If the coordinates of A and B are (3,5,7)and (1,7,6), respectively, find the coordinates of the point C.
View SolutionSuppose ABCD is a quadrilateral such that the coordinates of A, B and C are (1,3)(−2,6)and(5,−8) respectively. For what choices of coordinates of D will make ABCD a trapezium ?
View SolutionSuppose ABCD is a quadrilateral such that the coordinates of A, B and C are (1,3)(−2,6)and(5,−8) respectively. For what choices of coordinates of D will make ABCD a trapezium ?
View SolutionIf A and B are (1, 4) and (5, 2) respectively, find the coordinates of P when AP/BP=3/4 .
View SolutionThe three vertices of a parallelogram taken in order are (−1,0), (3,1) and (2,2) respectively. Find the coordinates of the fourth vertex.
View SolutionA ,B and C are the points (2,0),(5,0)and(5,3) respectively. Find coordinates of D such that ABCD is a square.
View SolutionThe coordinates of the mid points of sides AB, BC and CA of ABC are D(1,2,−3), E(3,0,1)and F(−1,1,−4) respectively. Write the coordinates of its centroid.
View Solution
