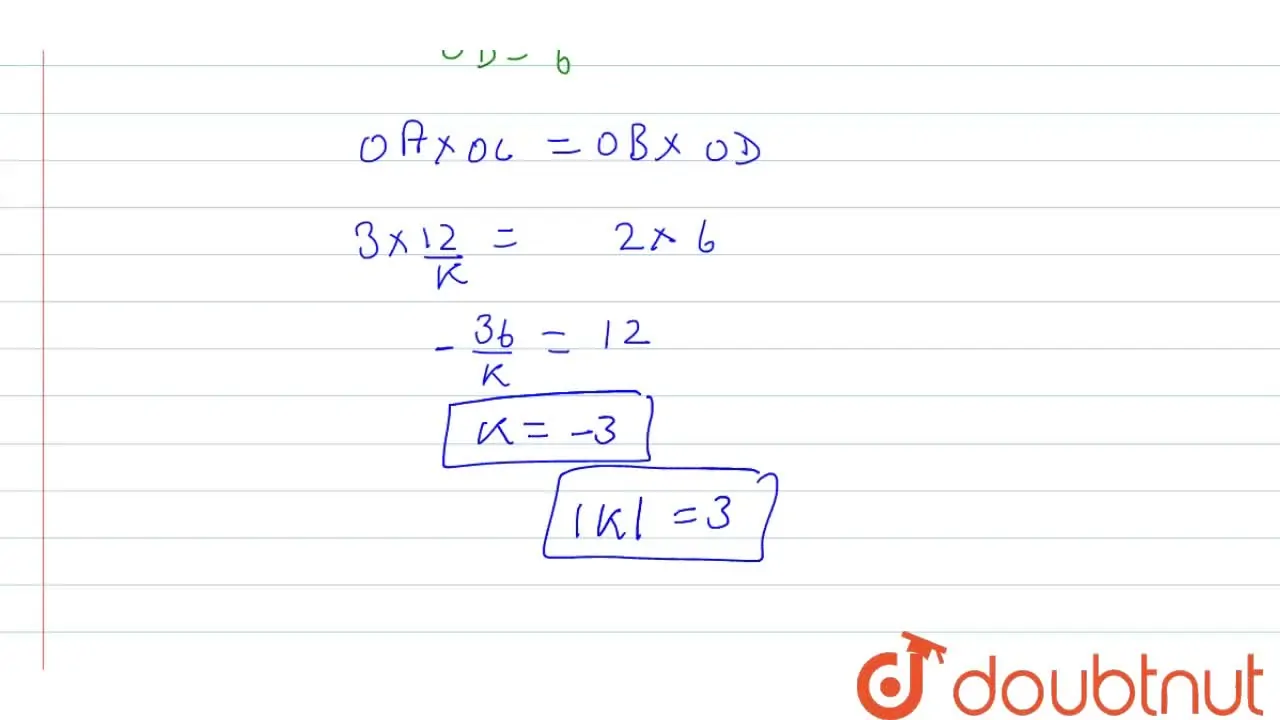
If lines 2x−3y+6=0 and kx+2y=12=0 cut the coordinate axes in concyclic points, then the value of |k| is
The correct Answer is:3
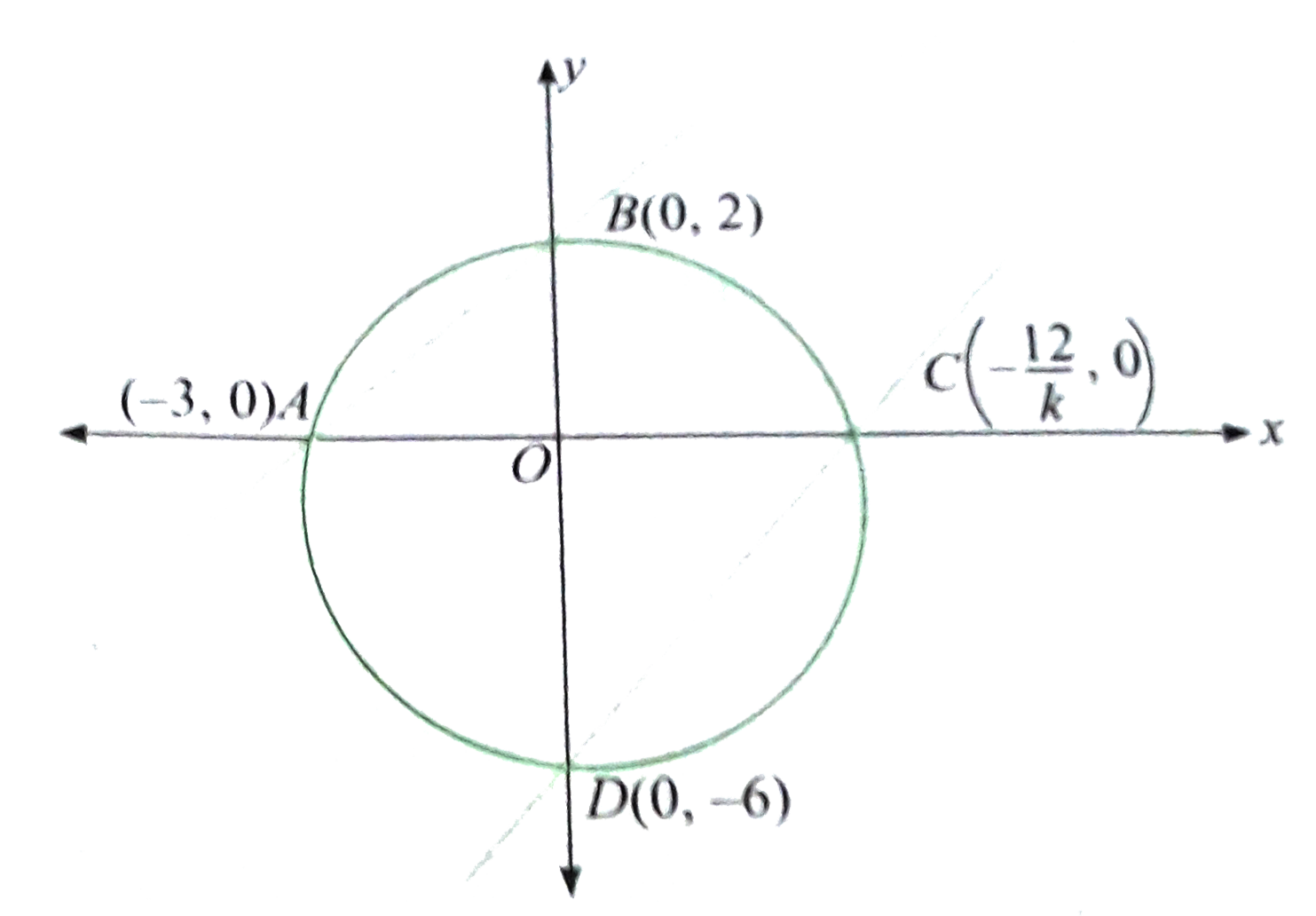
2x−3y+6=0 meets the axes at A(−3,0) and B(0,2). kx+2y+12=0 meets the axes at D(0,−6) and C(−12/k,0), Points A,B.C and D will be concyclic, if
OA×OC=OB×OD
⇒3×(−12/k)=2×6
⇒k=−3
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - JEE Main6videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Matrix match type4videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type2videos
COORDINATE SYSTEM
- BOOK - CENGAGE ENGLISHCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.213videos
CROSS PRODUCTS
Similar Questions
If the lines 2x−3y+7=0,3x+ky+5=0 cut the coordinate axes in concyclic points then k=
View SolutionIf the lines lx+2y+3=0 and 2y+mx+4=0 cut the co-ordinate axes in concylic points then lm=
View SolutionThe lines 2x+3y+19=0 and 9x+6y−17=0 , cut the coordinate axes at concyclic points.
View SolutionThe lines 2x+3y+19=0 and 9x+6y-17=0 cuts the coordinate axes in
View SolutionIf the lines x−2y+3=0,3x+ky+7=0 cut the coordinate axes in concylic points, then k=
View SolutionThe lines 2x−5y+1=0 and 10x−4y−3=0 meets the coordinate axes in concylic points, then equation to the circle is
View SolutionShow that the lines 3x−y+3=0 and x−3y−6=0 cut the coordinate axes at concylic points. Show that the equation of the circle passing through these points is x2+y2−5x−y−6=0
View SolutionIf the lines a1x+b1y+c1=0 and a2x+b2y+c2=0 cut the coordinae axes at concyclic points, then prove that |a1a2|=|b1b2|
View SolutionThe algebraic sum of distances of the line ax+by+2=0 from (1,2),(2,1)and(3,5) is zero and the lines bx−ay+4=0 and 3x+4y+5=0 cut the coordinate axes at concyclic points. Then (a) a+b=−27 (b) area of triangle formed by the line ax+by+2=0 with coordinate axes is 145 (c) line ax+by+3=0 always passes through the point (−1,1) (d) max {a,b}=57
View SolutionIf the lines 2x+3y+1=0,6x+4y+1=0 intersect the co-ordinate axes in 4 points, then the circle passing through the points is
View Solution