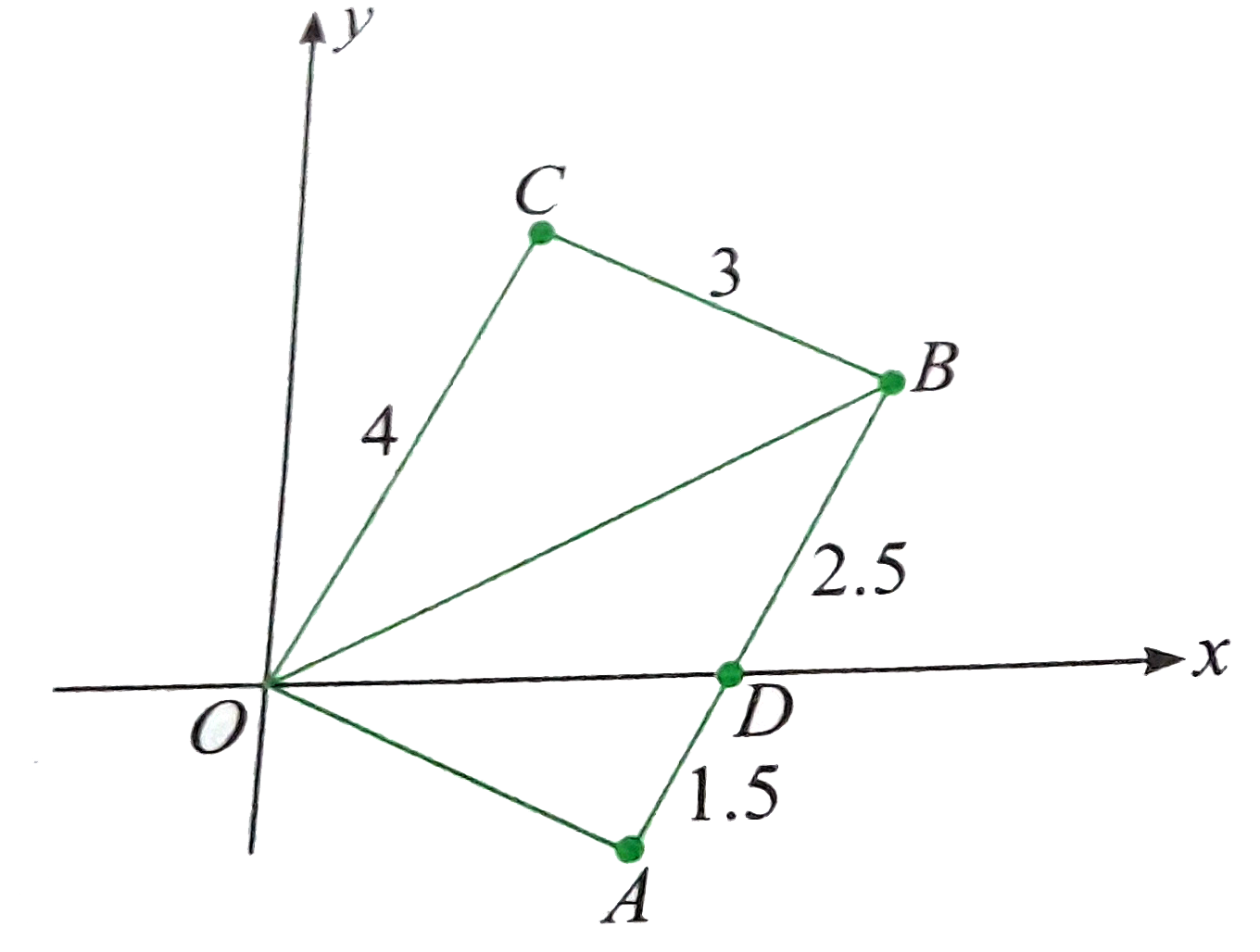
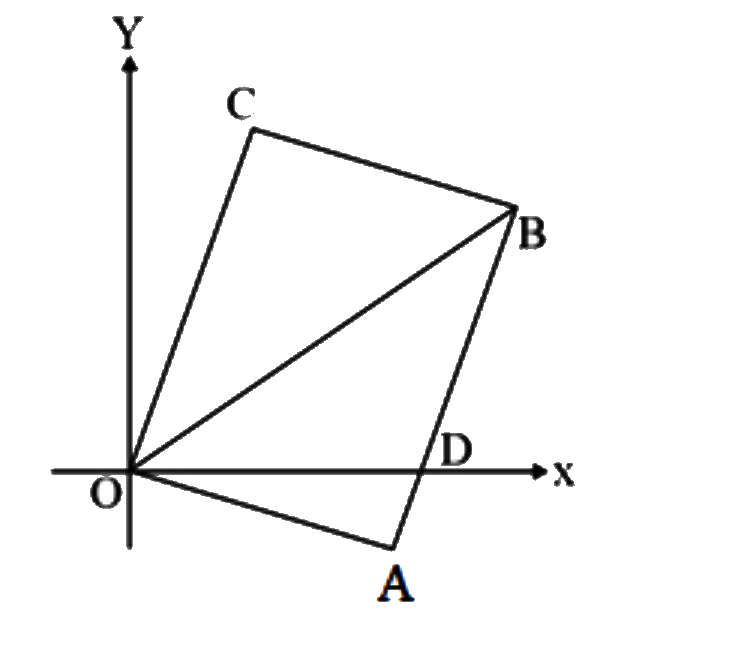
In the given figure, OABC is a rectangle. Slope of OB is
a. $1 / 4$ b. $1 / 3$ c. $1 / 2$ d. Cannot be determined

A

$1 / 4$

B

$1 / 3$

C

$1 / 2$

D

Cannot be determined

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Clearly, $O B = 5$
So, $\frac{O A}{O B} = \frac{3}{5} = \frac{1.5}{2.5} = \frac{A D}{D B}$
Thus, OD bisects $∠ A O B$ .
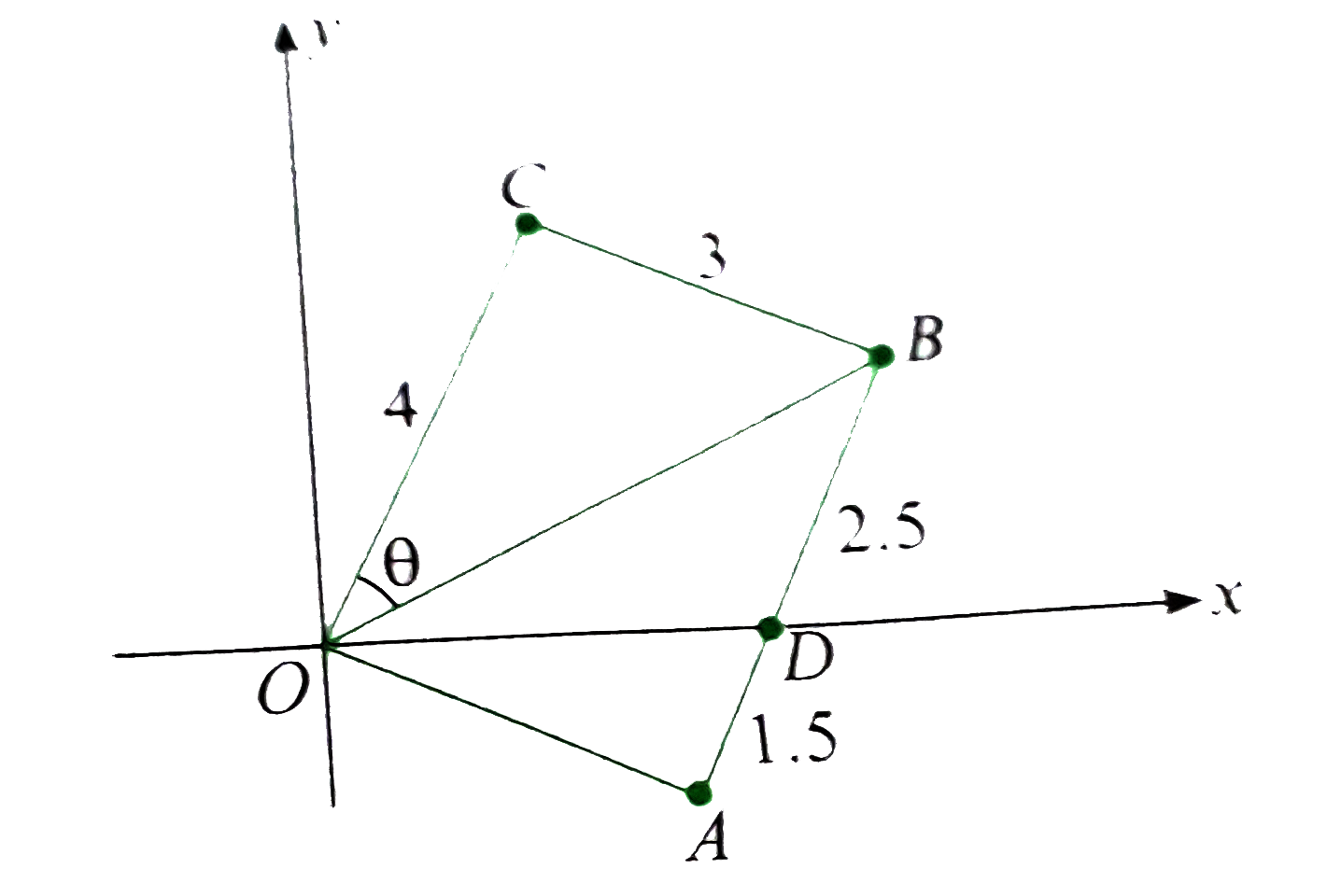
Let $∠ B O C = θ$ . Then $tan θ = \frac{3}{4}$
$∴ ∠ B O C = \frac{1}{2} ∠ A O B = \frac{1}{2} (90^{\circ} - θ)$
$∴$ Slope of $O B = \frac{tan (1)}{2} (90^{\circ} - θ) = \frac{{tan 45}^{\circ} - tan . \frac{θ}{2}}{1 + {tan 45}^{\circ} + tan . \frac{θ}{2}}$
Now, $tan θ = \frac{3}{4}$
$\Rightarrow \frac{2 tan . \frac{θ}{2}}{1 - {tan}^{2} . \frac{θ}{2}} = \frac{3}{4}$
$3 - 3 {tan}^{2} . \frac{θ}{2} = 8 tan . \frac{θ}{2}$
$\Rightarrow 3 {tan}^{2} . \frac{θ}{2} + 8 tan . \frac{θ}{2} - 3 = 0$
$\Rightarrow (3 tan . \frac{θ}{2} - 1) (tan . \frac{θ}{2} + 3) = 0$
So, $tan . \frac{θ}{2} = \frac{1}{3} (∵ \frac{θ}{2} is acute)$
$∴$ Slope of $O B = \frac{1 - \frac{1}{3}}{1 + \frac{1}{3}} = \frac{1}{2}$

|

Updated on:21/07/2023

Class 12 MATHS THREE DIMENSIONAL GEOMETRY

Topper's Solved these Questions

Similar Questions

CENGAGE ENGLISH-COORDINATE SYSYEM -Exercises

Home
Ask Doubt
Profile