Which of the following is highest?
(a) cosec 1 (b) cosec 2
(c) cosec 4 (d) cosec (−6)
Video Solution
Text Solution
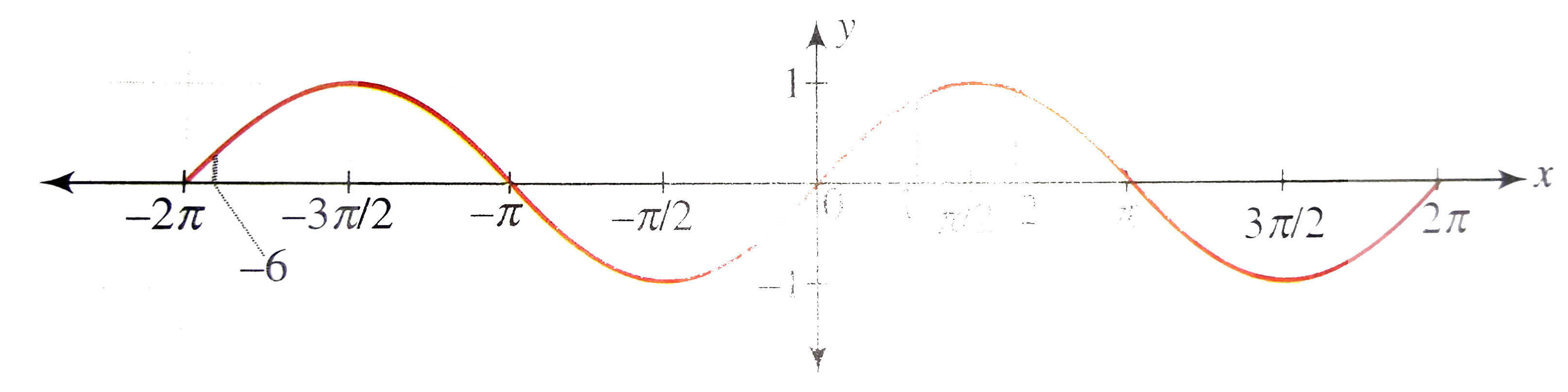
Consider sin 1, sin 2 and -sin 6 (sin 4 is negative, hence cosec 4 cannot be maximum).
Form the graph, sin (-6) is the least positive value, hence cosesc (-6) is maximum.
|
Topper's Solved these Questions
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercises
22 Videos
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGE ENGLISHCHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - EXERCISES
34 Videos
- HIGHT AND DISTANCE BOOK - CENGAGE ENGLISHCHAPTER - HIGHT AND DISTANCE EXERCISE - Archives
3 Videos
Similar Questions
cosec−1(2)
View Solution∫ cosec42xdx
View SolutionWhich of the following is the principal value branch of cosec−1x ?
View SolutionProve the following identities :
cosecA+cotA=1cosecA−cotA
View SolutionProve the following identities :
cosecA(1+cosA)(cosecA−cotA)=1
View SolutionProve the following identities :
cosecAcosecA−1+cosecAcosecA+1=2sec2A
View SolutionProve the following identities :
1+sinA1−sinA=cosecA+1cosecA−1
View SolutionFrom the following figure, find the values of :
 cosec C
cosec C
View SolutionProve the following identities :
(cosecA−cotA)2+1secA(cosecA−cotA)=2cotA
View SolutionProve the following identities :
cotA+cosecA−1cotA−cosecA+1=1+cosAsinA
View Solution
