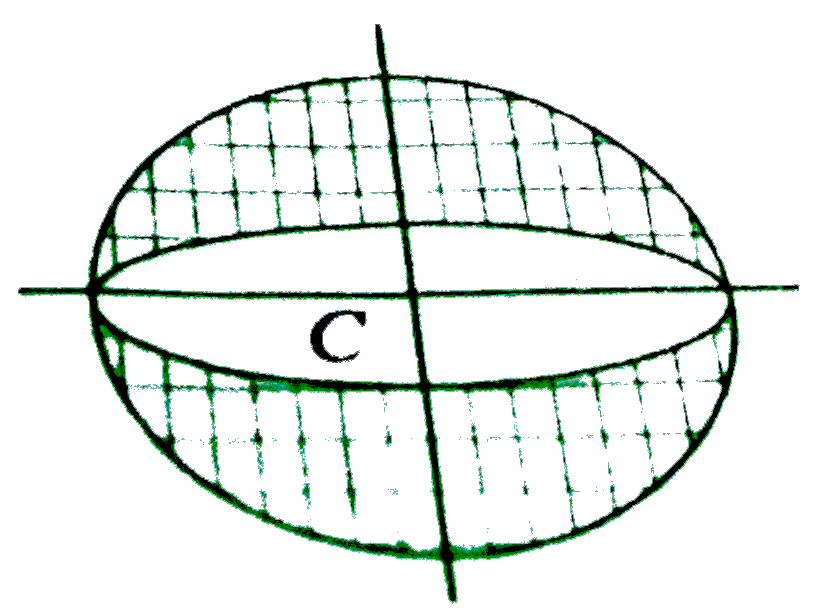
There are some experiment in which the outcomes cannot be identified discretely. For example, an ellipse of eccentricity is inscribed in a circle and a point within the circle is chosen at random. Now, we want to find the probability that this point lies outside the ellipse. Then, the point must lie in the shaded region shown in Figure. Let the radius of the circle be a and length of minor axis of the ellipse be 2b. Given that
Then, the area of circle serves as sample space and area of the shaded region represents the area for favorable cases. Then, required probability is
Now, answer the following questions.
Two persons A and B agree to meet at a place between 5 and 6 pm. The first one to arrive waits for 20 min and then leave. If the time of their arrival be independant and at random, then the probability that A and B meet is
The correct Answer is:A
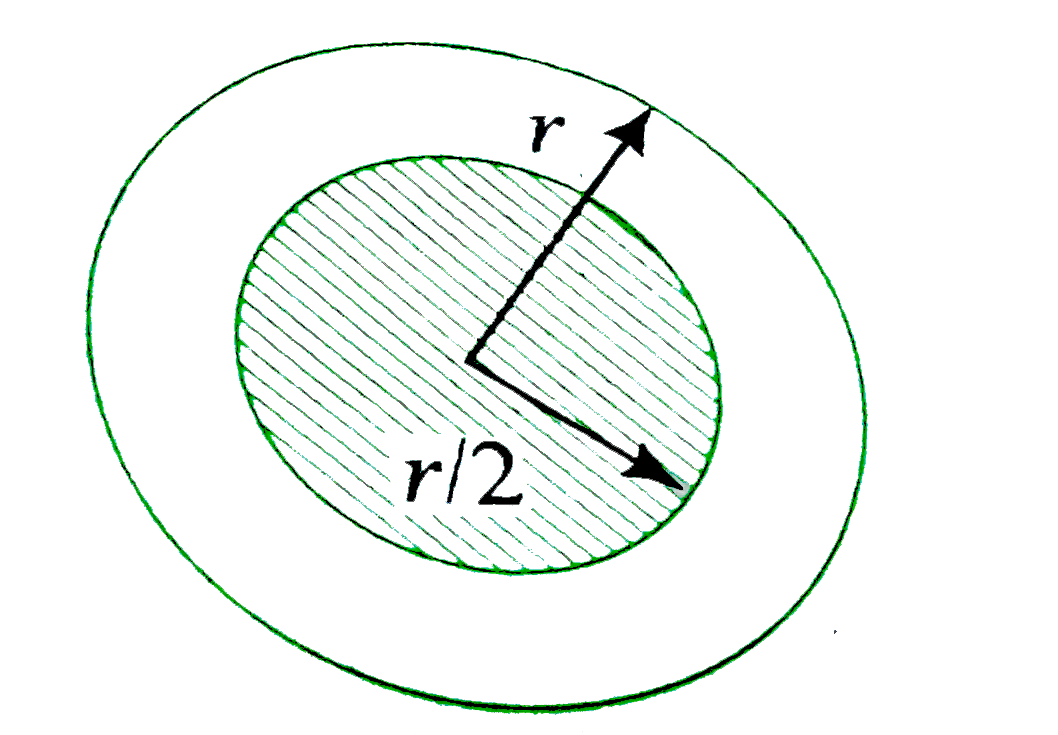
For the favorable cases, the points should lie inside the concentric circle of radius . So the desired probability is given by
Topper's Solved these Questions
- PROBABILITY I Book:CENGAGE ENGLISHChapter:PROBABILITY I Exercise:Match
Explore 2 Videos
- PROBABILITY I Book:CENGAGE ENGLISHChapter:PROBABILITY I Exercise:Numerical Value Type
Explore 7 Videos
- PROBABILITY I Book:CENGAGE ENGLISHChapter:PROBABILITY I Exercise:MCQ
Explore 54 Videos
- PROBABILITYBook:CENGAGE ENGLISHChapter:PROBABILITYExercise:Comprehension
Explore 2 Videos
- PROBABILITY IIBook:CENGAGE ENGLISHChapter:PROBABILITY IIExercise:MULTIPLE CORRECT ANSWER TYPE
Explore 6 Videos
Similar Questions
An ellipse of eccentricity 2√23 is inscribed in a circle and a point within the circle is chosen at random. Let the probability that this point lies outside the ellipse be p. Then the value of 105 p is
View SolutionAn ellipse is inscribed in a circle and a point within the circle is chosen at random. If the probability that this point lies outside the ellipse is 23 then the eccentricity of the ellipse is: (A) 2√23 (B) √53 (C) 89 (D) 23
View SolutionA square is incribed in a circle . If p1 is the probability that a randomly chosen point of the circle lies within the square and p2 is the probability that the point lies outside the square then
View SolutionA point is selected at random from inside a circle. The probability that the point is closer to the circumference of the circle than to its centre, is
View SolutionThree points P,QandR are selected at random from the cirumference of a circle. Find the probability p that the point lie on a semi-circle
View SolutionA circle of maximum area is inscribed in an ellipse. If p is the probability that a point within the ellipse chosen at random lies outside the circle, then the eccentricity of the ellipse is
View SolutionA circle has its centre at the origin and a point P (5,0) lies on it . The point Q (6,8) lies outside the circle.
View SolutionRectangle ABCD is inscribed in a circle. If the radius of the circle is 2 and ¯¯¯¯¯¯CD=2, what is the area of the shaded region ?

View SolutionThe triangles inside △ABC , shown above, are formed by joining the midpoints of the sides and then repeating the process. If a point is chosen at random inside △ABC ,what is the probability that the point lies in the shaded region ?
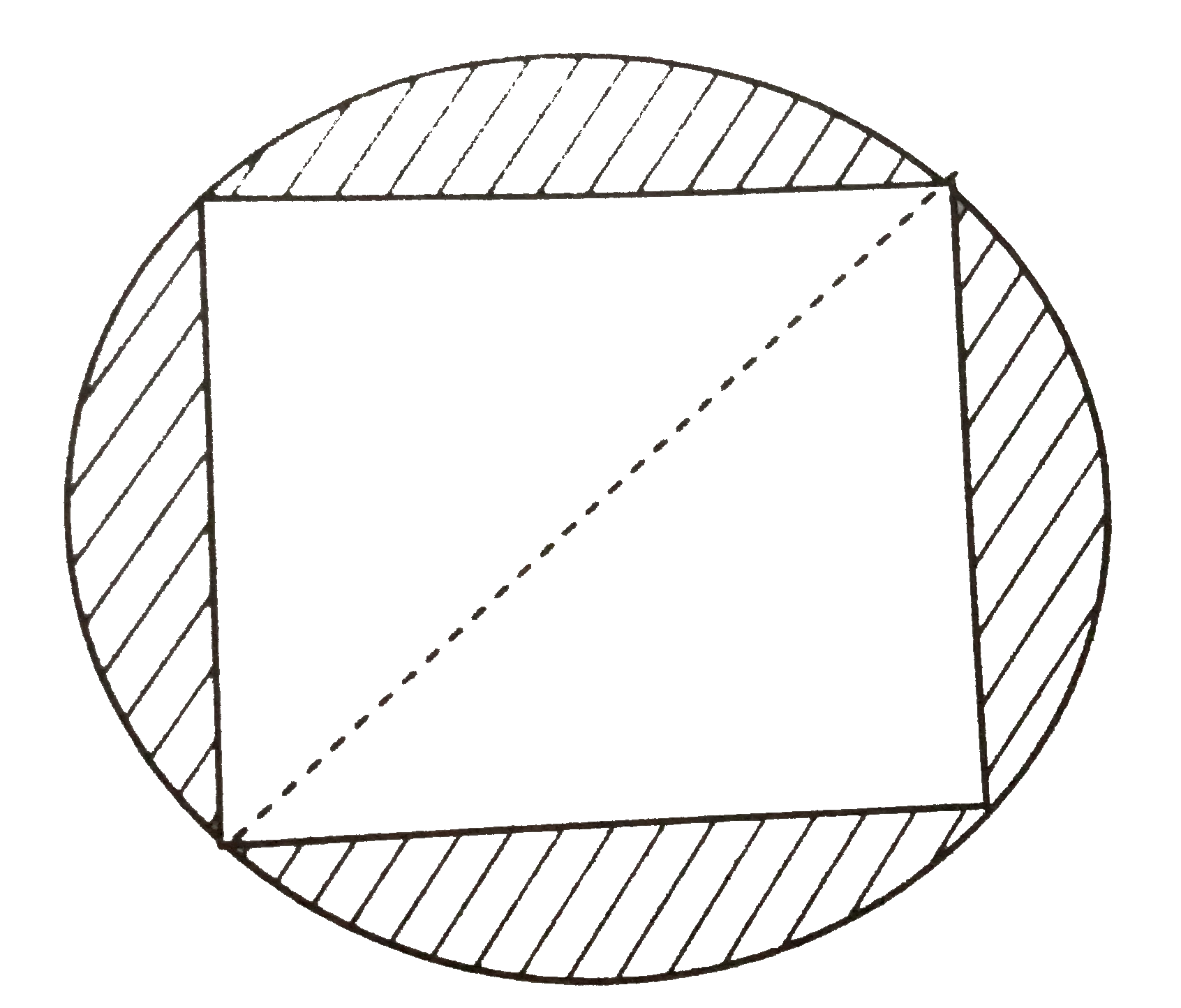
View SolutionIn figure, a square of diagonal 8 cm is inscribed in a circle. Find the area of the shaded region.
View Solution