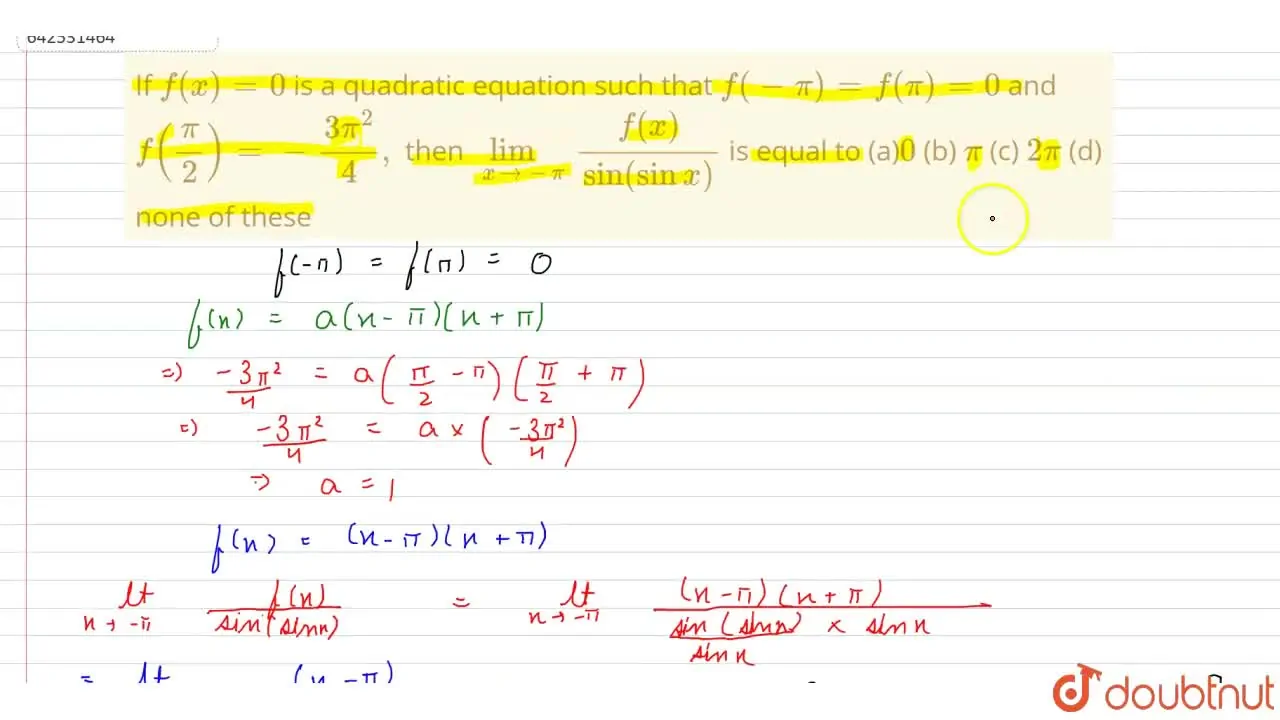
If f(x)=0 is a quadratic equation such that f(−π)=f(π)=0 and f(π2)=−3π24, then limx→−πf(x)sin(sinx) is equal to (a)0 (b) π (c) 2π (d) none of these
−32π
−12
(2π)
32π
Given f(x)=x2−π2
limx→−π+x2−π2sin(sinx)=limh→0(−π+h)2−π2sin(sin(−π+h))
=limh→0−2hπ+h2−sin(sinh)
=limh→0h−2π−sin(sinh)sinh×sinhh=2π
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - LINEAR INEQUALITIESEXERCISE - All Questions68videos
LINEAR INEQUALITIES
Similar Questions
If f(x)=0 is a quadratic equation such that f(−π)=f(π)=0 and f(π2)=−3π24, then limx→−π+f(x)sin(sinx) is equal to
View SolutionThe range of f(x)=sin−1(x2+1x2+2) is (a)[0,π2] (b) (0,π6) (c) [π6,π2] (d) none of these
View SolutionThe range of f(x)=sin−1(x2+1x2+2) is (a)[0,π2] (b) (0,π6) (c) [π6,π2] (d) none of these
View SolutionIf f(x) is a continuous function in [0,π] such that f(0)=f(x)=0, then the value of
∫π/20{f(2x)−f''(2x)}sinxcosxdx is equal toView Solutionf(x)=sin+√3cosx is maximum when x= π3 (b) π4 (c) π6 (d) 0
View SolutionA function f(x) is such that f(x+π2)=π2−|x|∀x. Find f(π2), if it exists.
View SolutionIf f(x)=sin−1cosx, then the value of f(10)+f′(10) is (a)11−π2 (b) π2−11 (c)5π2−11 (d) none of these
View SolutionIf f(x)=sinx+cosx and g(x)={|x|x,x≠02,x=0 then the value of ∫2π−π/4gof(x)dx is equal to (a) 3π4 (b) π4 (c) π (d) None of these
View SolutionIf f(x)=∫x0ecostecost+e−(cost)dt, then 2f(π)= (A) 0 (B) π (C) −π (D) none of these
View SolutionIf f(x) is continuous in [0,π] such that f(π)=3and∫π20(f(2x)+f''(2x))sin2xdx=7 then find f(0).
View Solution