As electric lamp and a conductor of resistance 4Ω are connected in series to a 6 V battery. The current drawn by the lamp is 0.25 A. Find the resistance of the electric lamp.
To find the resistance of the electric lamp when it is connected in series with a conductor of resistance 4Ω to a 6V battery, and the current drawn by the lamp is 0.25A, we can follow these steps:
Step 1: Use Ohm's Law
Ohm's Law states that:
V=I×R
Where:
- V is the voltage (in volts),
- I is the current (in amperes),
- R is the resistance (in ohms).
Step 2: Calculate the Total Resistance
Given that the total voltage V is 6V and the current I is 0.25A, we can rearrange Ohm's Law to find the total resistance R:
R=VI=6V0.25A
Step 3: Perform the Calculation
Now, calculate the total resistance:
R=60.25=24Ω
Step 4: Relate Total Resistance to Individual Resistances
In a series circuit, the total resistance R is the sum of the individual resistances:
Rtotal=Rlamp+Rconductor
Where:
- Rlamp is the resistance of the electric lamp,
- Rconductor=4Ω.
Step 5: Solve for the Resistance of the Lamp
Now, we can rearrange the equation to find the resistance of the lamp:
Rlamp=Rtotal−Rconductor=24Ω−4Ω
Step 6: Perform the Final Calculation
Now, calculate the resistance of the lamp:
Rlamp=24−4=20Ω
Final Answer
The resistance of the electric lamp is 20Ω.
---
Ohm's Law states that:
V=I×R
Where:
- V is the voltage (in volts),
- I is the current (in amperes),
- R is the resistance (in ohms).
Given that the total voltage V is 6V and the current I is 0.25A, we can rearrange Ohm's Law to find the total resistance R:
R=VI=6V0.25A
Now, calculate the total resistance:
R=60.25=24Ω
In a series circuit, the total resistance R is the sum of the individual resistances:
Rtotal=Rlamp+Rconductor
Where:
- Rlamp is the resistance of the electric lamp,
- Rconductor=4Ω.
Now, we can rearrange the equation to find the resistance of the lamp:
Rlamp=Rtotal−Rconductor=24Ω−4Ω
Now, calculate the resistance of the lamp:
Rlamp=24−4=20Ω
The resistance of the electric lamp is 20Ω.
Similar Questions
An electric lamp of resistance 2Ω and a conductor of resistance 4Ωare connected to a
6V Battery as shown in the circuit. Calculate:
(a) the totak resistance of the circuit,
(b) the current through the circuit,
(c) the potential difference across the (i) electric lamp
and (ii) conductor, and
(d) power of the lamp.
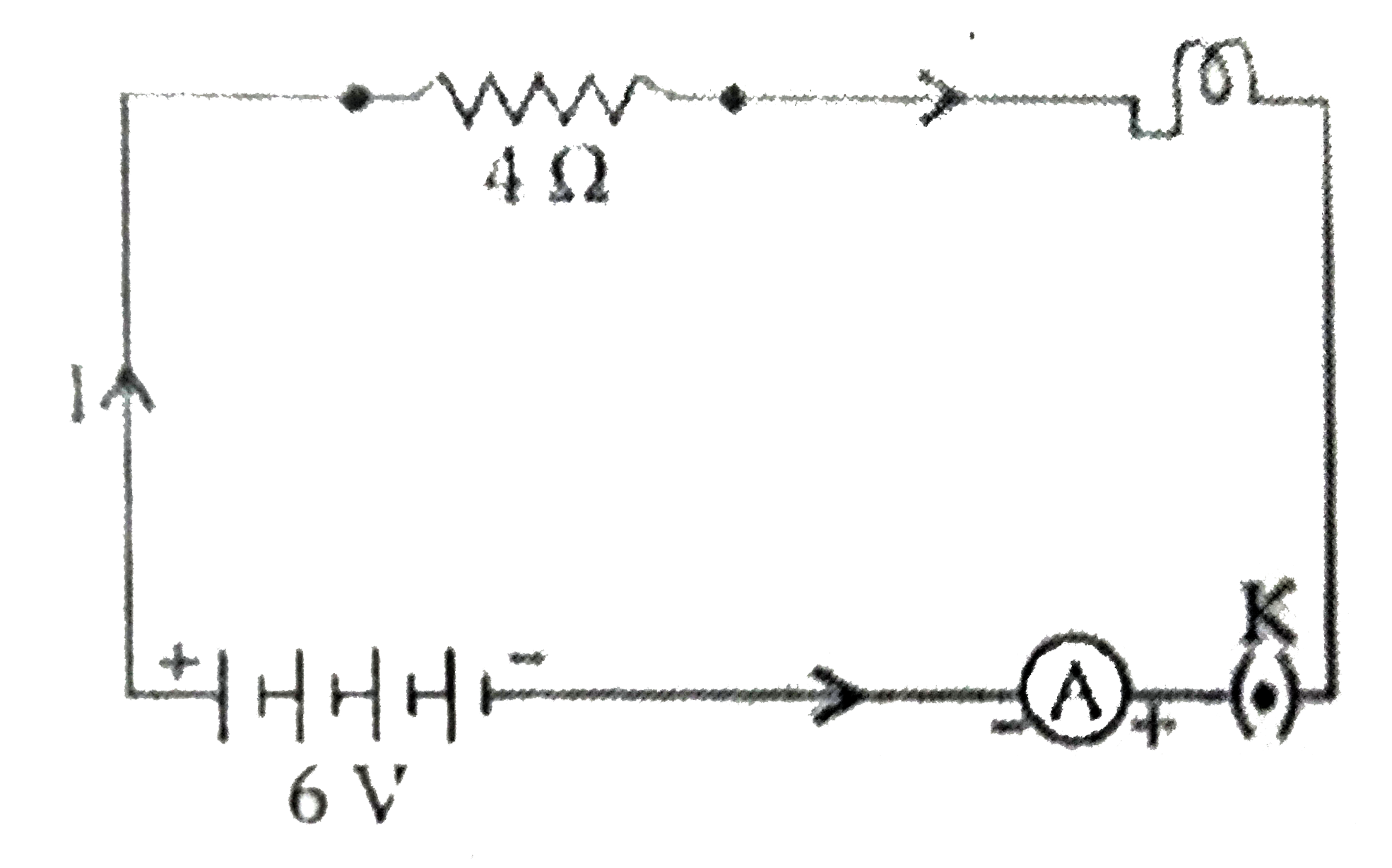
View SolutionA coil of inductance 8.4mH and resistance 6 Ω is connected to a 12V battery. The current in the coil is 1.0A at approximately the time.
View Solution
Knowledge Check
A battery of emf 15 V and internal resistance of 4Ω is connected to a resistor. If the current in the circuit is 2A and the circuit is closed. Resistance of the resistor and terminal voltage of the battery will be
A2.5Ω,6VB3.5Ω,6VC2.5Ω,7VD3.5Ω,7V
A coil of resistance 40Ω is connected across a 4.0 V battery. 0.10 s after the battery is connected, the current in the coil is 63 mA. Find the inductance of the coil.
View Solution(a) A current of 1A flows in a series curcuit containing an electric lamp and a conductor of 5Ω when connected to a 10V battery. Calculate the resistance of the electric lamp.
(b) Now if a resistance of 10Ω is connected in parallel with this series combination, what change (if any) in current flowing through 5Ω conductor and potential difference across the lamp will take place ? Give reason.
View SolutionA coil of inductance 8.4 mH and resistance 6(Ω) is connected to a 12 V battery. The current in the coil is 1.0 A at approximately the time
View SolutionAn inductor of 2 H and a resistance of 10Ω are connects in series with a battery of 5 V. The initial rate of change of current is
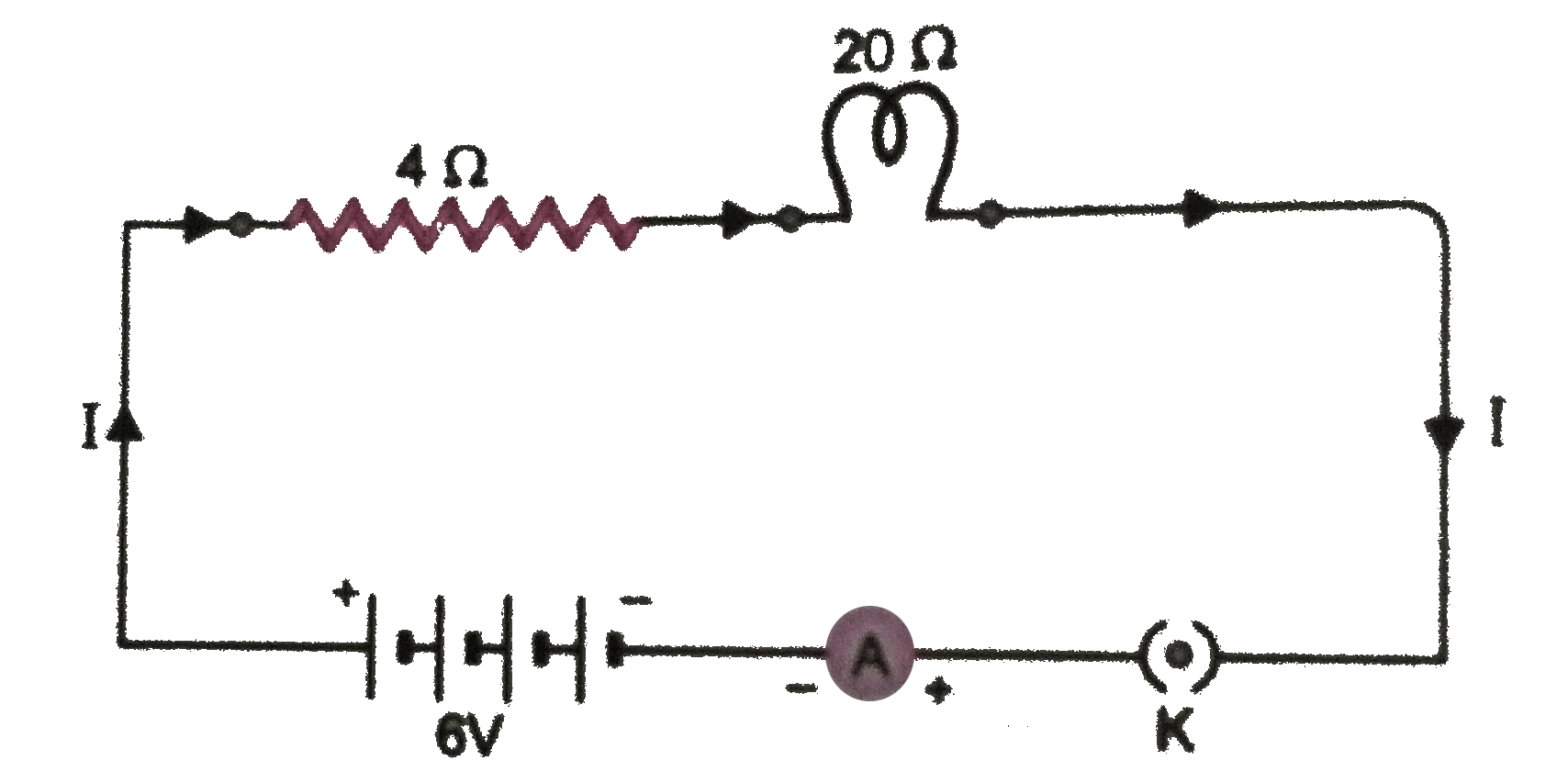
View SolutionAn electric lamp, whose resistance is 20Ω and a conductor of 4Ω resistance are connected to a 6V battery as shown in (Fig. 3.18) Calculate.
(a) the total resistance of the circuit,
(b) the current through the circuit, and
( c) the potential difference across the electric lamp and the conductor.
 .
.
View SolutionThree identical cells each of emf 2 V and internal resistance 1Ω are connected in series to form a battery. The battery is then connected to a parallel combination of two identical resistors, each of resistance 6Ω Find the current delivered by the battery.
View Solutionthe current in a circuit containing a battery connected to 2Ω resistance is 0.9A. When ana additional resistance of 8Ω is connected to the same battery the current observed in the circuit is 0.3A. Then the internal resistance of the battery is xΩ. Find value of x.
View Solution