Question

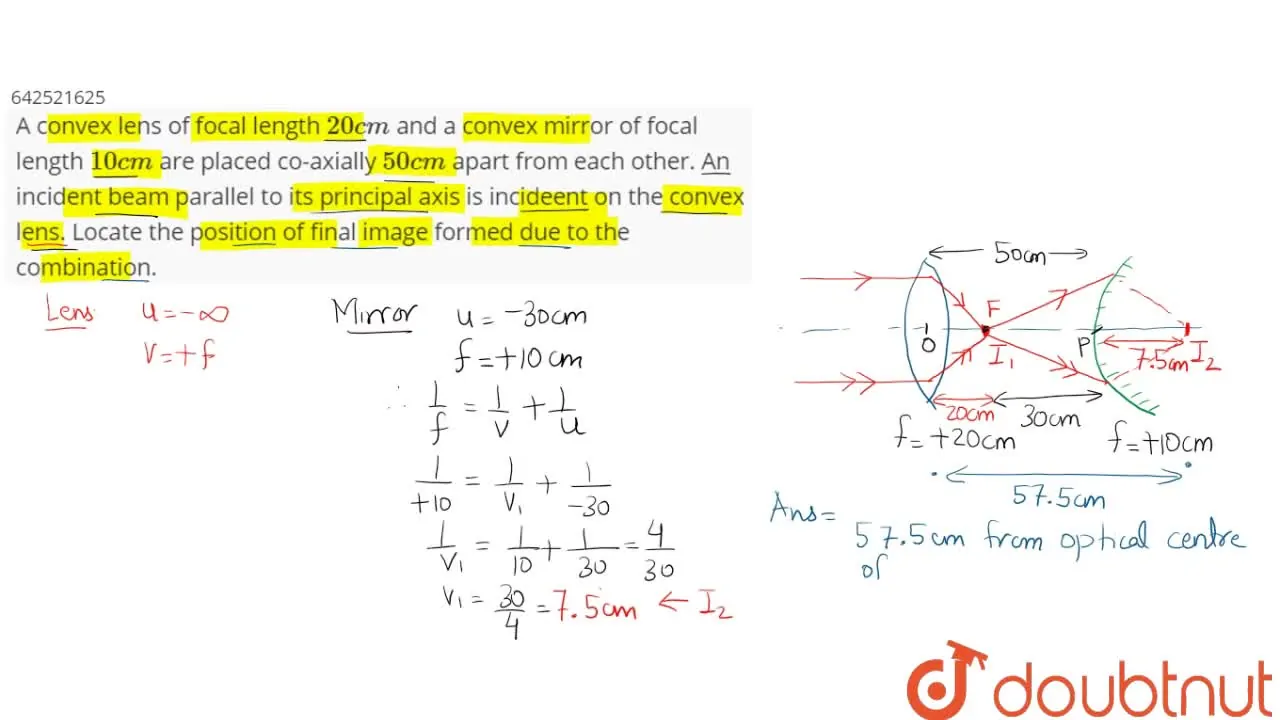
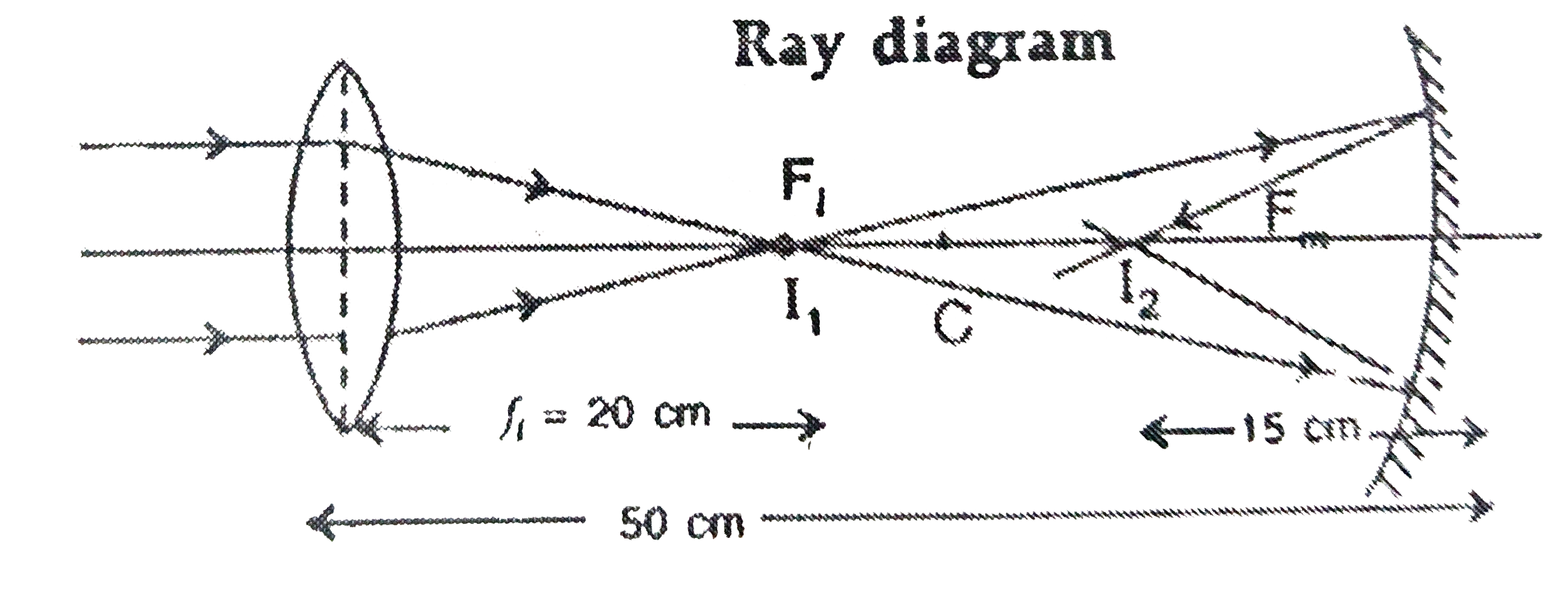
A convex lens of focal length $20cm$ and a convex mirror of focal length $10cm$ are placed co-axially $50cm$ apart from each other. An incident beam parallel to its principal axis is incideent on the convex lens. Locate the position of final image formed due to the combination.

Explanation

To solve the problem step-by-step, we will follow these steps:

Step 1: Identify the components and their properties
- We have a convex lens with a focal length (f_lens) of +20 cm.
- We have a convex mirror with a focal length (f_mirror) of +10 cm.
- The distance between the lens and the mirror is 50 cm.

Step 2: Determine the image formed by the convex lens
Since the incident beam is parallel to the principal axis, it will converge at the focal point of the lens.

Using the lens formula:
\( \frac{1}{f} = \frac{1}{v} - \frac{1}{u} \)
Where:
- \( f = +20 \) cm (focal length of the lens)
- \( u = -\infty \) (object distance for parallel rays)

For parallel rays:
\( \frac{1}{20} = \frac{1}{v} - 0 \)
Thus, the image distance \( v \) from the lens is:
\( v = 20 \text{ cm} \)

Step 3: Locate the position of the image with respect to the mirror
The image formed by the lens (I1) is 20 cm from the lens. Since the lens and mirror are 50 cm apart, the distance of the image from the mirror (u_mirror) is:
\( u_{mirror} = 50 - 20 = 30 \text{ cm} \)
Since the object is in front of the mirror, we take this as negative:
\( u_{mirror} = -30 \text{ cm} \)

Step 4: Use the mirror formula to find the final image position
Using the mirror formula:
\( \frac{1}{f} = \frac{1}{v} + \frac{1}{u} \)
Where:
- \( f = +10 \) cm (focal length of the mirror)
- \( u = -30 \) cm (object distance for the mirror)

Substituting the values:
\( \frac{1}{10} = \frac{1}{v_{mirror}} + \frac{1}{-30} \)
Rearranging gives:
\( \frac{1}{v_{mirror}} = \frac{1}{10} + \frac{1}{30} \)
Finding a common denominator (30):
\( \frac{1}{v_{mirror}} = \frac{3}{30} + \frac{1}{30} = \frac{4}{30} \)
Thus:
\( v_{mirror} = \frac{30}{4} = 7.5 \text{ cm} \)

Step 5: Determine the final image position with respect to the lens
The final image (I2) is formed at a distance of 7.5 cm from the mirror. Since the mirror is 50 cm from the lens, the final image position from the lens is:
\( \text{Final image position} = 50 - 7.5 = 57.5 \text{ cm} \)

Final Answer:
The final image formed due to the combination of the convex lens and convex mirror is located at 57.5 cm from the optical center of the lens.

---