Using Biot-Savart’s law, derive an expression for magnetic field at any point on axial line of a current carrying circular loop. Hence, find magnitude of magnetic field intensity at the centre of circular coil.
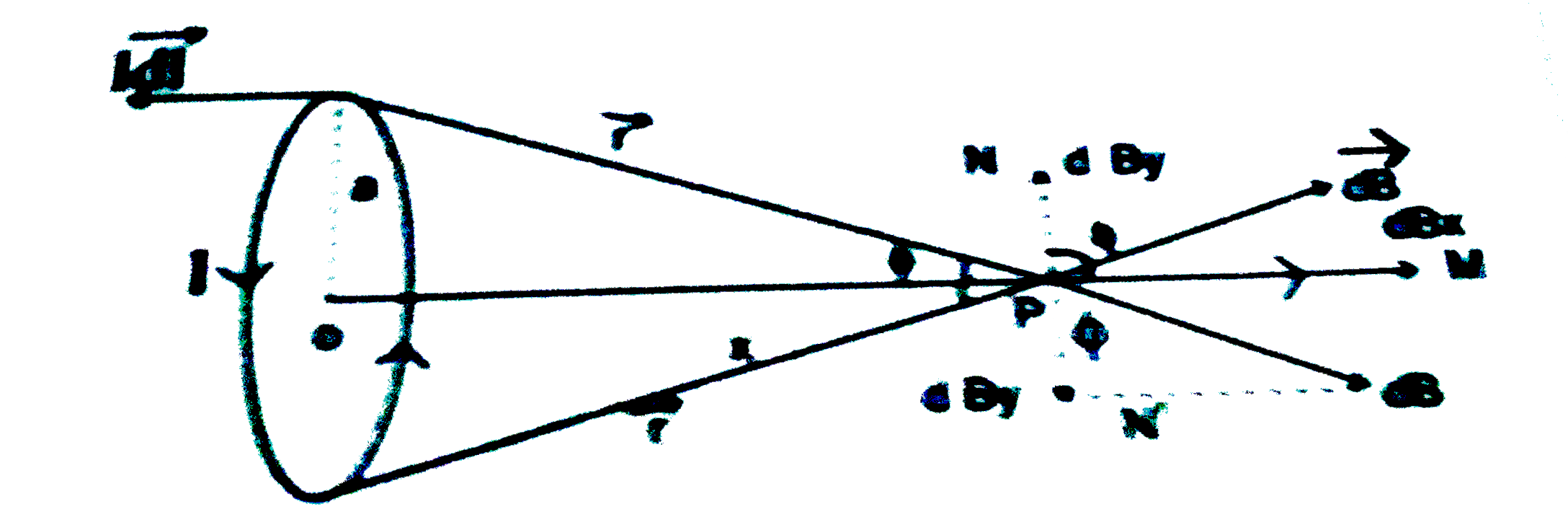
According to Biot-Savart's law, magnetic field due to a current element is given by
−→dB=μ04πI→dlX¯xr2 Where r=√x2+a2
∴dB=μ04πIdlsin90∘x2+e2
And direction of −→dB is ⊥ to the plane containing I→dl and →r.
Resolving −→dB alonhg the x-axis and y-axis.
dBx=dBSinθ
dBy=dB cosθ
taking the contribution of whole current loop we get
Bx∮Bx=∮Bsinθ=∫μ04π1dlx2+a2a√x&2+a2.
Bx=μ04π1n(x2+a2)E/Z∮dl=μ04πln×2πa(x2+a2)E/Z
And By=∮dBy=∮dBcosθ=0
∴Bp=√B2x+B2y=Bx=μ04π2IA(x2+a2)E/Z
∴−→Bp=μ04π2m(x2+a2)(∵→m=IA)
For centre x = 0
∴∣∣∣−→B0∣∣∣=μ04π2Iπa2a2=μ0(l2a) in the direction of →m
Topper's Solved these Questions
- SAMPLE PAPER 2019Book:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:SAMPLE PAPER 2019Exercise:SECTION D
Explore 6 Videos
- SAMPLE PAPER 2019Book:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:SAMPLE PAPER 2019Exercise:SECTION B
Explore 9 Videos
- EXAM REVISION SERIESBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:EXAM REVISION SERIESExercise:All Questions
Explore 41 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:SECTION - B
Explore 7 Videos
Similar Questions
Use Biot-Savart law to derive the expression for the magnetic field on the axis of a current carrying circular loop of radius R.
Draw the magnetic field lines due to circular wire carrying current I.
View SolutionThe magnetic field at the centre of the current carrying coil is
View SolutionFind the magnetic field induction at a point on the axis of a circular coil carrying current and hence find the magnetic field at the centre of circular coil carrying current.
View SolutionWhat will be magnetic field at centre of current carrying circular loop of radius R?
View SolutionFind the magnetic field at the centre of the circular loop shown in figure.

View Solution(a) Using Biot-Savart's law, derive an expression for the magnetic field at the centre of a circular coil of radius R, number of turns N, carrying current.
(b) Two small identical circular coils marked 1,2 carry equal currents and are placed with their geometric axes perpendicular to each other as shown in the figure. Derive an expression for the resultant magnetic field at O.
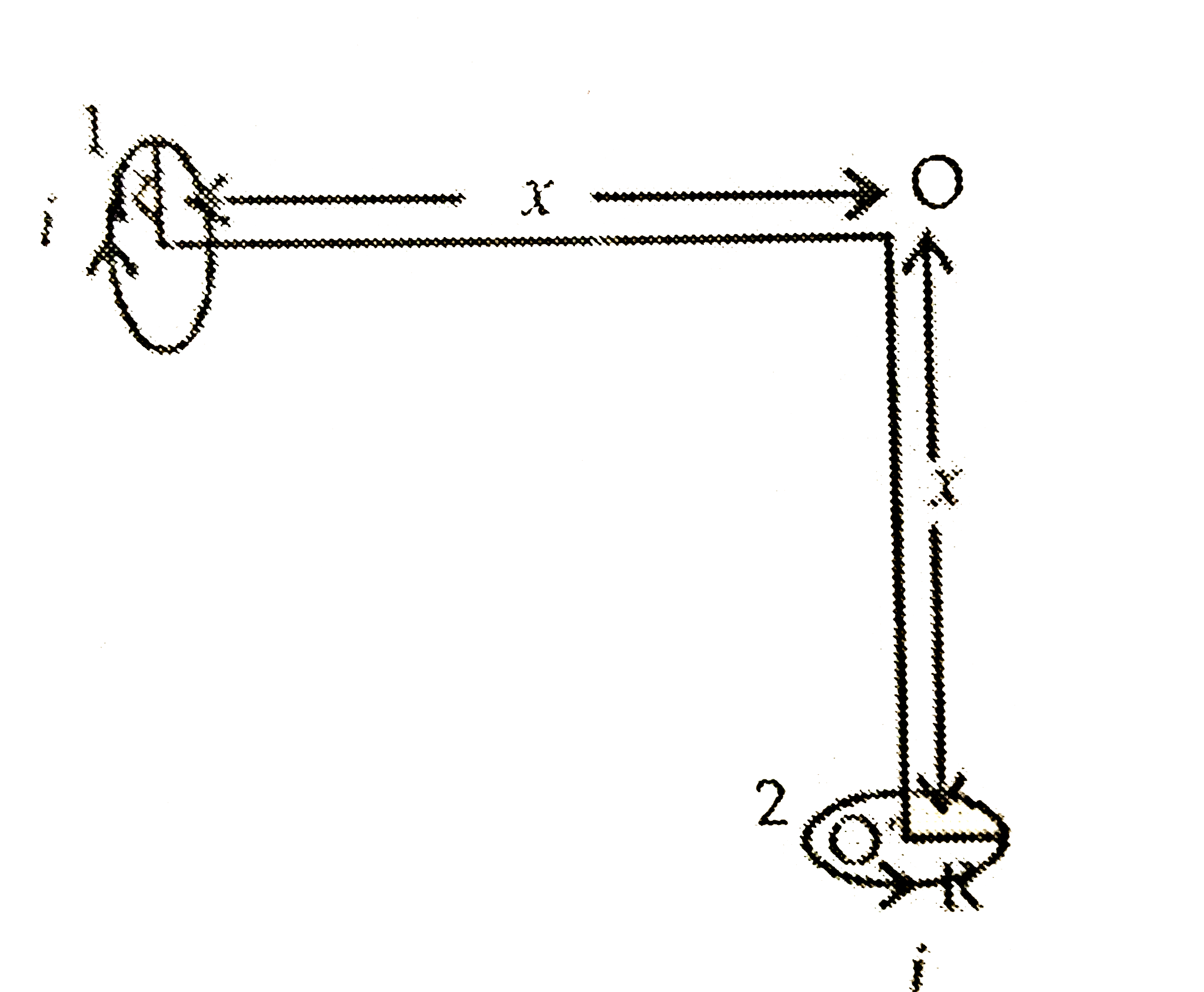
View Solution(a) Using Biot-Savart's law, derive the expression for the magnetic field in the vector form at a point on the axis of a circular current loop.
(b) What does a toroid consist of? Find out the expression for the magnetic field inside a toroid for N turns of the coil having the average radius r and carrying a current I. Show the magnetic field in the open space inside and exterior to the toroid is Zero.
View Solution(a) State Biot - Savart law and ecpress it in the vector form.
(b) Using Biot - Savart law, law obtain the expression for the magnetic field due to a circular coil of radius r, carrying a current I at point on its axis distant x from the centre of the coil.
View SolutionB0 is the magnetic field at point P for a given straight wire as shown in figure. If this wirre is bent into a circular loop, then magnetic field at the centre of loop is
_PHY_E06_130_Q01.png)
View SolutionThe magnetic field at centre of a hexagonal coil of side l carrying a current I is

View Solution