An open box, with a square base, is to be made out of a given quantity of metal sheet of area C2. Show that the maximum volume of the box is C36√3
As base of the box is square,
So, area of the box =a2+4ah
Here, a is the side of suare base and h is the height.
Now, it is given that,
C2=a2+4ah
⇒h=14[C2−a2a]
Now, Volume of the box(V)=a⋅a⋅h=a2h
⇒V=14[C2−a2a]a2
⇒V=14[aC2−a3]→(1)
⇒dVda=14[C2−3a2]
Now, for maximum volume, dVda=0
⇒14[C2−3a2]=0
⇒C2=3a2⇒a=C√3
Now, Putting value of a in (1),
⇒Vmax=14⎡⎣(C√3)(C2)−(C√3)3⎤⎦
⇒Vmax=C36√3
So, maximum volume of the box is C36√3 cubic units.
Topper's Solved these Questions
Similar Questions
An open box with a square base is to be made out of a given quantity of card board of area c2 square units. Show that the maximum volume of the box is c36√3 cubic units.
View SolutionAn open box with a square base is to be made out of a given quantity of card board of area c2 square units. Show that the maximum volume of the box is c36√3 cubic units.
View Solution
Knowledge Check
A 3-inch-tall rectangular box with a square base is constructed to hold a circular pie that has a diameter of 8 inches. Both are shown below. What is the volume, in cubic inches, of the smallest such box that can hold this pie?
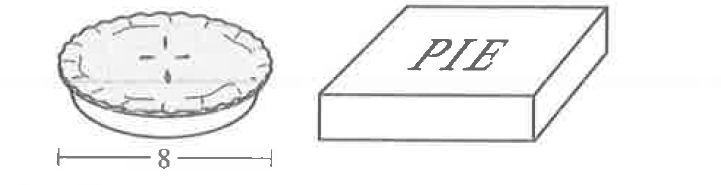 A24B64C72D192
A24B64C72D192
OR An open box with a square base is to be made out of a given quantity of cardboard of area c2 square units. Show that the maximum volume of the box is c36 √3 cubic units.
View SolutionAn open tank with a square base and vertical sides is to be constructed form a metal sheet so as to hold a given quantity of water. Show that the cost of material will be least when depth of tank is half its width. If the cost is to be borne by nearby settled lower income families, for whom water will be provided, what kind of value is hidden in the question
View SolutionAn open tank is to be constructed with square base and vertical sides so as to contain a given quantity of water. Show that the expenses of lining with lead will be least, if depth is made half of width.
View SolutionAn open tank is to be constructed with square base and vertical sides so as to contain a given quantity of water. Show that the expenses of lining with lead will be least, if depth is made half of width.
View SolutionA square piece of tin of side 18 cm is to be made into a box without top by cutting a square from each corner and folding up the flaps to form a box. What should be the side of the square to be cut off so that the volume of the box is maximum? Also, find the maximum volume.
View SolutionA square piece of tin of side 18 cm is to be made into a box without top, by cutting a square from each corner and folding up the flaps to form the box. What should be the side of the square to be cut off so that the volume of the box is the maximum possible?
View SolutionThe volume of a closed rectangular metal box with a square base is 4096 cm3. The cost of polishing the outer surface of the box is Rs 4 per cm2. Find the dimensions of the box for the minimum cost of polishing it.
View Solution
