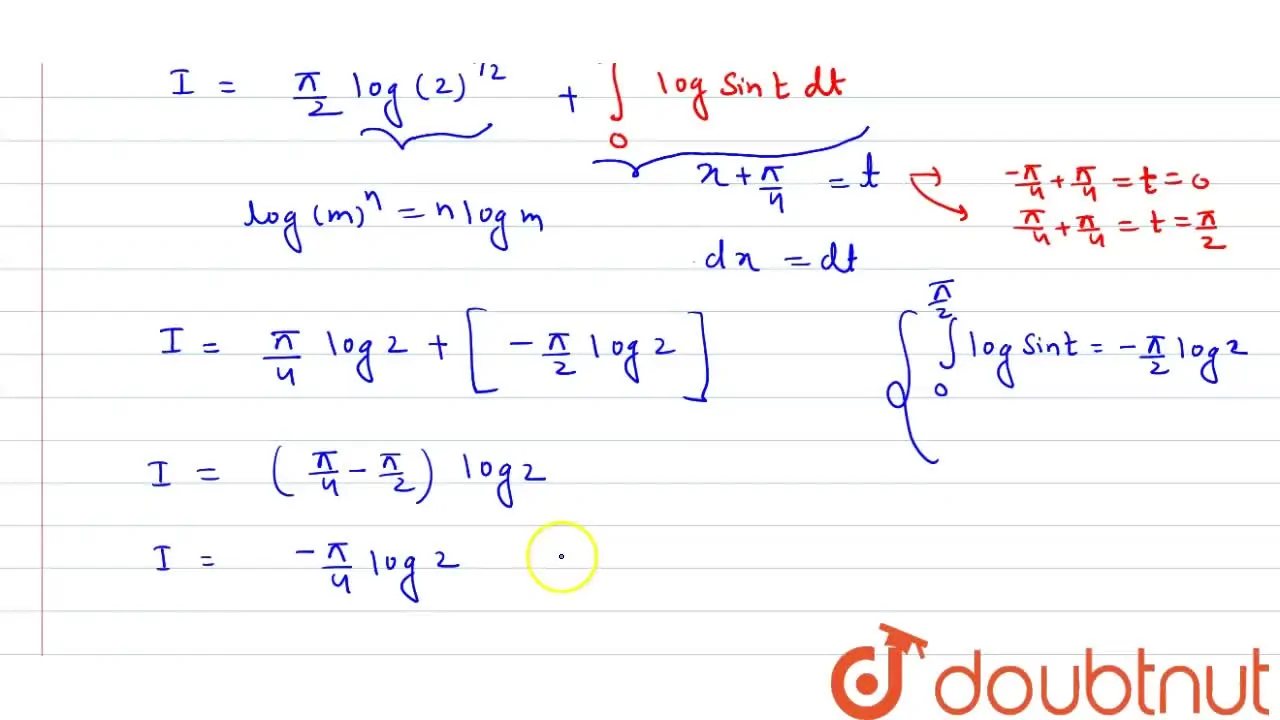
Prove that ∫π/4−π/4log(sinx+cosx) dx=−π4 log2.
Video Solution
Text Solution
The correct Answer is:N/a
Let I=∫π/4−π/4log(sinx+cos)dx.....(i)
I=∫π/4−π/4log{sin(π4−π4−x)+cos(π4−π74−x)}dx
=∫π/3−π/4log{sin(−x)+cos(−x)}+dx
=∫π/4−π/4log(cosx−sinx)dx.....(ii)
From Eqs. (i) and (ii),
2I=∫π4−π/4logcos2xdx
2I=∫π/40logcos2xdx.....(iii)
[∵∫a−af(x)=2∫a0f(x),iff(−x)=f(x)]
Put 2x=t⇒dx=dt2
As x→0, then t→0
and x→π4, then t→π2
2I=12∫π/20logcostdt....(iv)
2I=12∫π/20logcos(π2−t)dt,[∵∫a0f(x)dx=∫a0f(a−x)dx]
⇒2I=12∫π/20logsintdx.........(v)
On adding Eqs. (iv) and (v), we get
4I=12∫π/20logsintcostdt
⇒4I=12∫π/20log'sin2t2dt
4I=12sinπ/20logsin2xdx−12∫π/20log2dx
⇒4I=12∫π/20logsin(π2−2x)dx−log2.π4
⇒4I=12∫π/20logcos2xdx−π4log2
⇒4I=∫π/40logcos2xdx−π4log2[∵∫2a0f(x)dx=2∫a0f(x)dx]
⇒4I=2I−π4log2 [from Eq. (iii)]
∴I=−π8log2=π8log(12)
|
Updated on:21/07/2023
Related Playlists
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTEGRALSEXERCISE - Objective Type12videos
INTEGRALS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTEGRALSEXERCISE - Fillers3videos
INTEGRALS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTEGRALSEXERCISE - Fillers3videos
INTEGRALS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - DIFFERENTIAL EQUATIONS EXERCISE - Objective1videos
DIFFERENTIAL EQUATIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Fillers18videos
INVERSE TRIGONOMETRIC FUNCTIONS
Similar Questions
Evaluate: ∫π/4−π/4log(sinx+cosx)dx
View SolutionEvaluate: ∫π/2−π/2log(sinx+cosx)dx
View SolutionProve that : ∫3π/4π/411+cosxdx=2
View SolutionProve that: ∫π/20sinxsinx+cosxddx=π4
View SolutionEvaluate: ∫nπ−π4−π4|sinx+cosx|dx
View SolutionEvaluate: ∫nπ−π4−π4|sinx+cosx|dx
View SolutionProve that: ∫π/20log(sinx)dx=∫π/20log(cosx)dx=−π2log2
View SolutionProve that : ∫π/20xsinx+cosxdx=π4√2log∣∣∣√2+1√2−1∣∣∣
View Solution∫π2−π2cosxdx
View Solutionshow that ∫π/20(sinx−cosx)log(sinx+cosx)dx=0
View Solution