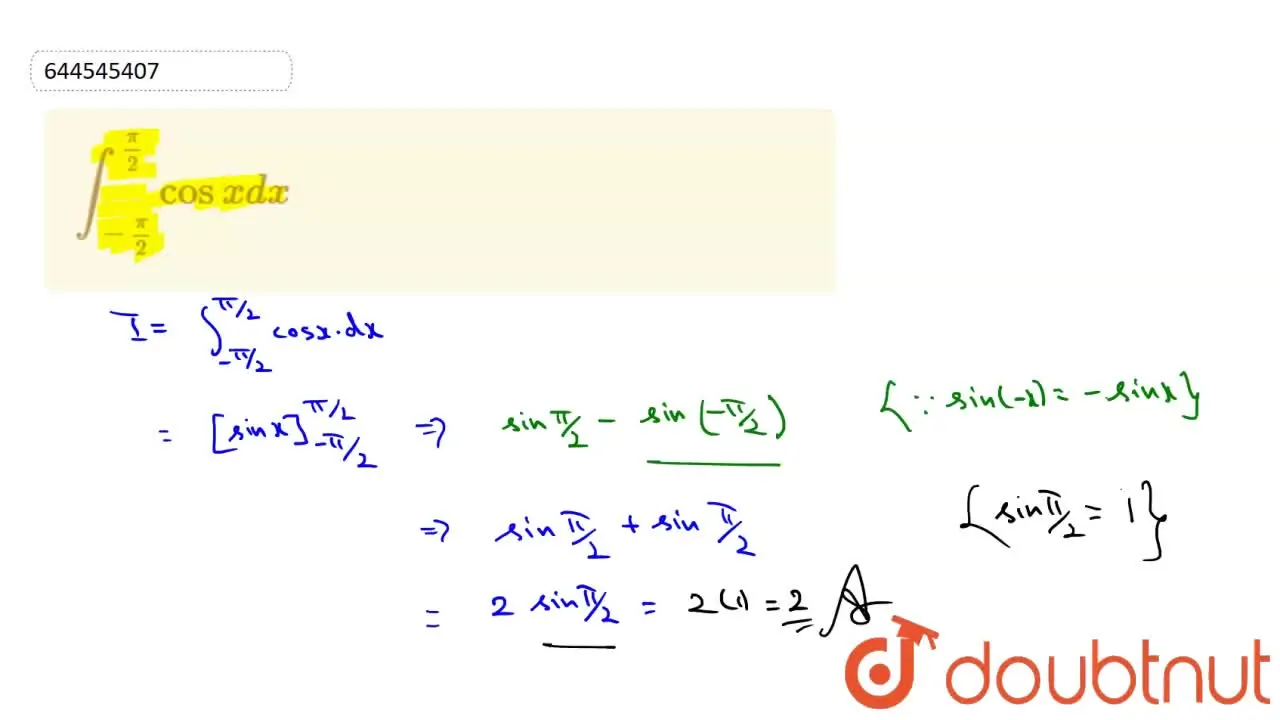
∫π2−π2cosxdx
Video Solution
Text Solution
−π2∫π2cosxdx=0π2∫0cosxdx=2 ( :' cos x is even function)
|
Updated on:21/07/2023
Related Playlists
- BOOK - RESONANCE ENGLISHCHAPTER - DEFINITE INTEGRATION & ITS APPLICATION EXERCISE - Self practive problem106videos
DEFINITE INTEGRATION & ITS APPLICATION
- BOOK - RESONANCE ENGLISHCHAPTER - DEFINITE INTEGRATION & ITS APPLICATION EXERCISE - Exercise 164videos
DEFINITE INTEGRATION & ITS APPLICATION
- BOOK - RESONANCE ENGLISHCHAPTER - COMBINATORICSEXERCISE - Exercise-2 (Part-II: Previously Asked Question of RMO)5videos
COMBINATORICS
- BOOK - RESONANCE ENGLISHCHAPTER - DPPEXERCISE - QUESTION656videos
DPP
Similar Questions
Evaluate the following integrals
(i) ∫∞RGMmx2dx
(ii) ∫r2r1−kq1q2x2dx
(iii) ∫vuMvdv
(iv) ∫∞0x−1/2dx
(v) ∫π/20sinxdx
(vi) ∫π/20cosxdx
(vii) ∫π/2−π/2cosxdxView SolutionEvaluate : ∫π2−π2cosx1+exdx
View SolutionEvaluate: ∫π/2−π/2cosx1+exdx
View SolutionEvaluate: ∫π/2−π/2x2cosx1+exdx
View SolutionEvaluate : ∫π2−π2sin2xdx
View SolutionEvaluate: ∫π/2−π/2sin7xdx
View SolutionEvaluate: ∫π/2−π/2|sinx|dx
View Solution∫π2−π2|sinx|dx
View SolutionThe value of ∫20π−20π|sinx|[sinx]dx is (where [.] denotes greatest integer function)
View SolutionEvaluate: ∫π/2−π/2log(sinx+cosx)dx
View Solution