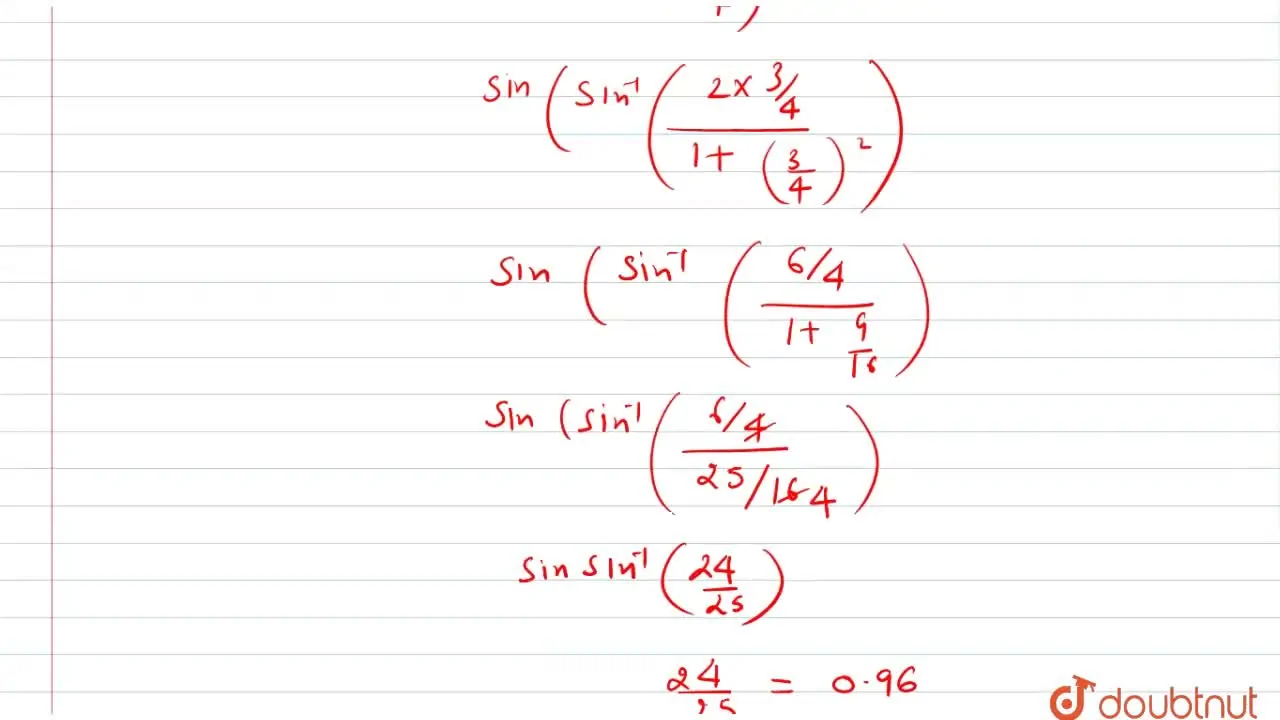The value of sin[2tan−1(0.75)] is
A
0.75
B
1.5
C
0.96
D
sin1.5
Video Solution
Text Solution
The correct Answer is:C
We have, sin[2tan−1(0.75)]=sin(2tan−1'34),[∵0.75=75100=34]
=sin⎛⎝sin−1'2.341+916⎞⎠=sin[sin−1'3/225/16]
=sin[sin−1(4850)]=sin[sin−1(2425)]=2425=0.96
|
Updated on:21/07/2023
Related Playlists
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Fillers18videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Fillers18videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTEGRALSEXERCISE - Fillers3videos
INTEGRALS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LINEAR PROGRAMMINGEXERCISE - TRUE/FALSE2videos
LINEAR PROGRAMMING
Similar Questions
The value of sin(2 tan−1(0.75)) is equal to (a) 0.75 (b) 1.5 (c) 0.96 (d) sin−11.5
View SolutionThe value of tan75
View SolutionThe value of tan75∘=
View SolutionThe value of 2sin15∘.cos75∘
View SolutionThe value of tan75∘−cot75∘ is equal to
View SolutionIf (x−1)(x2+1)>0, then find the value of sin(12tan−1.2x1−x2−tan−1x)
View SolutionIf (x−1)(x2+1)>0, then find the value of sin(12tan−1.2x1−x2−tan−1x)
View SolutionFind the value of: sin750
View SolutionThe value of sin2750−sin2150 is a.1/2 b. √3/2 c. 1 d. 0
View SolutionFind the value of expression: sin(2tan−1(13))+cos(tan−12√2)
View Solution