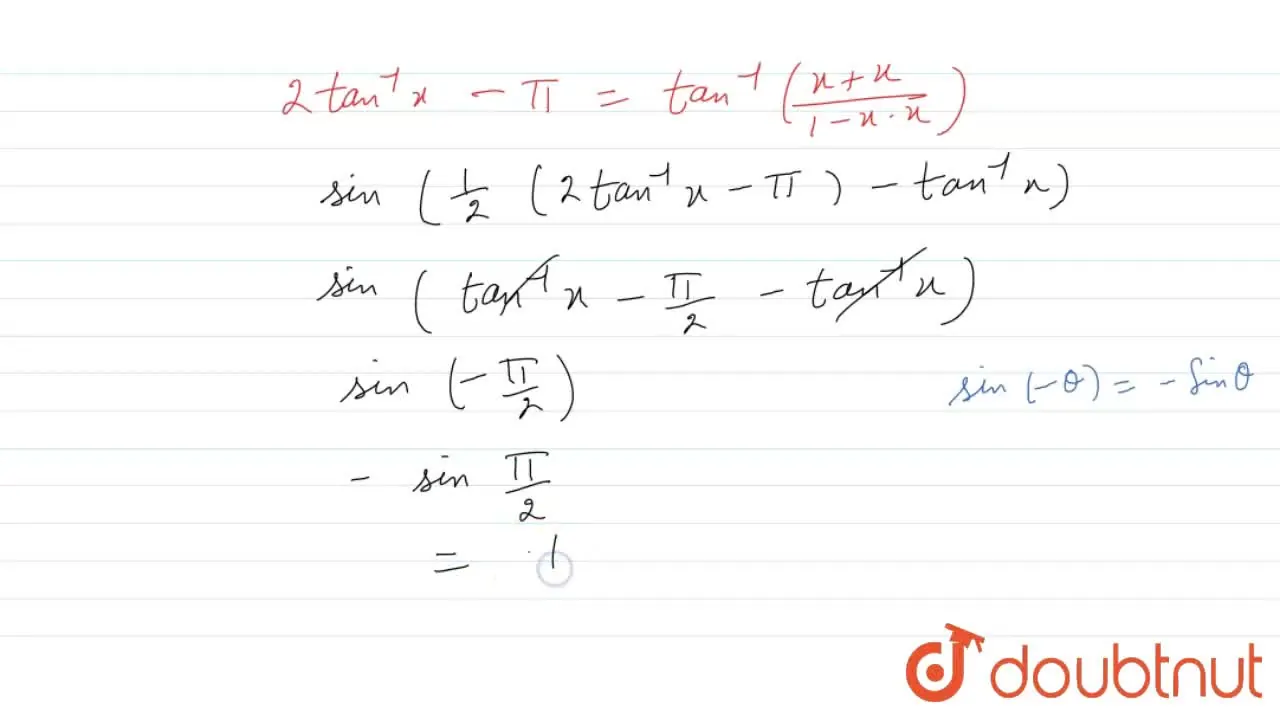
If (x−1)(x2+1)>0, then find the value of sin(12tan−1.2x1−x2−tan−1x)
Video Solution
Text Solution
(x−1)(x2+1)>0
∴Sin[12tan−1(2x1−x2)−tan−1x]
=sin[12(−π+2tan−1x)−tan−1x]=sin(−π2)=−1
|
Updated on:21/07/2023
Similar Questions
The value of ∫10tan−1(2x−11+x−x2)dx is
View SolutionIf tan−1x=θ, find the value of sin−1.2x1+x2
View SolutionIf x>y>0, then find the value of tan−1.xy+tan−1[x+yx−y]
View SolutionIf x>1 , then write the value of sin−1(2x1+x2) in terms of tan−1x
View SolutionIf x∈(0,1), then find the value of tan−1(1−x22x)+cos−1(1−x21+x2)
View SolutionIf x<0 , then write the value of cos−1(1−x21+x2) in terms of tan−1x
View SolutionFind the value of tan−1(−x)−cot−1(−1x),x>0
View SolutionProve that sin−1(2x1+x2)=tan−1(2x1−x2)
View SolutionFind the derivative of tan−1(2x)1−x2w.r.tsin−1(2x)1+x2
View SolutionFind the value of tan−1(x−2x−1)+tan−1(x+2x+1)=π4
View Solution