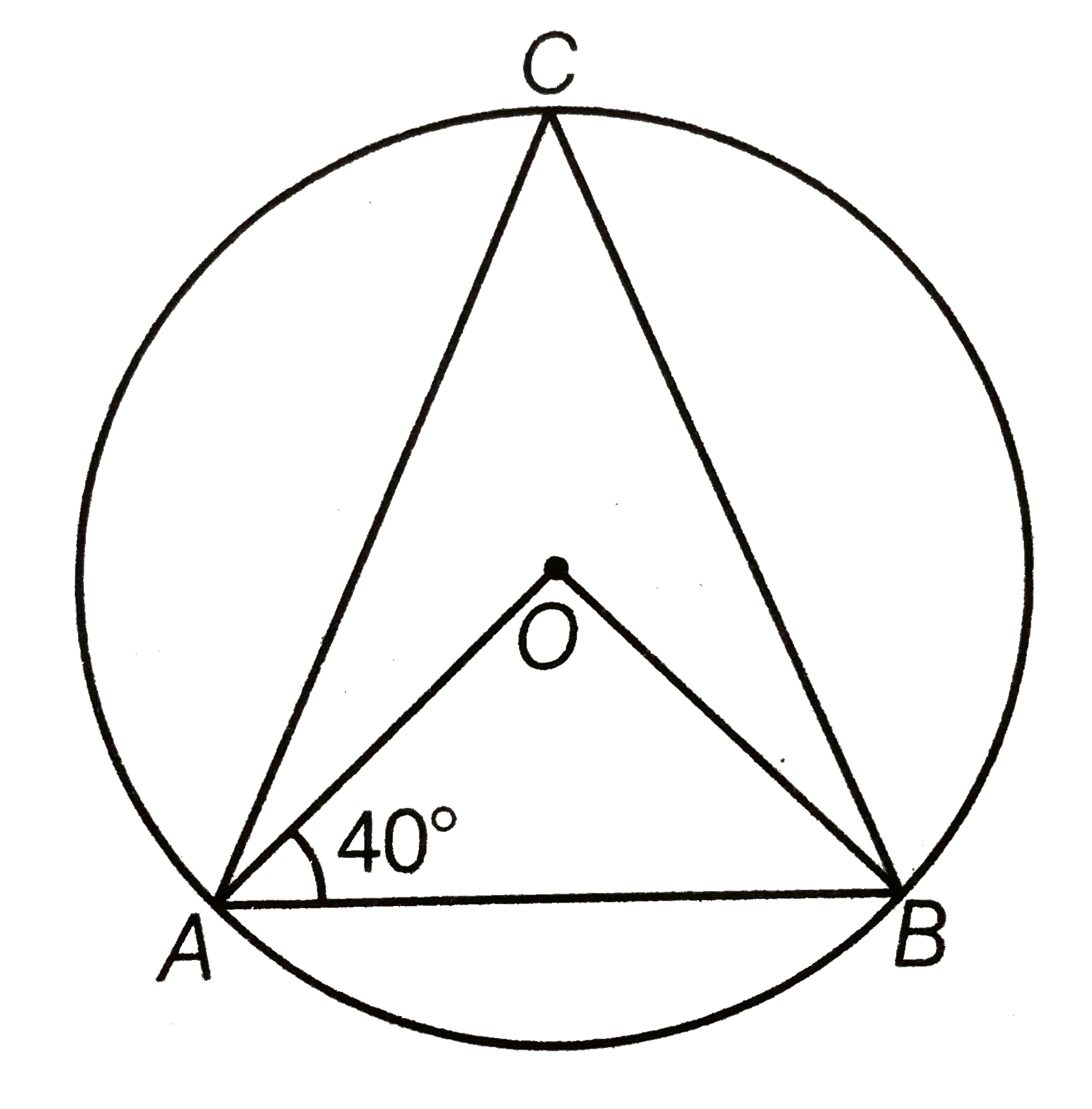
In figure, if ∠OAB=40∘,then∠ACB is equal to
A
50∘
B
40∘
C
60∘
D
70∘
Video Solution
Text Solution
The correct Answer is:A
In ΔOAB,OA=OB[both are the radius of a circle]
∴∠OAB=∠OBA⇒∠OBA=40∘
[angle opposite to equal sides are equal]
Also, ∠AOB+∠OBA+∠BAO=180∘
[by angle sum property of a triangle]
∴∠AOB+40∘+40∘=180∘
⇒∠AOB=180∘−80∘=100∘
We know that, in a circle, the angle subtended by an arc at the centre is twice the angle subtended by it at the remaining part of the circle.
∴∠AOB=2∠ACB
⇒100∘=2∠ACB
∴∠ACB=100∘2=50∘
|
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.2
10 Videos
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.3
20 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Exercise-9.4 Long Answer Type Questions
10 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions
5 Videos
Similar Questions
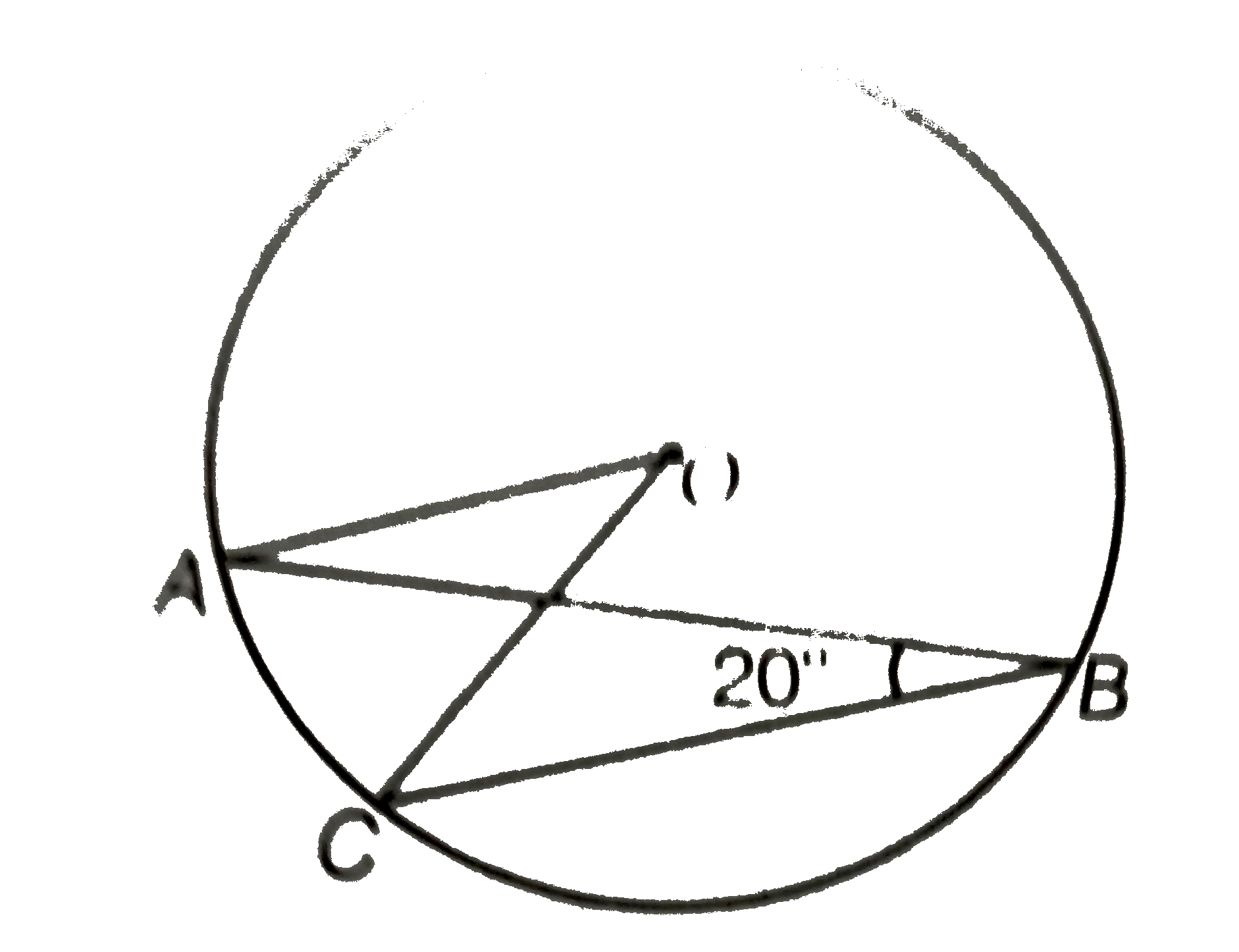
If figure, if∠ABC=20∘,then∠AOC is equal to
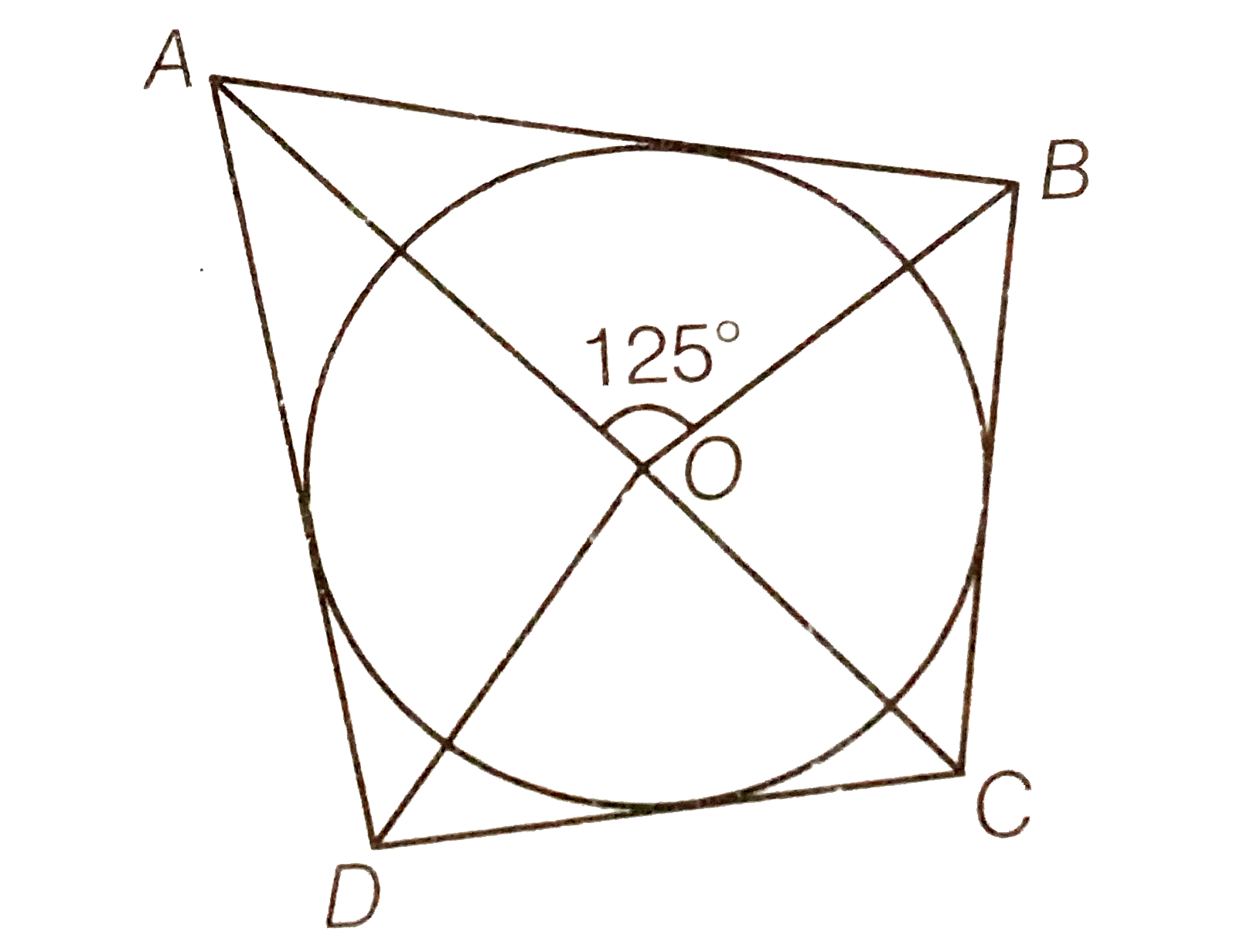
View SolutionIn figure, if ∠AOB=125∘,then∠COD is equal to
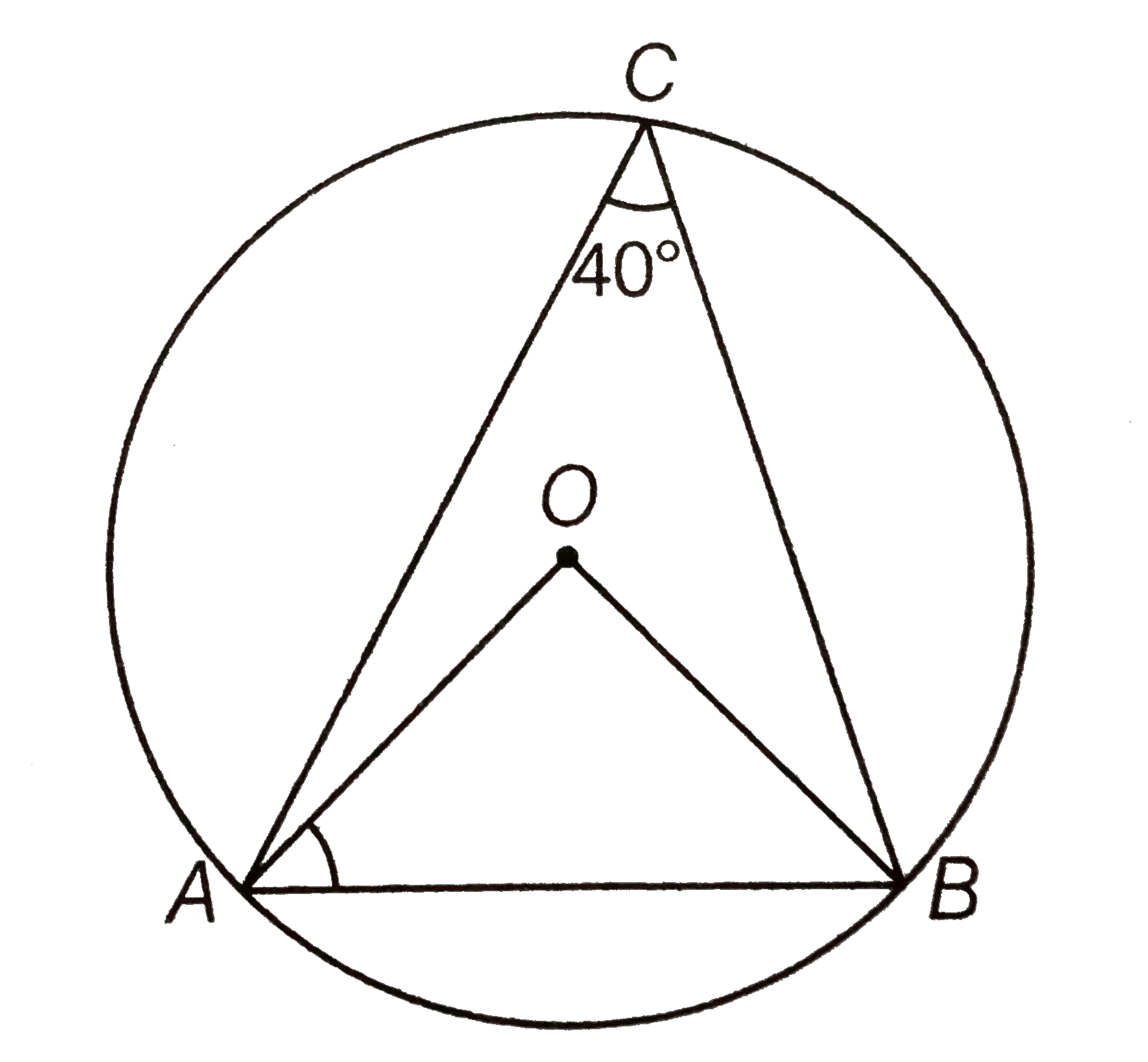
View SolutionIn figure, ∠ACB=40∘. Find ∠OAB.
View SolutionIn figure, if ∠DAB=60∘,∠ABD=50∘,then ∠ACB is equal to
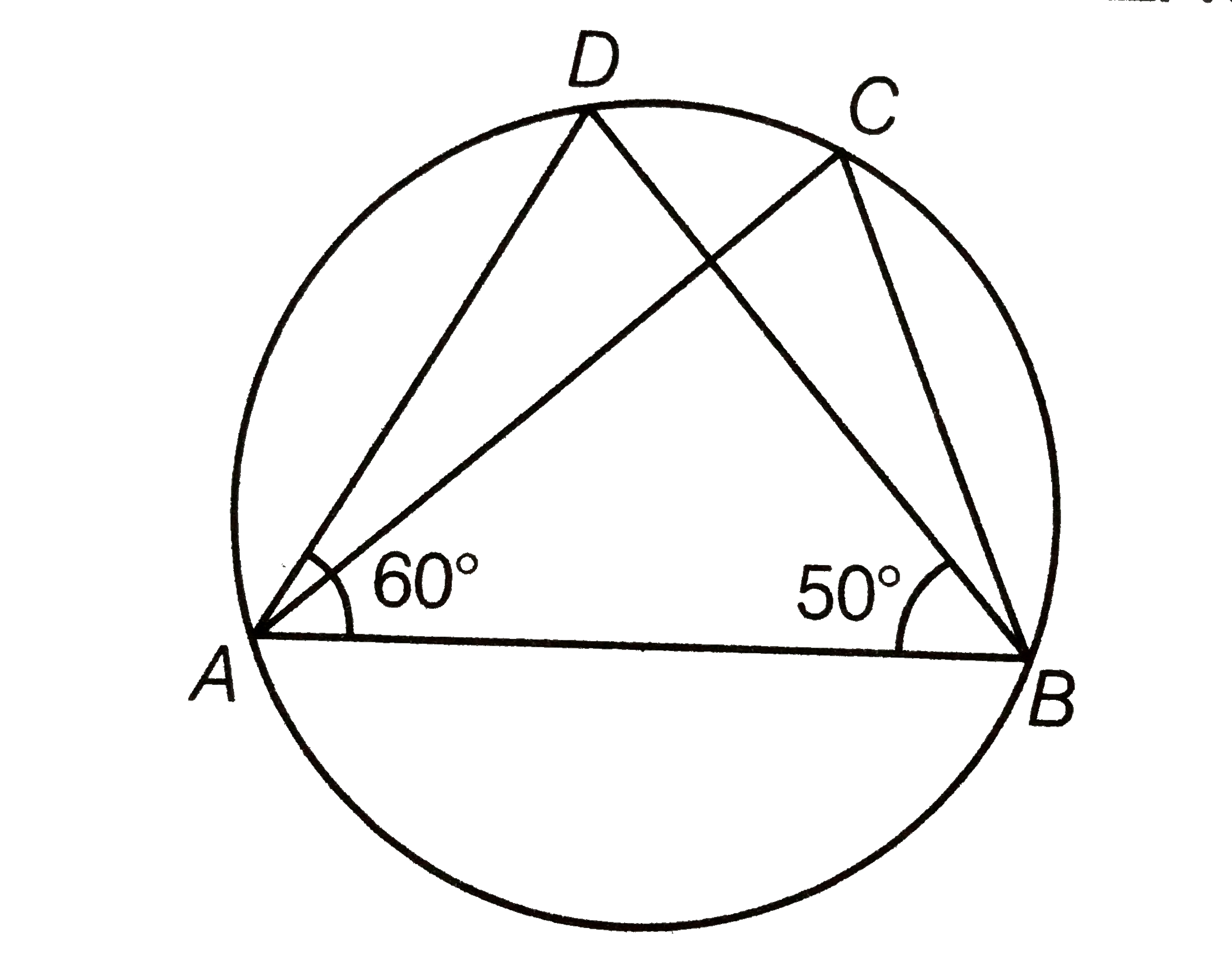
View SolutionIn figure, BC is a diameter of the circle and ∠BAO=60∘. Then,∠ADCis equal to
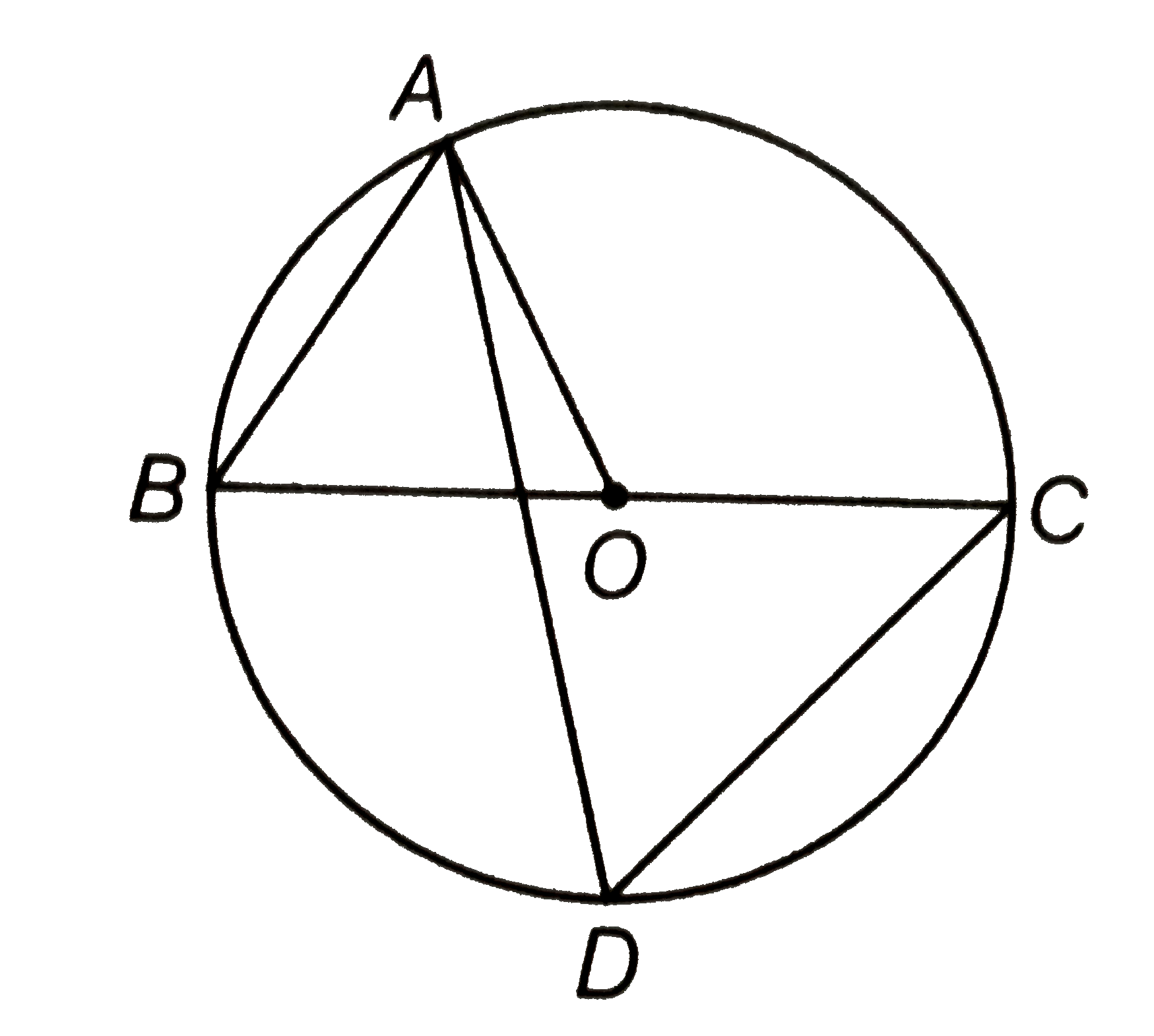
View SolutionIn figure, if PA and PB are tangents to the circle with centre O such that ∠APB=50∘, then ∠OAB is equal to
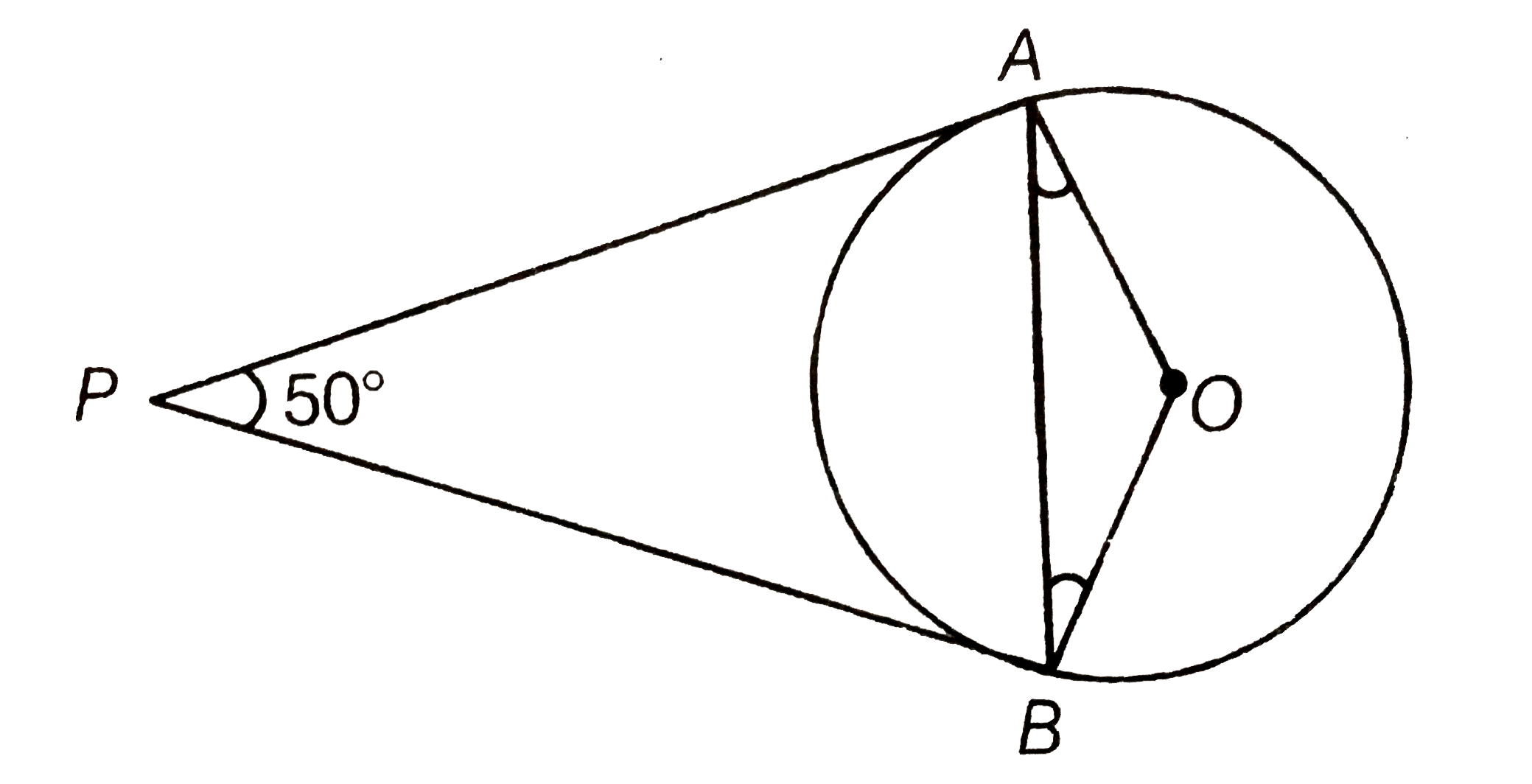
View SolutionIn figure, if ∠AOB=90∘and∠ABC=30∘, then∠CAO is equal to
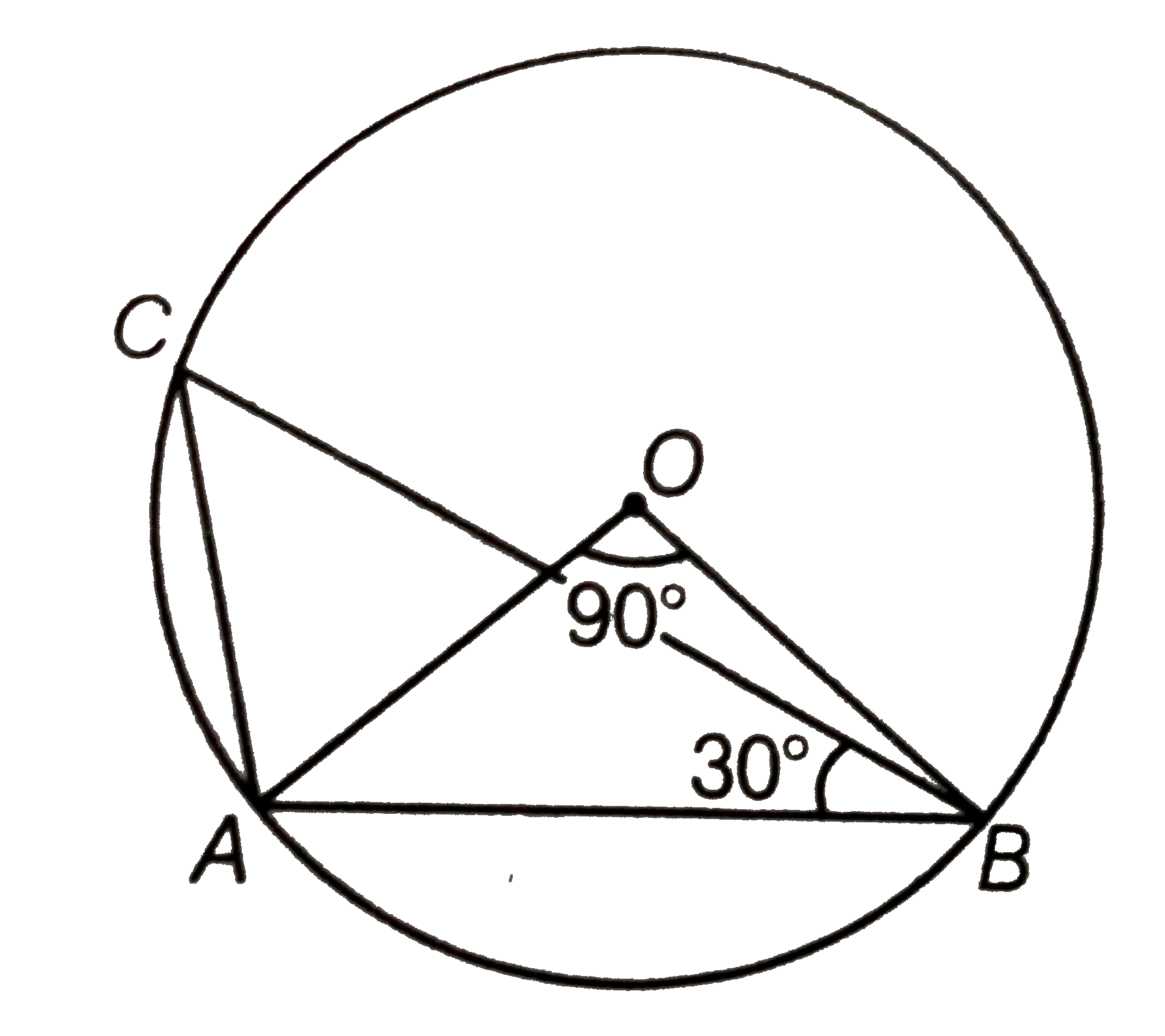
View SolutionIn Figure-2 PQ is tangent to the circle with centre at O , at the point B. If ∠AOB = 100∘ , then ∠ABP is equal to
(A) 50∘
(B) 40∘
(C) 60∘
(D) 80∘
View SolutionIn figure, ∠OAB=30∘and∠OCB=57∘. Find ∠BOCand∠AOC.
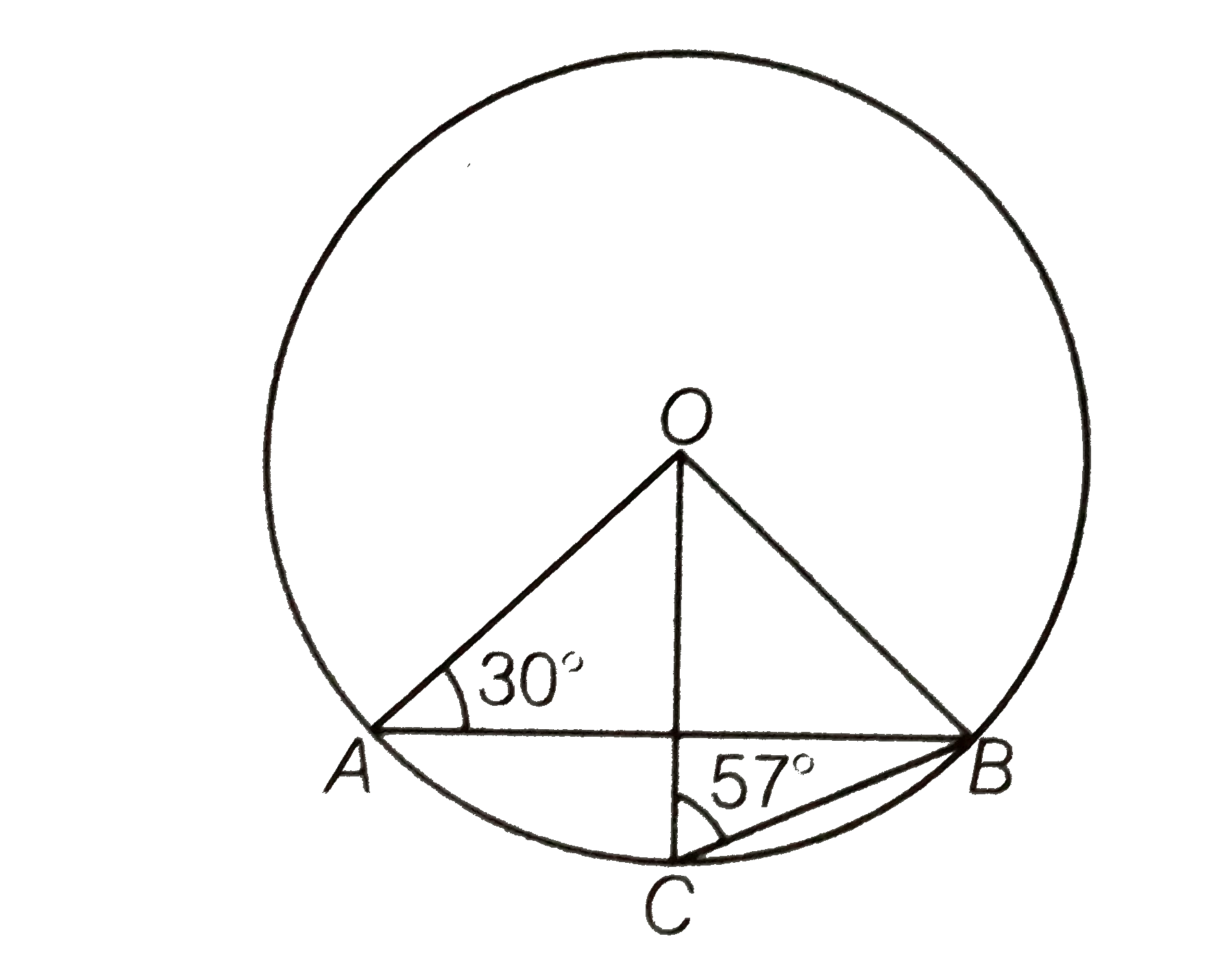
View SolutionIn the given figure, ∠OAB=30∘and∠OCB=57∘, find ∠BOCand∠AOC

View Solution
