Consider a cycle tyre being filled with air by a pump. Let V be the volume of the tyre (fixed) and at each stroke of the pump ΔV(<<V) of air is transferred to the tube adiabatically. What is the work done when the pressure in the tube is increased from p1 to p2 ?
To find the work done when the pressure in the tube is increased from p1 to p2 during the adiabatic filling of a cycle tyre, we can follow these steps:
Step 1: Understand the Adiabatic Process
In an adiabatic process, the relationship between pressure and volume is given by:
PVγ=constant
where γ is the adiabatic index (ratio of specific heats).
Step 2: Relate Changes in Pressure and Volume
When a small volume ΔV of air is added, the change in pressure ΔP can be related to the change in volume:
P(V+ΔV)γ=(P+ΔP)Vγ
This can be rearranged to express the relationship between ΔP and ΔV.
Step 3: Expand Using Binomial Approximation
Using the binomial expansion for small changes, we can write:
(V+ΔV)γ≈Vγ+γVγ−1ΔV
Substituting this into our equation gives:
P(Vγ+γVγ−1ΔV)=(P+ΔP)Vγ
This simplifies to:
PγVγ−1ΔV=ΔPVγ
Step 4: Solve for ΔP
From the above equation, we can isolate ΔP:
ΔP=PγΔVV
Step 5: Work Done in Terms of Pressure Change
The work done W during this process can be expressed as:
W=∫p2p1VdP
Substituting dP in terms of ΔP:
dP=PγVdV
Thus, we can rewrite the work done as:
W=∫p2p1V(PγV)dV
Step 6: Integrate to Find Work Done
Since V is constant during the process, we can take it out of the integral:
W=Vγ∫p2p1dP
Evaluating the integral gives:
W=Vγ(p2−p1)
Final Result
Thus, the work done when the pressure in the tube is increased from p1 to p2 is:
W=Vγ(p2−p1)
In an adiabatic process, the relationship between pressure and volume is given by:
PVγ=constant
where γ is the adiabatic index (ratio of specific heats).
When a small volume ΔV of air is added, the change in pressure ΔP can be related to the change in volume:
P(V+ΔV)γ=(P+ΔP)Vγ
This can be rearranged to express the relationship between ΔP and ΔV.
Using the binomial expansion for small changes, we can write:
(V+ΔV)γ≈Vγ+γVγ−1ΔV
Substituting this into our equation gives:
P(Vγ+γVγ−1ΔV)=(P+ΔP)Vγ
This simplifies to:
PγVγ−1ΔV=ΔPVγ
From the above equation, we can isolate ΔP:
ΔP=PγΔVV
The work done W during this process can be expressed as:
W=∫p2p1VdP
Substituting dP in terms of ΔP:
dP=PγVdV
Thus, we can rewrite the work done as:
W=∫p2p1V(PγV)dV
Since V is constant during the process, we can take it out of the integral:
W=Vγ∫p2p1dP
Evaluating the integral gives:
W=Vγ(p2−p1)
Thus, the work done when the pressure in the tube is increased from p1 to p2 is:
W=Vγ(p2−p1)
Topper's Solved these Questions
- THERMODYNAMICS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - THERMODYNAMICS EXERCISE - LONG ANSWER TYPE QUESTIONS
5 Videos
- THERMODYNAMICS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - THERMODYNAMICS EXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
5 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Very short Answer type Questions
15 Videos
- UNITS AND MEASUREMENTS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - UNITS AND MEASUREMENTS EXERCISE - Long Answer Type Questions
9 Videos
Similar Questions
When air is pumped into a cycle tyre the volume and pressure of the air in the tyre both are increased. What about Bouyle's law in this case?
View SolutionConsider a cylindrical tube closed at one end and fitted with a conducting, movable piston at the other end. A cathode is fixed in the tube near the closed end and an anode is fixed with the piston. A gas is filled in the tube at pressure p. Using Paschen equation V = f(pd), show that the sparking potential does not change as the piston is slowly moved in or out. Assume that the temperature does not change in the process.
View SolutionA cycle followed by an engine (made of one mole of perfect gas in a cylinder with a piston) is shown in figure.
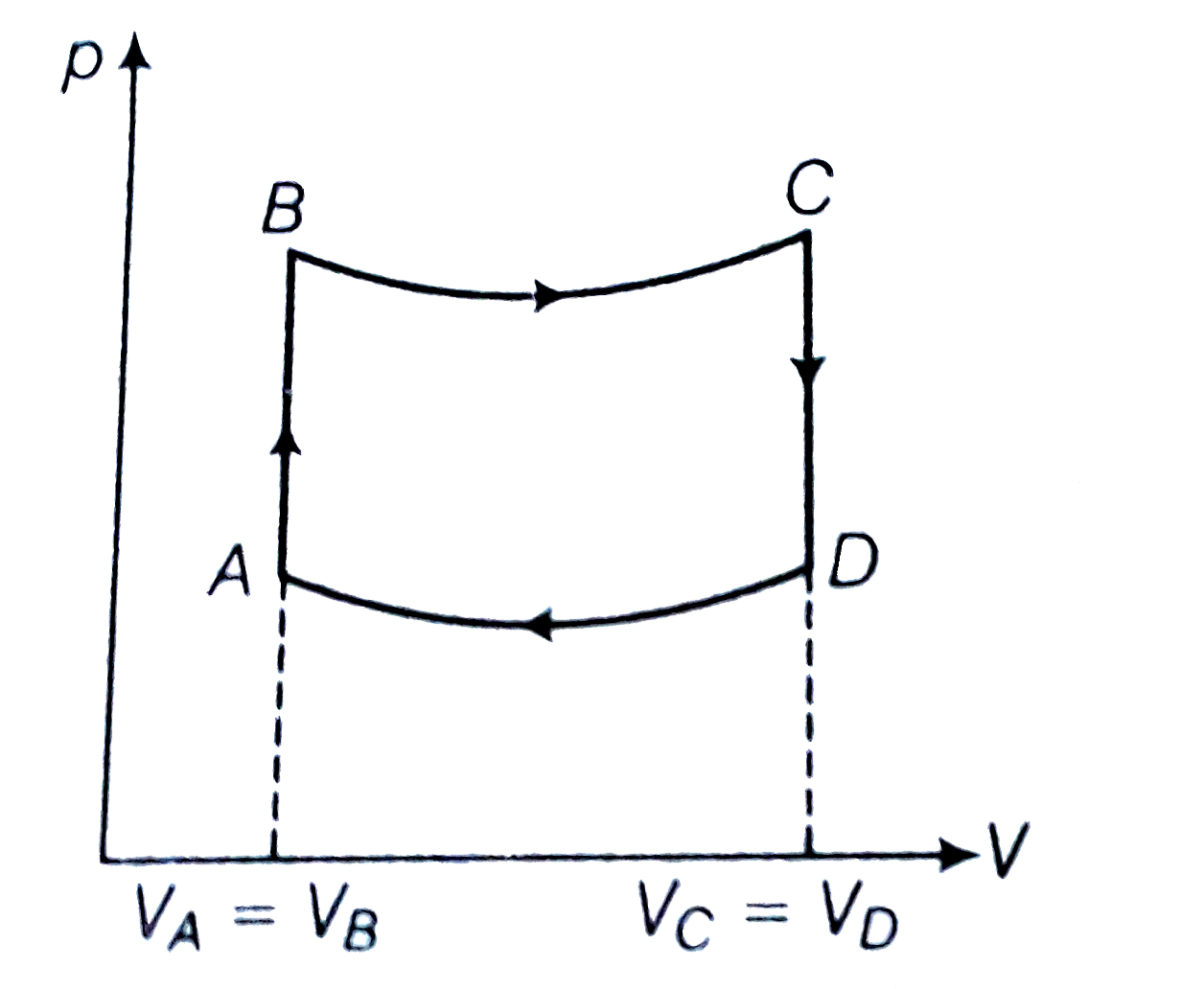
A to B volume constant, B to C adiabatic, C to D volume constant and D to A adiabatic
VC=VD=2VA=2VB
(a) In which part of the cycle heat is supplied to the engine form outside?
(b) In which part of the cycle heat is being given to the surrounding by the engine ?
(c ) What is the work done by the engine in one cycle ? Write your answer in term of pA,pB,VA ?
(d) What is the efficiency of the engine ?
(γ=53 for the gas), (CV=32R for one mole )
View SolutionIn a tyre of "Ferrari" car of Mr. Obama, a tube having a volume of 12.3 litres is filled with air at a pressure of 4 atm at 300 K.Due to travelling, the temperature of the tube and air inside it raised to 360 K and pressure reduced to 3.6 atm in 20 minutes. If the porosity (number of pores per unit area) of the tube material is 5×103 pores/cm2 and each pore can transfer air from inside to outside of the tube with the rate of 6.023×108 molecules per minute.Calculate the total surface area (m2) of the tube.(R=0.082 Lt-atm/mole-K)
Give your answer divide by 100.
View SolutionA cylindrical tube of uniform cross-sectional area A is fitted with two air tight frictionless position. The pistons are connected to each other by a metallic wire. Initially the pressure of the gas is po and temperature is To, atmospheric pressure is also po Now the temperature of the gas is increased to 2T0, the tension in the wire will be

View Solution
A cylindrical tube of uniform cross-sectional area A is fitted with two air tight frictionless pistons. The pistons are connected to each other by a metallic wire. Initially the pressure of the gas is P0 and temperature is T0, atmospheric pressure is also P0. Now the temperature of the gas is increased to 2T0, the tension in the wire will be
View SolutionThe volume of an ideal gas Vis filled in a cylinder fitted with a piston at pressure P: The volume of the gas is changed to 4V each time by three different processes : (i) Change at constant pressure (ii) Adiabatic change (iii) Isothermal changes. Show these changes on P-V diagram. State giving reason in which change the work done is maximum.
View Solutionshows a cylindrical tube of cross-sectional area A fitted with two frictionless pistons. The pistons are connected to each other by a metallic wire. Initially, the temperature of the gas is T0and its pressure is p0which equals the atmospheric pressure, (a)What is the tention in the wire?(b)What will be the tention if the temperature is increased to 2T_(0)?

View SolutionThe equation of a state of a gas is given by p(V−b)=nRT. If 1 mole of a gas is isothermally expanded from volume V and 2V, the work done during the process is
View SolutionAir is pumped into an automobile tyre's tube up to pressure pf 200kPa in the morning when the air temperature is 20∘C.During the day the temperature rises to 40∘C and the tube expand by 2%. Calculate the pressure of the air in the tube at this temperature.
View Solution
