Question

A particle is moving in a circular path of radius r. The displacement after half a circle would be

Options

A. zero
B. `pi`r
C. 2r
D. `2pi` r

Explanation

To solve the problem of finding the displacement of a particle moving in a circular path of radius $$r$$ after it has moved half a circle, we can follow these steps:

1. Understand the Circular Path:
- The particle is moving along a circular path with a radius \( r \). Let’s denote the center of the circle as point \( O \).

2. Identify Initial and Final Points:
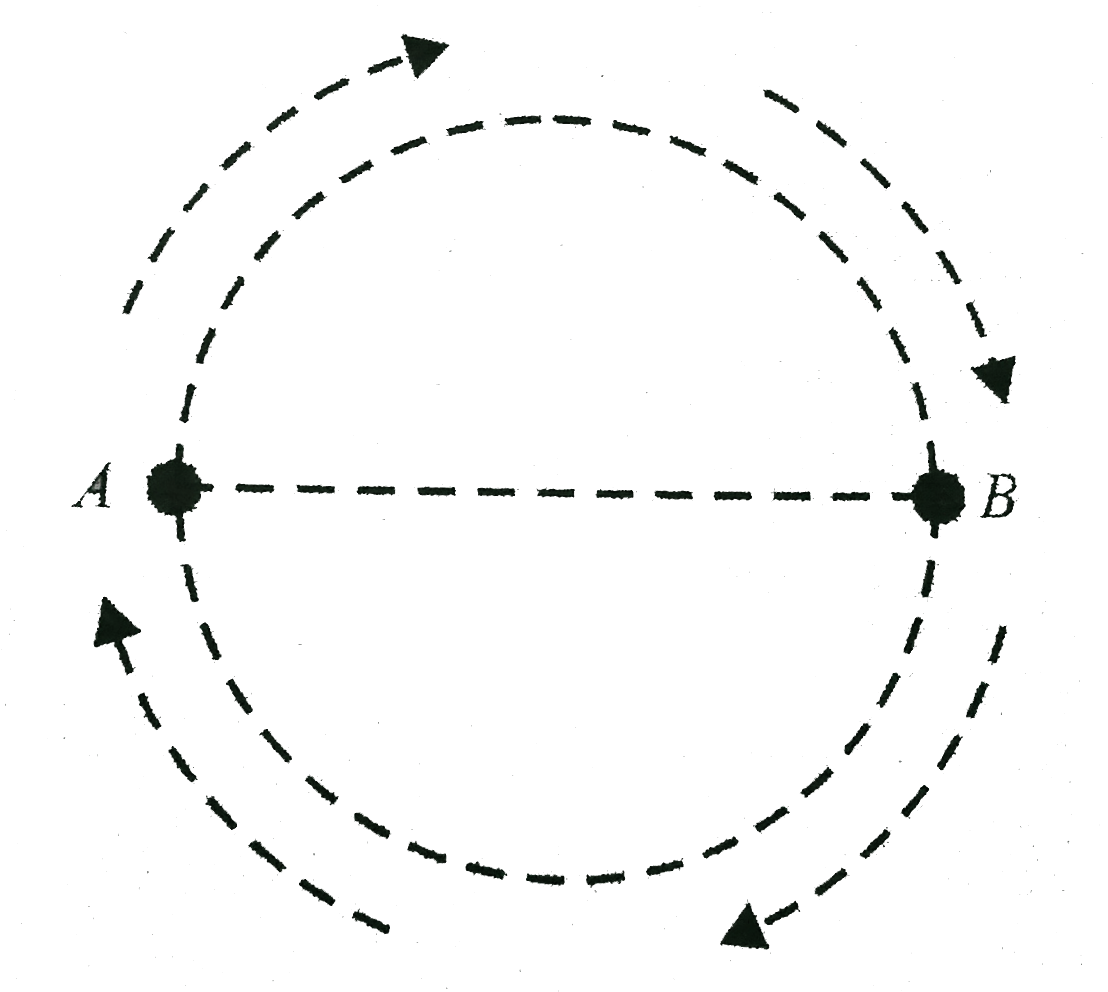
- Let the initial position of the particle be point \( A \) on the circle. After moving half a circle, the particle will reach point \( B \), which is directly opposite point \( A \) on the circle.

3. Draw the Diagram:
- Visualize or draw a circle with center \( O \). Mark point \( A \) on the circumference and point \( B \) directly opposite to \( A \).

4. Determine the Displacement:
- Displacement is defined as the shortest distance between the initial and final positions. In this case, the displacement will be the straight line distance from point \( A \) to point \( B \).

5. Calculate the Distance:
- The distance \( AB \) can be found by considering the triangle \( OAB \). The distance \( OA \) (radius) is \( r \) and the distance \( OB \) (radius) is also \( r \).
- The line segment \( AB \) forms the base of an isosceles triangle \( OAB \) where \( O \) is the vertex opposite the base \( AB \).

6. Use the Properties of the Circle:
- Since \( A \) and \( B \) are diametrically opposite, the length of \( AB \) can be calculated as the diameter of the circle. The diameter \( d \) is given by:
\( d = 2r \)
- Therefore, the displacement \( AB \) is equal to the diameter of the circle.

7. Final Result:
- The displacement after half a circle is:
\( \text{Displacement} = 2r \)

Conclusion:
Thus, the displacement of the particle after moving half a circle is \( 2r \).

---