How does the sign of the phase angle ϕ, by which the supply voltage leads the current in an LCR serices circuit, change as the supply frequency is gradually increased from very low to very high values ?
The correct Answer is:A
To analyze how the sign of the phase angle ϕ changes as the supply frequency in an LCR series circuit is gradually increased from very low to very high values, we can follow these steps:
Step 1: Understand the Phase Angle Formula
The phase angle ϕ in an LCR circuit is given by the equation:
tanϕ=XL−XCR
where:
- XL=2πfL (inductive reactance)
- XC=12πfC (capacitive reactance)
- R is the resistance.
Step 2: Analyze at Very Low Frequency
When the frequency f is very low:
- XL=2πfL becomes a small value.
- XC=12πfC becomes a very large value because it is inversely proportional to f.
Thus, we have:
tanϕ=XL−XCR≈small−largeR<0
This implies that ϕ<0 (the voltage lags behind the current).
Step 3: Analyze at Resonant Frequency
At the resonant frequency fr:
- At resonance, XL=XC, which leads to:
tanϕ=XL−XCR=0
Thus, ϕ=0 (the voltage and current are in phase).
Step 4: Analyze at Very High Frequency
When the frequency f is very high:
- XL=2πfL becomes a very large value.
- XC=12πfC becomes a very small value.
Thus, we have:
tanϕ=XL−XCR≈large−smallR>0
This implies that ϕ>0 (the voltage leads the current).
Conclusion
As the supply frequency increases from very low to very high:
- At very low frequency: ϕ<0 (voltage lags current)
- At resonance: ϕ=0 (voltage and current are in phase)
- At very high frequency: ϕ>0 (voltage leads current)
The phase angle ϕ in an LCR circuit is given by the equation:
tanϕ=XL−XCR
where:
- XL=2πfL (inductive reactance)
- XC=12πfC (capacitive reactance)
- R is the resistance.
When the frequency f is very low:
- XL=2πfL becomes a small value.
- XC=12πfC becomes a very large value because it is inversely proportional to f.
tanϕ=XL−XCR≈small−largeR<0
This implies that ϕ<0 (the voltage lags behind the current).
At the resonant frequency fr:
- At resonance, XL=XC, which leads to:
tanϕ=XL−XCR=0
Thus, ϕ=0 (the voltage and current are in phase).
When the frequency f is very high:
- XL=2πfL becomes a very large value.
- XC=12πfC becomes a very small value.
tanϕ=XL−XCR≈large−smallR>0
This implies that ϕ>0 (the voltage leads the current).
As the supply frequency increases from very low to very high:
- At very low frequency: ϕ<0 (voltage lags current)
- At resonance: ϕ=0 (voltage and current are in phase)
- At very high frequency: ϕ>0 (voltage leads current)
Topper's Solved these Questions
- ALTERNATING CURRENTBook:NCERT EXEMPLAR ENGLISHChapter:ALTERNATING CURRENTExercise:Short Answer types questions
Explore 1 Video
- ALTERNATING CURRENTBook:NCERT EXEMPLAR ENGLISHChapter:ALTERNATING CURRENTExercise:Long Answe types Questions
Explore 5 Videos
- ALTERNATING CURRENTBook:NCERT EXEMPLAR ENGLISHChapter:ALTERNATING CURRENTExercise:Long Answe types Questions
Explore 5 Videos
- ATOMSBook:NCERT EXEMPLAR ENGLISHChapter:ATOMSExercise:Long Answer Type
Explore 6 Videos
Similar Questions
(a) Deriver and expression for the average power consumed in a series LCR circuit connected to a.c., source in which the phase different between the voltage and the current in the circuit is ϕ.
(b) Define the quality factor in an a.c. circuit. Why should the quality factor have high value in receiving circuits ? Name the factors on which it depends.
View SolutionHow do the energy gaps between successive electron energy levels in an atom very from low to high n values ?
View Solution
Knowledge Check
In series LCR circuit, the phase angle between supply voltage and current is
Atanϕ=XL−XCRBtanϕ=RXL−XCCtanϕ=RXL+XCDtanϕ=XL+XCR
(i) Find the value of the phase difference between the current and the voltage in the series LCR circuit shown below. Which one leads in phase : current or voltage ?
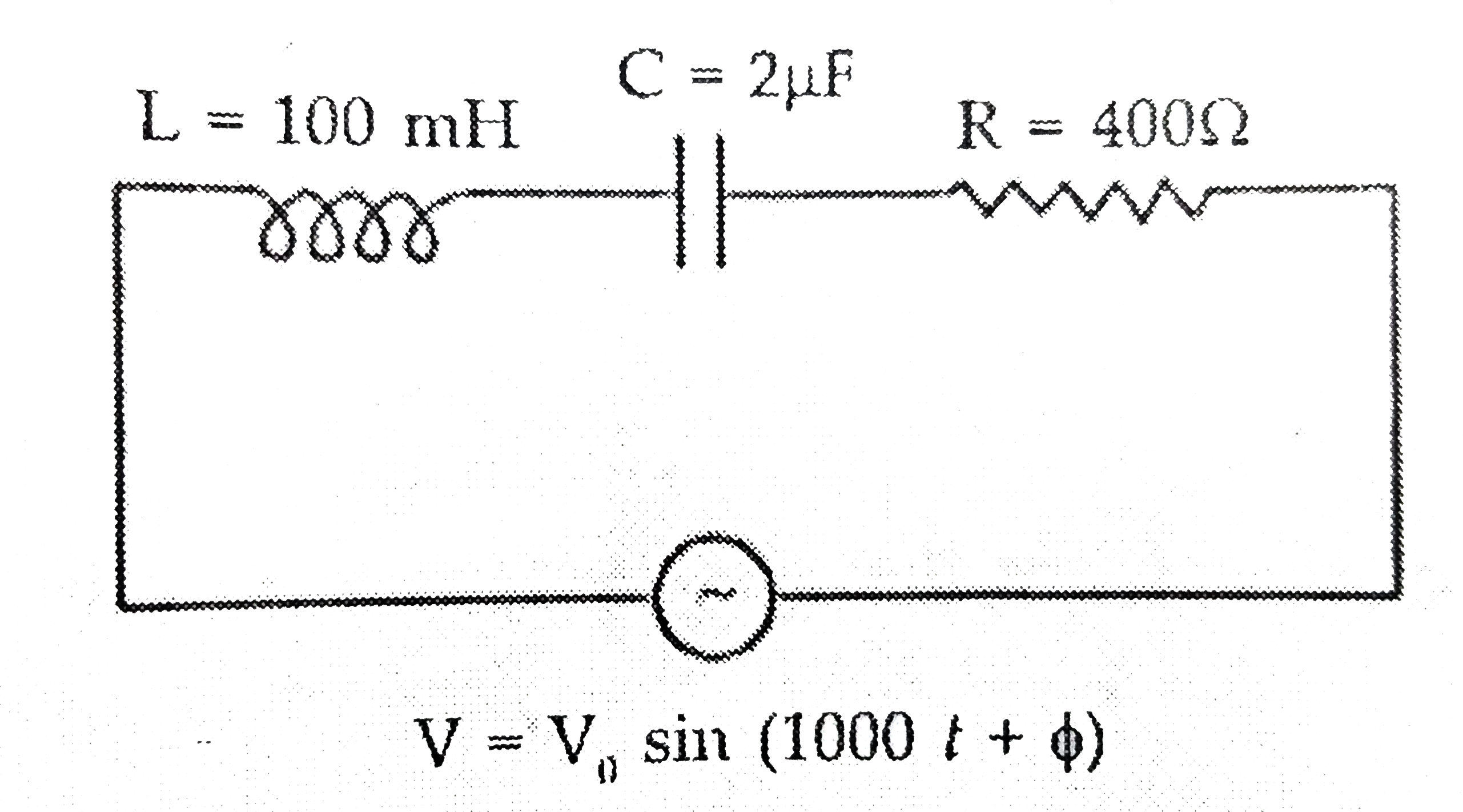
(ii) What making any other change, find the value of the additional capacitor C, to be connected in parallel with the capacitor C, in order to make the power factor of the circuit unity.
View SolutionLoudness is psychological response to a sound. It depends on both the intensity and the frequency of the sound. As a rule of thumb, a doubling in loudness is approximately associated with an increase in sound level of 10 dB. (this rule of thumb is relatively inaccurate at very low or very high frequencis.) If the loudness of the machines in illustration 6. is to be doubled, how many machines at the same distance from the worker must be running?
View SolutionLength AB in the figure shown in 5 m. The body is released from A. Friction is sufficient for pure rolling to take place.

In the above case suppose we have four bodies ring, disc, solid sphere and hollow sphere. The angle θ is now gradually increased. Which body will start slipping very fast. All the bodies have same mass and radius. Coefficient of friction is also same ?
View SolutionIn a series RLC circuit, if the frequency is increased to a very large value, what value does the phase angle between current and voltage approach ?
View SolutionThe maximum values of the alternating voltages and current are 400V and 20A respectively in a circuit connected to 50Hz supply and these quantities are sinusoidal. The instantaneous values of the voltage and current are 200√2V and 10A, respectively at t=0, both are increasing positively.
(a) Write down the expression for voltage and current at time t.
(b) Determine the power consumed in the circuit.
View SolutionA 300Ω resistor, a 0.250H inductor, and a 8.00μF capacitor are in series with an Ac with voltage amplitude 120V and angular frequency 400rad/B.
(a) What is the current amplitude?
(b) Wheat is the phase angle of the source voltage with respect to the current? Does the source Lag, or lead the current?
(c) What are the voltage amplitudes across the resistor, inductor, and capacitor ?
View SolutionA student focuses the image of a well illuminated distant object on a screen using a convex lens. After that the gradually moves the object towards the lens and each time focuses its image on the screen by adjucting the lens.
(a) In which direction -towards the screen or away from the screen, does he move the lens ?
(b)What happens to the size of the image-does it decrease or increase ?
(c) What happens to the image on the screen when he moves the object very close to the lens ?
View Solution