Question

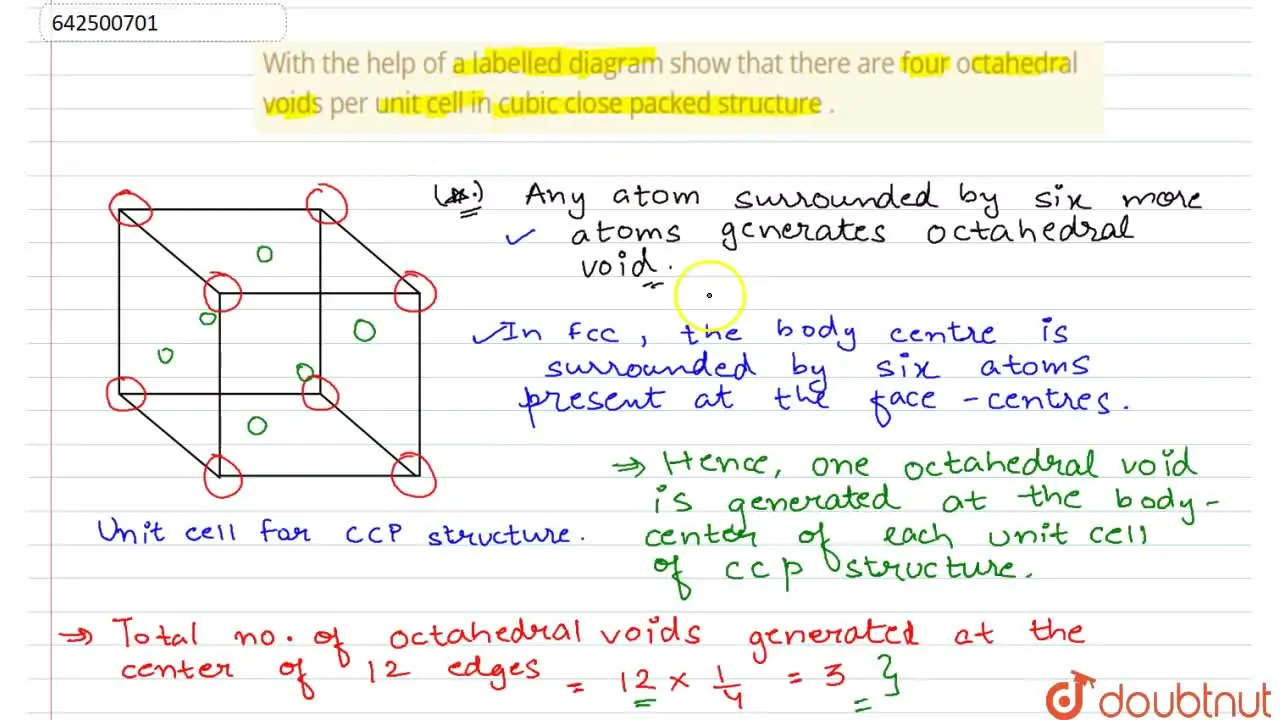
With the help of a labelled diagram show that there are four octahedral voids per unit cell in cubic close packed structure .

Explanation

To show that there are four octahedral voids per unit cell in a cubic close-packed (CCP) structure, we can follow these steps:

Step 1: Draw the Unit Cell
- Start by drawing a cube to represent the unit cell of the cubic close-packed structure.
- Label the corners of the cube where the atoms are located. There are 8 corner atoms in total.

Step 2: Add Face-Centered Atoms
- Next, draw the atoms located at the center of each face of the cube. There are 6 face-centered atoms (one on each face).

Step 3: Identify Octahedral Voids
- Understand that an octahedral void is formed when an atom is surrounded by six other atoms.
- In the CCP structure, there are two main locations for octahedral voids:
1. At the Body Center: There is one octahedral void at the center of the cube (body center).
2. At the Edge Centers: There are octahedral voids located at the center of each of the 12 edges of the cube.

Step 4: Calculate the Contribution from the Body Center
- Since there is one octahedral void at the body center of the cube, we can say:
- Contribution from body center = 1 void.

Step 5: Calculate the Contribution from the Edge Centers
- Each edge of the cube has an octahedral void at its center. Since there are 12 edges in a cube, we need to consider how many of these voids belong to one unit cell.
- Each octahedral void at the edge center is shared by four adjacent unit cells. Therefore, the contribution from the edge centers can be calculated as:
- Total edge voids = 12 edges × (1/4) contribution per edge = 3 voids.

Step 6: Total Number of Octahedral Voids
- Now, add the contributions from both locations:
- Total octahedral voids = Contribution from body center + Contribution from edge centers
- Total octahedral voids = 1 + 3 = 4.

Conclusion
- Thus, we conclude that there are a total of four octahedral voids per unit cell in a cubic close-packed structure.

Labeled Diagram
- Ensure to label the body center and the edge centers in your diagram to clearly indicate where the octahedral voids are located.

---