Match the following
WordNumber of permutations by using all the lettersI.INTER(A)6!II.FLOWER(b)7!III.VICTORY(c)5!
The correct match is Solution in Telugu
a,b,c
b,c, a
c, b, a
c, a, b
The correct Answer is:C
Step by step video, text & image solution for Match the following {:("Word","Number of permutations by using all the letters"),(I."INTER",(A)6!),(II."FLOWER",(b)7!),(III.VICTORY",(c)5!):} The correct match is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PERMUTATIONS & COMBINATIONSBook:AAKASH SERIESChapter:PERMUTATIONS & COMBINATIONSExercise:EXERCISE-II
Explore 229 Videos
- PARTIAL FRACTIONSBook:AAKASH SERIESChapter:PARTIAL FRACTIONSExercise:PRACTICE EXERCISE
Explore 31 Videos
- PROBABILITYBook:AAKASH SERIESChapter:PROBABILITYExercise:Practise Exercise
Explore 165 Videos
Similar Questions
1+1.1!+2.2!+3.3!+.......+n.n! is equal to
View SolutionThe remainder obtained when the polynomial x4−3x3+9x2−27x+81 is divided by x-3 is
View SolutionThe probability that a non leap year will have 53 Wednesdays is
View SolutionHow many permutations can be made using all the letters of the word FLOWER?
View SolutionFind the number of words formed by permuting all the letters of the following word: RUSSIA
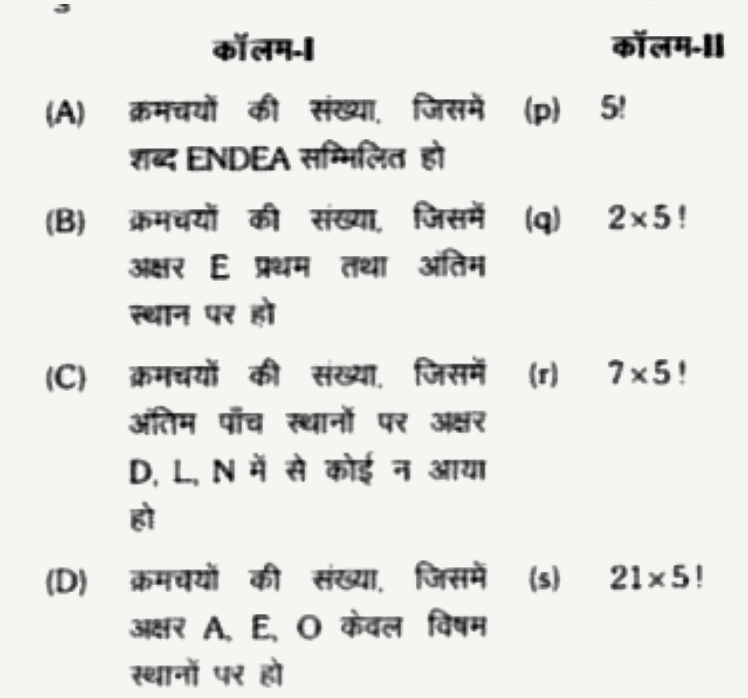
View SolutionConsider all possible permutations of the letters of the word ENDEANOEL. Match the statements/ expressions in column I with the statement/expressions in

View SolutionConsider all possible permutations of the letters of the word ENDEANOEL
Match the statements/Expression in Column-I with the statements/Expressions in Column-II.
Column - I
(A) The number of permutations containing the word ENDEA is
(B) The number of permutations in which the letter E occurs in the first and the last position is
(C) The number of permutations in which none of the letters D, L, N occurs in the last five positions is
(D) The number of permutations in which the let- ters A, E, O occurs only in odd positions is
Column -II
(P) 5!
(Q) 2×5!
7×5!
(S)21×5!
View SolutionIF all permutations of the letters of the word INTER are arranged as in dictionary , then fifieth word is
View SolutionThe number of permutations that can be made using all the letters of the word DEEPTI is
View Solutionशब्द ENDEANOEL के अक्षरों के सभी संभावित क्रमचय को मान्य करना है।
कॉलम I में दिए गए वक्तव्यों का कॉलम II में दिये गये मानों से सुमेल करें
View SolutionIf all permutations of the letters of the word INTER are arranged as in dictionary, then fiftieth word is
View Solutionशब्द ENDEANOEL के अक्षरों के सभी संभावित क्रमचय को मान्य करना है।
कॉलम I में दिए गए वक्तव्यों का कॉलम II में दिये गये मानों से सुमेल करें

View Solution{:("IUPAC Name", "Formulae of ligand"),((i)"Bromo",(A)CO),((ii)"Carbonyl",(B)C_(6)H_(5)COO^(-)),((iii)"Benzoato",(C)H_(2)O),((iv)"Aquo",(D)Br^(-)):} The correct match is
View SolutionNumber of permutations that can be made using all the letters of the word MATRIX is
View SolutionFind the number of words formed by permuting all the letters of the following word: INDIA
View Solution
