For the damped oscillator, the mass m of the block is 200g,k=90Nm−1 and the damping constant b is 40gs−1. Calculate the period of oscillation.Solution in Gujrati
√km=√90×0.2
√18=4.2426≈4.243kgs−1 and b=0.04kgs−1
∴b<<√km
The time period T=2π√mk
=2×3.14√0.290
=6.28×0.471=0.296
∴T≈0.3s.
Topper's Solved these Questions
- OSCILLATIONSBook:KUMAR PRAKASHANChapter:OSCILLATIONSExercise:SECTION-B (NUMERICAL FROM TEXTUAL EXERCISE)
Explore 48 Videos
- OSCILLATIONSBook:KUMAR PRAKASHANChapter:OSCILLATIONSExercise:SECTION-B (ADDITIONAL EXERCISE)
Explore 9 Videos
- OSCILLATIONSBook:KUMAR PRAKASHANChapter:OSCILLATIONSExercise:SECTION-A (TRY YOURSELF)
Explore 64 Videos
- OBJECTIVE QUESTIONS AS PER NEW PAPER STYLE Book:KUMAR PRAKASHANChapter:OBJECTIVE QUESTIONS AS PER NEW PAPER STYLE Exercise:CHAPTER - 8 (Match Type questions)
Explore 5 Videos
- PHYSICAL WORLDBook:KUMAR PRAKASHANChapter:PHYSICAL WORLDExercise:SECTION-E (QUESTIONS FROM MODULE)
Explore 9 Videos
Similar Questions
Which of the following examples represent periodic motion?
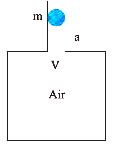
View SolutionAn air chamber of volume V has a neck area of cross section a into which a ball of mass m just fits and can move up and down without any friction (Fig.14.27). Show that when the ball is pressed down a little and released , it executes SHM. Obtain an expression for the time period of oscillations assuming pressure-volume variations of air to be isothermal [see Fig. 14.27].
View SolutionWhat is motion ?
View SolutionType of satellites are given in Column-I and their uses are given in Column-II. Match them properly :
Column-IColumn-II(1) Polar satellite(a) Tele communication(2) Geo-stationary satellite(b) Investigation(c) For information about atmosphere
View Solution.…. state of substance at very high temperature has generated hope of energy source for mankind.
View SolutionFor the damped oscillator shown in previous figure, k=180Nm−1 and the damping constant b is 40gs−1 .Period of oscillation is given as 0.3 s, find the mass of the block . (Assume b is much less than √km).
Hint :T=2π√mk
View SolutionThere is a LCR circuit , If it is compared with a damped oscillation of mass m oscillating with force constant k and damping coefficient 'b'. Compare the terms of damped oscillation with the devices in LCR circuit.
View Solutionकिसी अवमंदित ढोलक के लिए गुटके द्रव्यमान m=200g,k=90Nm−1 तथा अवमंदन स्थिर b=40gs−1 है।
दोलन का आवर्तकाल
View SolutionFor the damped oscillator shown in previous figure, k=180Nm−1 and the damping constant b is 40gs−1 .Period of oscillation is given as 0.3 s, find the mass of the block . (Assume b is much less than √km).
View SolutionFor a damped oscillator the mass 'm' of the block is 200 g, k = 90 N m^(-1) and the damping constant b is 40 g s^(-1) . Calculate the period of oscillation.
View SolutionFor a damped oscillator. The mass of the block is 200 g. k=90 N.m^-1 and the damping constant b is 40 g. s^-1 . Calculate the period of oscillation.
View SolutionA LCR circuit behaves like a damped harmonic oscillator. Comparing it with a physical spring- mass damped oscillator having damping constant b, mass m and oscillating with a force constant k, the correct equivalence will be
View SolutionFor the damped oscillator, the mass m of the block is 200g,k=90Nm−1 and the damping constant b is 40gs−1. Calculate time taken for its amplitude of vibrations to drop to half of its initial value.
View SolutionFor the damped oscillator, the mass m of the block is 200g,k=90Nm−1 and the damping constant b is 40gs−1. Calculate the time taken for its mechanical energy to drop to half its initial value.
View SolutionFor the damped oscillator, the mass m of the block is 400g, k = 120 Nm^(-1) and the damping constant b is 50 gs^(-1) . Calculate the period of oscillation.
View Solution