(A): ABAB ..... pattern of close packing gives hcp arrangement
(R): In hcp arrangement each sphere is associated with two tetrahedral voids Solution in Telugu
Both (A) and (R) are true and (R) is the correct explanation of (A)
Both (A) and (R) are true and (R) is not the correct explanation of (A)
(A) is true but (R) is false
Both (A) and (R) are false
The correct Answer is:B
Step by step video, text & image solution for (A): ABAB ..... pattern of close packing gives hcp arrangement (R): In hcp arrangement each sphere is associated with two tetrahedral voids by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SOLID STATEBOOK - AAKASH SERIESCHAPTER - SOLID STATEEXERCISE - LEVEL - I (EXERCISE - I)
68 Videos
- SOLID STATEBOOK - AAKASH SERIESCHAPTER - SOLID STATEEXERCISE - LEVEL - I (EXERCISE - II)
77 Videos
- SOLID STATEBOOK - AAKASH SERIESCHAPTER - SOLID STATEEXERCISE - OBJECTIVE EXERCISE -3
22 Videos
- REVISION EXERCISE BOOK - AAKASH SERIESCHAPTER - REVISION EXERCISE EXERCISE - COMPLEX COMPOUNDS
47 Videos
- SOLIDS STATEBOOK - AAKASH SERIESCHAPTER - SOLIDS STATEEXERCISE - PRACTICE EXERCISE
63 Videos
Similar Questions
Which of the following is amorphous in nature?
View SolutionWhich of the following is not the true about crystalline solids
View SolutionWith which one of the following elements silicon should be doped so as to give p-type sem iconductor?
View SolutionIn which of the following complexes the metal ion is in zero oxidation state ?
View SolutionWhich of the following is considered as true amorphous solid
View SolutionIf in a closest packed arrangement r is the radius of the sphere respresenting the tetrahedral void and R is the radius of the spheres in closest packed arrangement then
View SolutionWhat are tetrahedral and octahedral voids in a close-packed structure of particles? How many voids are there in a ccp structure of 'N' atoms? Why is the number of octahedral voids in an hcp or a ccp arrangement of particles equal to the number of packed particles?
View SolutionIn HCP or CCP constituent particles occupy 74% of the available space. The remaining space (26%) in between the spheres remains unoccupied and is called interstitial voids or holes. Considering the close packing arrangement, each sphere in the second layer rests on the hollow space of the first layer, touching each other. The void created is called tetrahedral void. If R is the radius of the spheres in the close packed arrangement then,
R (radius of tetrahedral void) = 0.225 R
In a close packing arrangement, the interstitial void formed by the combination of two triangular voids of the first and second layer is called octahedral coid. Thus, double triangular void is surrounded by six spheres. The centre of these spheres on joining, forms octahedron. If R is the radius of the sphere. in a close packed arrangement then,
R (radius of octahedral void = 0.414 R).
In the figure given below, the site marked as S is a
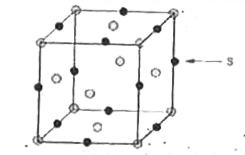
View SolutionIn HCP or CCP constituent particles occupy 74% of the available space. The remaining space (26%) in between the spheres remains unoccupied and is called interstitial voids or holes. Considering the close packing arrangement, each sphere in the second layer rests on the hollow space of the first layer, touching each other. The void created is called tetrahedral void. If R is the radius of the spheres in the close packed arrangement then,
R (radius of tetrahedral void) = 0.225 R
In a close packing arrangement, the interstitial void formed by the combination of two triangular voids of the first and second layer is called octahedral coid. Thus, double triangular void is surrounded by six spheres. The centre of these spheres on joining, forms octahedron. If R is the radius of the sphere. in a close packed arrangement then,
R (radius of octahedral void = 0.414 R).
If the anions (A) form hexagonal close packing and cations (C ) occupy only 2/3rd octahedral voids in it, then the general formula of the compound is
View SolutionIn HCP or CCP constituent particles occupy 74% of the available space. The remaining space (26%) in between the spheres remains unoccupied and is called interstitial voids or holes. Considering the close packing arrangement, each sphere in the second layer rests on the hollow space of the first layer, touching each other. The void created is called tetrahedral void. If R is the radius of the spheres in the close packed arrangement then,
R (radius of tetrahedral void) = 0.225 R
In a close packing arrangement, the interstitial void formed by the combination of two triangular voids of the first and second layer is called octahedral coid. Thus, double triangular void is surrounded by six spheres. The centre of these spheres on joining, forms octahedron. If R is the radius of the sphere. in a close packed arrangement then,
R (radius of octahedral void = 0.414 R).
Mark the false statement :
View SolutionIn HCP or CCP constituent particles occupy 74% of the available space. The remaining space (26%) in between the spheres remains unoccupied and is called interstitial voids or holes. Considering the close packing arrangement, each sphere in the second layer rests on the hollow space of the first layer, touching each other. The void created is called tetrahedral void. If R is the radius of the spheres in the close packed arrangement then,
R (radius of tetrahedral void) = 0.225 R
In a close packing arrangement, the interstitial void formed by the combination of two triangular voids of the first and second layer is called octahedral coid. Thus, double triangular void is surrounded by six spheres. The centre of these spheres on joining, forms octahedron. If R is the radius of the sphere. in a close packed arrangement then,
R (radius of octahedral void = 0.414 R).
In Schottky defect
View SolutionThe arrangement of spheres in hexagonal close packing (hcp) is :
View SolutionTotal number of tetrahedral and octahedral voids in hcp arrangment are 1218.
View SolutionAssertion (A) : Packing efficiency of hcp and ccp structures is 74%.
Reason (R) : If the number of close packed spheres is N, then number of tetrahedral voids is N.
View Solution(A): ABAB ..... pattern of close packing gives hcp arrangement
(R): In hcp arrangement each sphere is associated with two tetrahedral voids
View Solution
