A candidate obtained the following percentage of marks in an examination English 60, Maths 90, Physics 75, Chemistry 66. If weighs 2, 4, 3, 3 are allotted to these subjects respectively, then the weight mean is given by
60+90+75+662+4+3+3
60×2+90×4+75×3+66×32+4+3+3
60×2+90×4+75×3+66×34
60+90+75+664
The correct Answer is:B
To find the weighted mean of the percentages obtained by the candidate in the examination, we will follow these steps:
1. Identify the given data:
- Percentages:
- English: 60%
- Maths: 90%
- Physics: 75%
- Chemistry: 66%
- Weights:
- English: 2
- Maths: 4
- Physics: 3
- Chemistry: 3
2. Multiply each percentage by its corresponding weight:
- For English: 60×2=120
- For Maths: 90×4=360
- For Physics: 75×3=225
- For Chemistry: 66×3=198
3. Sum the weighted values:
Total Weighted Marks=120+360+225+198=903
4. Sum the weights:
Total Weights=2+4+3+3=12
5. Calculate the weighted mean:
Weighted Mean=Total Weighted MarksTotal Weights=90312=75.25
Final Answer:
The weighted mean of the percentages is 75.25.
- Percentages:
- English: 60%
- Maths: 90%
- Physics: 75%
- Chemistry: 66%
- Weights:
- English: 2
- Maths: 4
- Physics: 3
- Chemistry: 3
- For English: 60×2=120
- For Maths: 90×4=360
- For Physics: 75×3=225
- For Chemistry: 66×3=198
Total Weighted Marks=120+360+225+198=903
Total Weights=2+4+3+3=12
Weighted Mean=Total Weighted MarksTotal Weights=90312=75.25
The weighted mean of the percentages is 75.25.
Topper's Solved these Questions
Similar Questions
A student obtained the following marks in percentages in his semester examination English 50, Maths 65, Statistics 70, Economics 58 and Accountancy 63. The weights of these subjects are 2, 2, 1, 1 and 1 respectively. What is the weighted arithmetic mean? (a) 60 (b) 61 (c) 62 (d) 63
View SolutionA student obtains the following percentage in an examination.
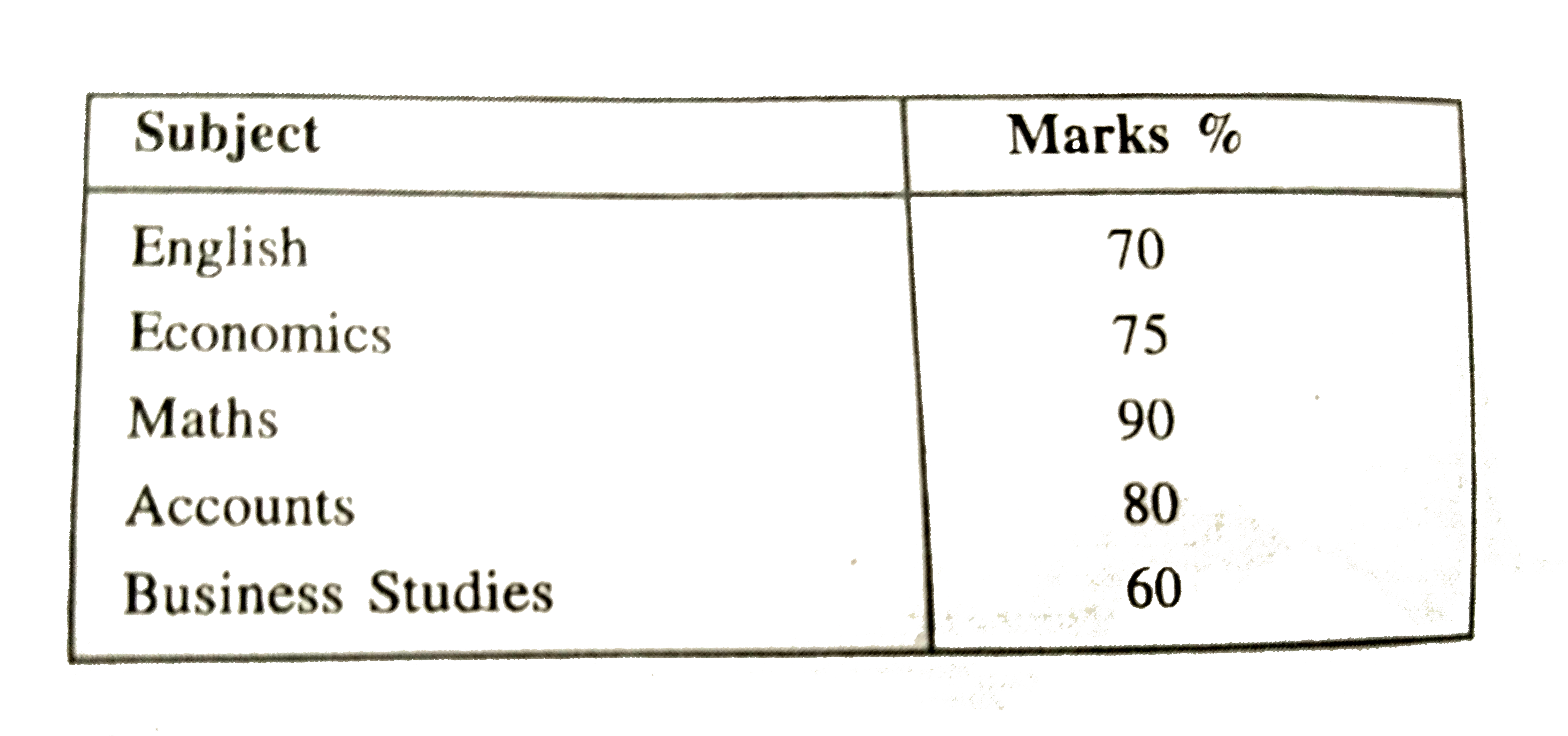
(i) Find out simple mean marks , if all subjectes are given equal weight.
(ii) Find out weighted mean marks, if the respective weights are 1,2,1,2,3
View Solution
Knowledge Check
In an examination, a candidate scores the following percentage of marks. English-44, Hindi-58, Maths—74, Physics-61, Chemistry—62. If weights 2, 4, 4, 5, 3 respectively, are allotted to these subjects, then the candidate's weighted mean percentage is
A61B61.5C62D62.5A candidate obtains the marks in subjects English 60 Hindi 75 , Mathematics 63 , Physics 59 and Chemistry 55 . In the weights of 1,2,1,3,3 respectively are alloted to the subjects then the candidates weighted mean is
A59B615C10D61.5A candidate obtains the marks in subjects, English 40, Hindi 30, Mathematics 60, Physics 70 and Chemistry 40. In the weights of 1, 2, 2, 3, 3 respectively are allotted to the subjects, then in the candidates weighted mean is
A59B615C10D50
The mean and standard deviation of marks obtained by 50 students of a class in three subjects, Mathematics, Physics and Chemistry are given below:
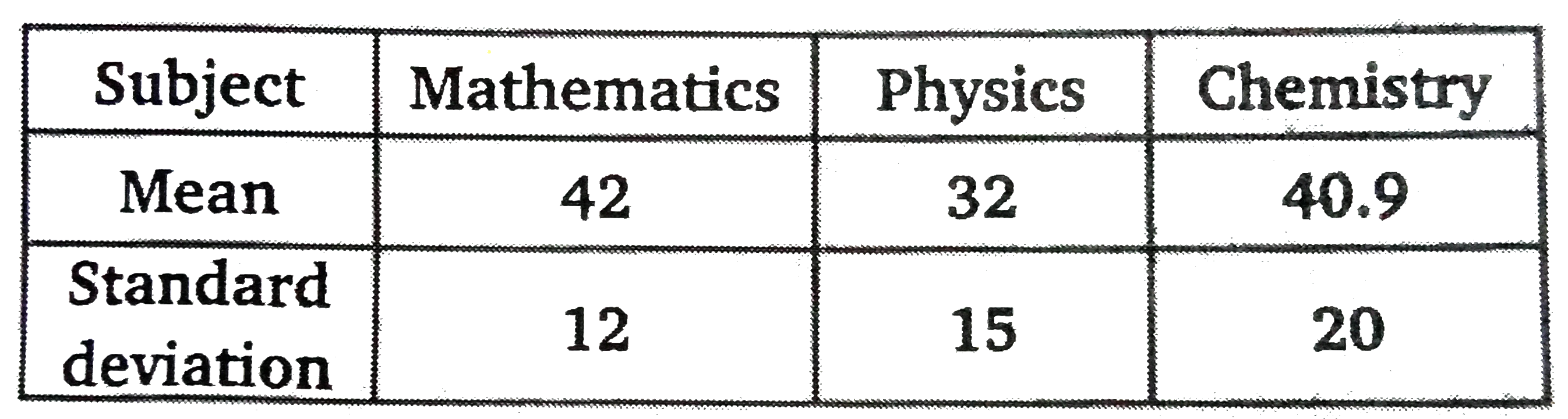
Which of the three subjects shows the highest variability in marks and which shows the lowest?
View SolutionThe following data shows the number of students opting different subject in a college: Subjects, English, Maths, Physics, Chemistry, Eco., Commerce No. of Students, 45, 60, 20, 30, 10, 15 Construct a pie-diagram to represent the above data.
View SolutionTwo friends Jack and Jill attend IIT entrance test which has three sections, Mathematics, Physics and Chemistry.Each question in Mathematics, Physics and Chemistry carry 5 marks, 8 marks and 3 marks respectively. Jack attempted 10 questions in Mathematics, 12 in Physics and 6 in Chemistry while Jill attemped 18, 5 and 9 questions in Mathematics, Physics and Chemistry respectively Assuming that all the questions attempted were correct, find the individual marks obtained by the boys by showing the above information as a matrix product.
View SolutionA student obtained the following marks per centage in an examination English - 50, Accounts - 75, Economics - 60, B Std.-80, Hindi - 55. If weights are 2, 3, 3, 2, 1, respectively allotted to the subjects, his weighted mean is
View SolutionThe mean and standard deviation of marks obtained by 50 students of a class in three subjects, Mathematics, physics and Chemistry are given below
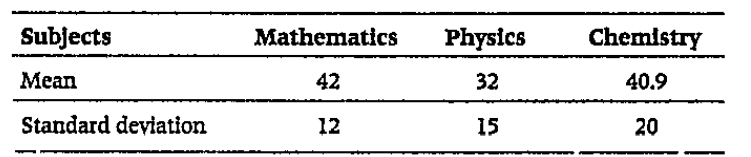
Which of these three subjects shows the highest variability in marks and which shows the lowest ?
View Solution
