A body of mass 5kg undergoes a change in speed from 20 to 0.20 m/s. The momentum of the body would
increase by 99kgm/s
decrease by 99kgm/s
increase by 101kgm/s
decrease by 101 kgm/s
The correct Answer is:B
To solve the problem of how the momentum of a body changes as its speed decreases from 20 m/s to 0.20 m/s, we will follow these steps:
Step 1: Understand the concept of momentum
Momentum (P) is defined as the product of mass (m) and velocity (v). The formula for momentum is:
P=m×v
Step 2: Identify the given values
- Mass of the body, m=5kg
- Initial velocity, v1=20m/s
- Final velocity, v2=0.20m/s
Step 3: Calculate the initial momentum (P1)
Using the formula for momentum:
P1=m×v1
Substituting the values:
P1=5kg×20m/s=100kg m/s
Step 4: Calculate the final momentum (P2)
Now, we calculate the final momentum using the final velocity:
P2=m×v2
Substituting the values:
P2=5kg×0.20m/s=1kg m/s
Step 5: Determine the change in momentum
To find the change in momentum, we subtract the final momentum from the initial momentum:
Change in momentum=P1−P2
Substituting the values:
Change in momentum=100kg m/s−1kg m/s=99kg m/s
Step 6: Conclusion
Since the momentum has decreased, the final answer is that the momentum of the body decreased by 99 kg m/s.
Final Answer
The momentum of the body would decrease by 99 kg m/s.
---
Momentum (P) is defined as the product of mass (m) and velocity (v). The formula for momentum is:
P=m×v
- Mass of the body, m=5kg
- Initial velocity, v1=20m/s
- Final velocity, v2=0.20m/s
Using the formula for momentum:
P1=m×v1
Substituting the values:
P1=5kg×20m/s=100kg m/s
Now, we calculate the final momentum using the final velocity:
P2=m×v2
Substituting the values:
P2=5kg×0.20m/s=1kg m/s
To find the change in momentum, we subtract the final momentum from the initial momentum:
Change in momentum=P1−P2
Substituting the values:
Change in momentum=100kg m/s−1kg m/s=99kg m/s
Since the momentum has decreased, the final answer is that the momentum of the body decreased by 99 kg m/s.
The momentum of the body would decrease by 99 kg m/s.
Topper's Solved these Questions
- LAWS OF MOTION, FRICTION AND ARCHIMEDES' PRINCIPLEBOOK - MCGROW HILL PUBLICATIONCHAPTER - LAWS OF MOTION, FRICTION AND ARCHIMEDES' PRINCIPLEEXERCISE - HIGHER ORDER THINKING QUESTIONS
34 Videos
- HEATING AND MAGNETIC EFFECTS OF CURRENT BOOK - MCGROW HILL PUBLICATIONCHAPTER - HEATING AND MAGNETIC EFFECTS OF CURRENT EXERCISE - HIGHER ORDER THINKING QUESTIONS
34 Videos
- MEASUREMENTBOOK - MCGROW HILL PUBLICATIONCHAPTER - MEASUREMENTEXERCISE - ELEMENTARY QUESTIONS (HIGHER ORDER THINKING QUESTIONS)
13 Videos
Similar Questions
A body of mass 3kg travelling with a velocity 5m/s collides head-on and elastically with a body of mass 2kg travelling at a speed 5m/s in opposite direction. The velocity of the second body after the collision is?
View SolutionA body of mass M moving with a speed V collides on a surface at an angle 45 degree without changing its speed the change in momentum of the body will be-
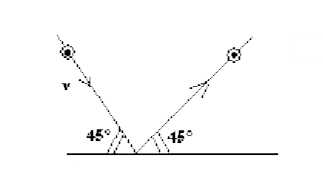
View Solution
Knowledge Check
A body of mass 5 kg undergoes a change in speed from 30 to 40m/s. Its momentum would increase by
A50kgm/sB75 kgm/sC150 kgm/sD350 kgm/sThe velocity of a body of mass 20 kg decreases from 20m/s to 5m/s in a distance of 100 m. Force on the body is:
A−27.5NB−47.5NC−37.5ND−67.5NThe velocity of a body of mass 20 kg decreases from 20m/s to 5m/s in a distance of 100 m. Force on the body is:
A−27.5NB−47.5NC−37.5ND−67.5N
A body of mass 2 kg is dropped from rest from a height 20 m from the surface of Earth. The body hiys the ground with velocity 10 m/s, then work done (g=10m/s2)
View SolutionA constant force acting on a body of mass of 5 kg change its speed from 5ms−1 to 10ms−1 in 10s without changing the direction of motion. The force acting on the body is
View SolutionQuestion stimulus:-
A constant force acting on a body of mass 5 kg changes its speed from 2ms−1 to 4ms−1 in 20 s, without changing its direction of motion. The magnitude of the force is
View SolutionA constant force acting on a body of mass 5kg change its speed from 5ms−1 in 10s without changing the direction of motion. The force acting on the body is
View SolutionA 60 kg body moving with speed 12 m/s collides inelastically with another body of mass 50 kg moving in same direction with speed 2 m/s. After collision the 50 kg body moves with a speed of 10 m/s. The value of coefficient of restitution (e) is :-
View Solution
