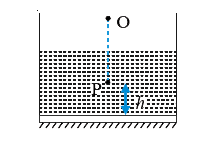
A plane mirror is placed at the bottom of the tank containing a liquid of refractive index μ. P is a small object at a height h above the mirror. An observer O-vertically above P outside the liquid see P and its image in the mirror. The apparent distance between these two will be:
2μh
2hμ
2hμ−1
h(1+1μ)
The correct Answer is:B
Image formation by a mirror ( either plane or spherical) does not depended on the medium.
The image of P will be formed at a distance h below the mirror. If d=depth of the liquid in the tank,
apparent depth of P=x1=d−hμ.
apparent depth of the image of P=x2=d+hμ.
apparent distance between P and its image=x_(2)-x_(1)=(2h)/(mu)*
Topper's Solved these Questions
- OPTICSBook:MTG GUIDEChapter:OPTICSExercise:Check your NEET vitals
Explore 25 Videos
- OPTICSBook:MTG GUIDEChapter:OPTICSExercise:AIPMT/NEET
Explore 55 Videos
- OPTICSBook:MTG GUIDEChapter:OPTICSExercise:AIPMT/NEET
Explore 55 Videos
- MAGNETIC EFFECTS OF CURRENT AND MAGNETISMBook:MTG GUIDEChapter:MAGNETIC EFFECTS OF CURRENT AND MAGNETISMExercise:AIPMT/NEET (MCQs)
Explore 48 Videos
Similar Questions
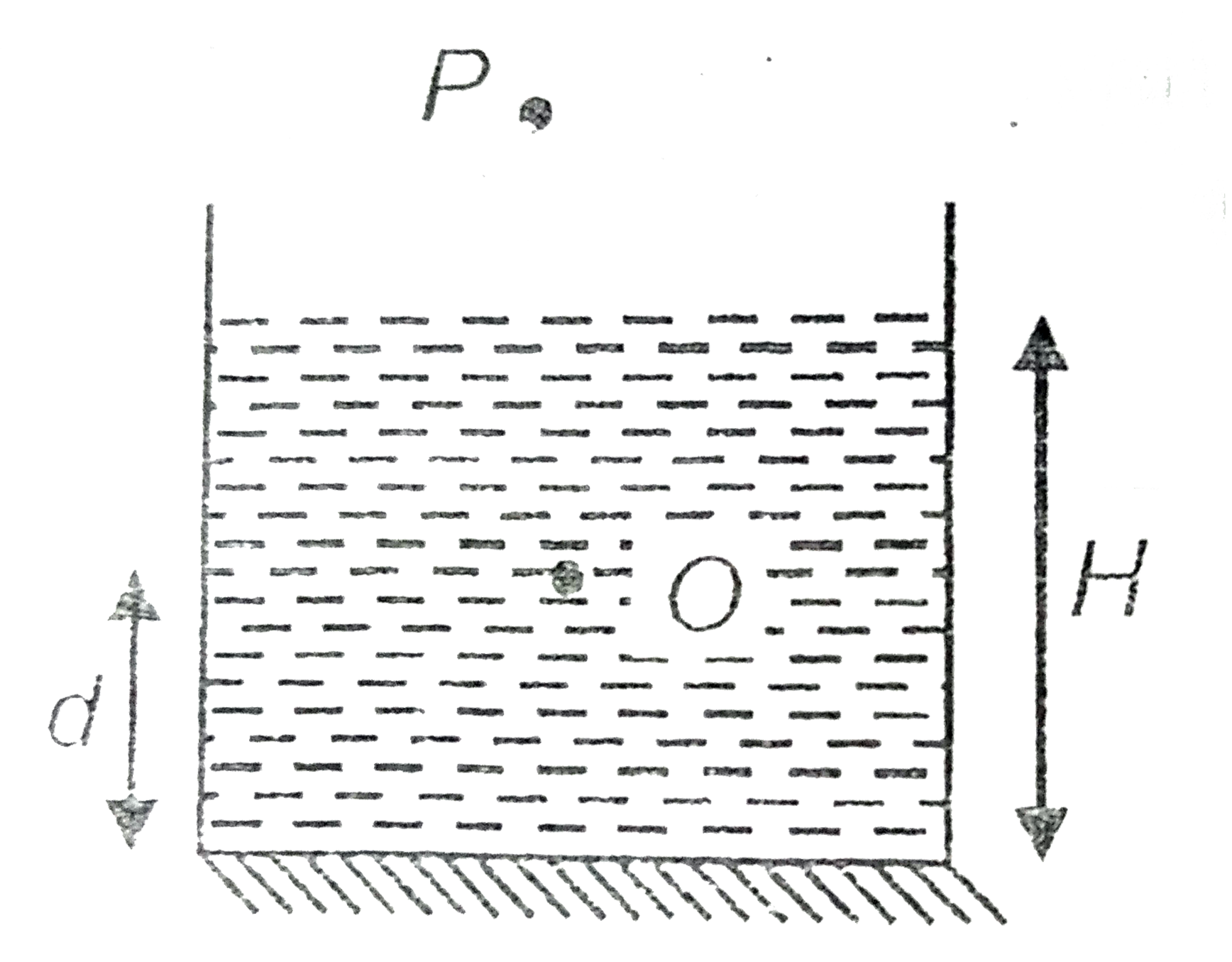
A tank contains a transparent liquid of refractive index μ. The bottom of the tank is a plane mirror as shown. A person at P looks at an object O and its image in the mirror. The distance between the object and its image in the mirror as preceived by the person is
View SolutionIn Fig. a plane mirror lies at a height h above the bottom of a beaker containingwater (refractive index μ) upto a height f. Find the position of the image of the
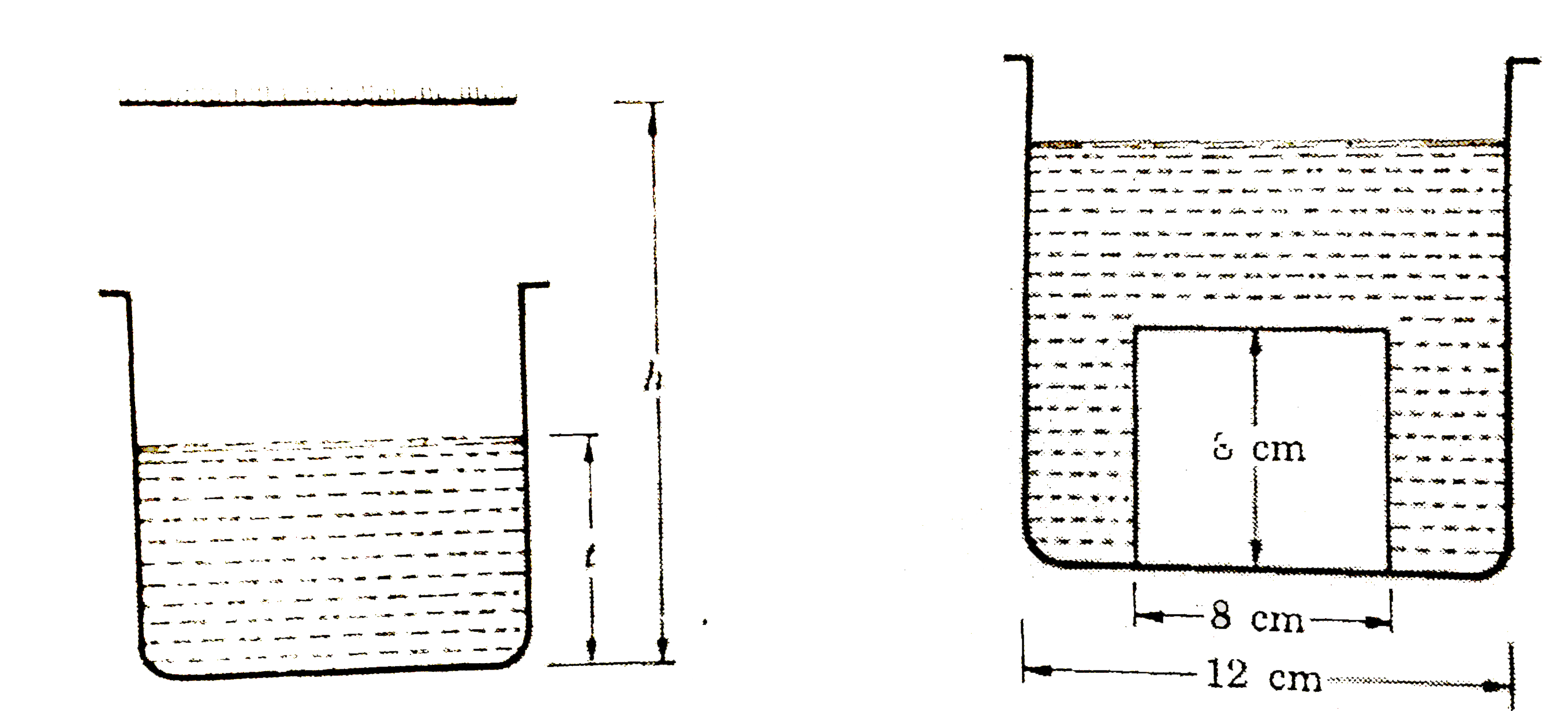
View Solution
Knowledge Check
A plane mirror is placed at the bottom of a tank containing a liquid of refractive index μ. P is a small object at a height h above the mirror. An observes P and its image in the mirror. The apparent distance between two will be
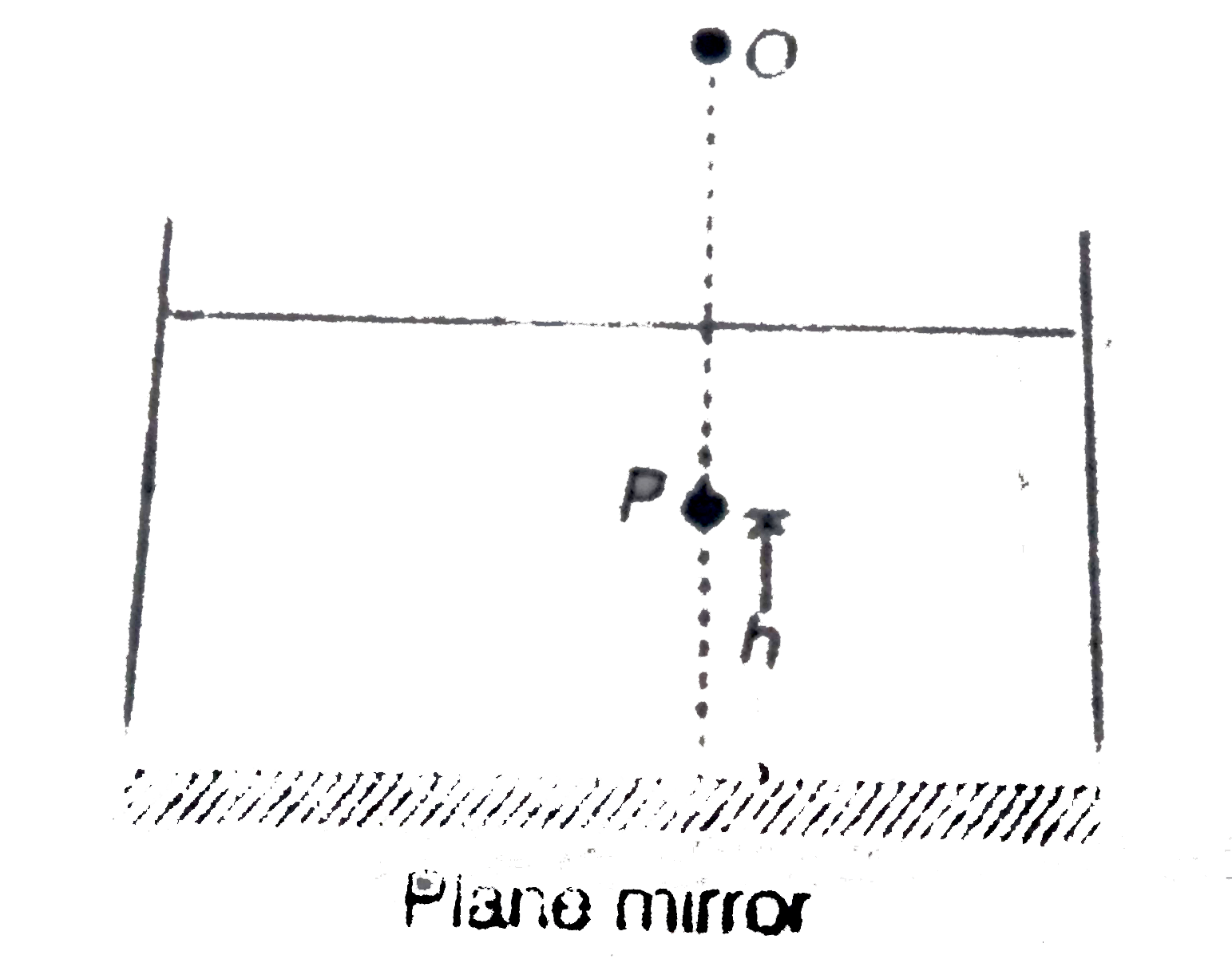 A2μhB2hμC2hμ−1Dh(1+1μ)
A2μhB2hμC2hμ−1Dh(1+1μ)A concave mirror of radius of curvature h is placed at the bottom of a tank containing a liquid of refractive index μ upto a depth d. An object P is placed at height h above the bottom of the miror. Outside the liquid, an observer O views the object and its image in the mirror. The apparent distance between these two will be
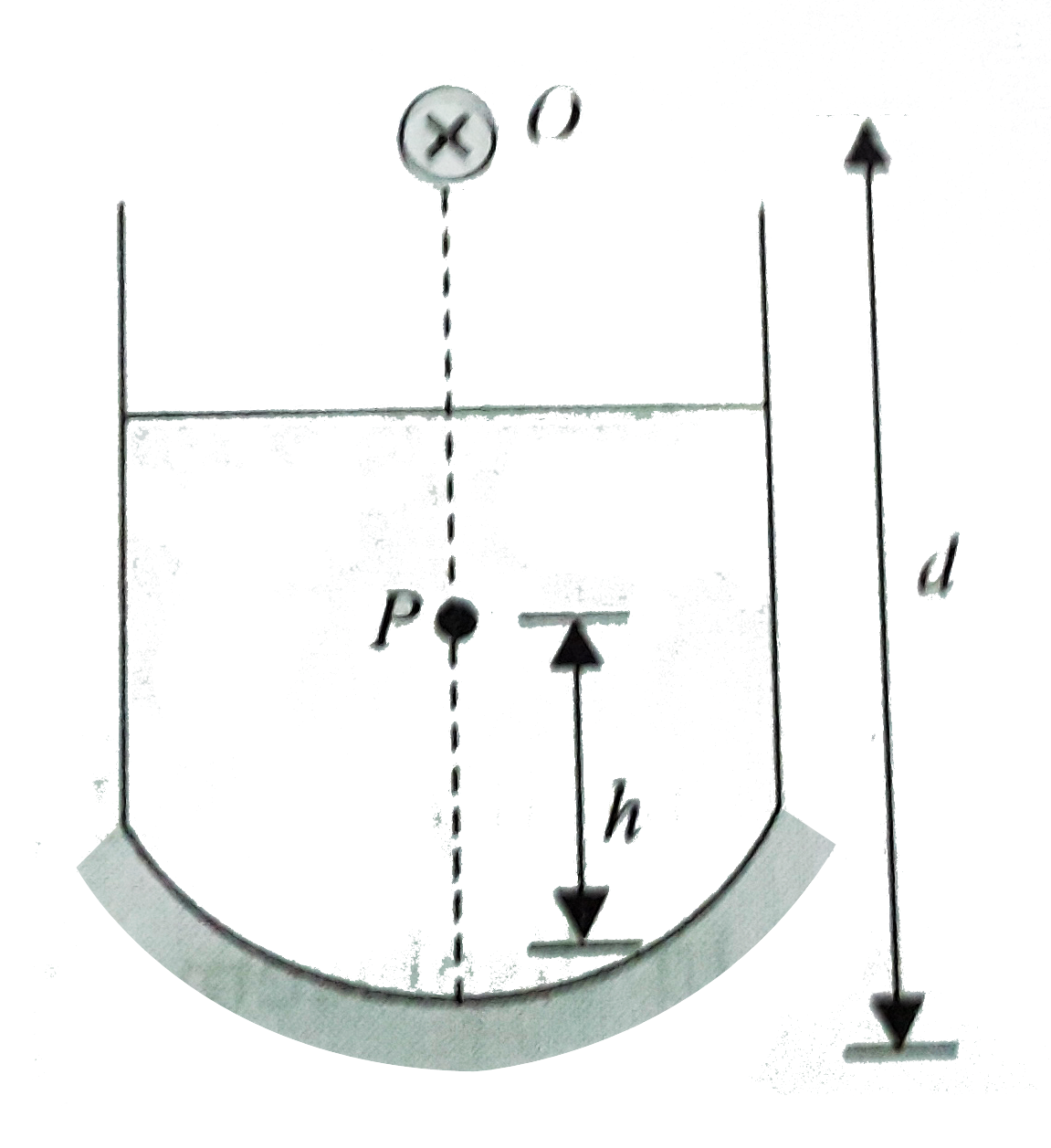 Ah(1−1μ)B2hμ−1CzeroD2hμ
Ah(1−1μ)B2hμ−1CzeroD2hμThere is a plane mirror at the bottom of tank filled with water having refractive index 4/3. A point object is placed at a height 10 cm above the bottom. What will be the apparent distance between point object and its image in plane mirror as observed by an observer outside the liquid in air ? Line joining observer and object is normal to the surface of water.
A10 cmB15 cmC20 cmD25cm
An object is placed at the bottom of a tank filled with liquid of refractive index 1.6. If the actual depth of the tank is 10 cm, what will be the apparent depth of the object?
View SolutionA small illuminated bulb is at the bottom of a tank, containing a liquid of refractive index μ upto a height H. Find the expression for the diameter of an opaque disc, floating symmetrically on the liquid surface in order to cut- off the light from the bulb.
View SolutionA plane mirror is fixed at the bottom of a vessel as shown in the figure. Water of refractive index μ is filled up to a height h in the vessel. There is a fish at a height h/2 from bottom of vessel and there is a bird at a height h above the surface of water in the vessel.
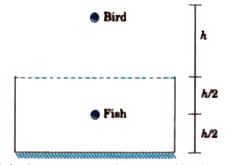
(a) Calculate apparent distance of bird as seen by the fish.
(b) Calculate apparent distance of image of bird in mirror as seen by the fish.
(c) Calculate apparent distance of fish as seen by the bird.
(d) Calculate apparent distance of image of fish in the mirror as seen by the bird.
View SolutionIn figure, a point source S is placed at a height h above the plane mirror in a medium of refractive index μ.
a. Find the number of images seen for normal view.
b. Find the distance between the images.

View SolutionTwo plane mirrors A and B are placed at a distance 12 cm apart facing towards each other . An object is placed in between them at a distance 8 cm from the mirror A . What will be the distance between them at a distance 8 cm from the mirror A . What will be the distance between the first two images formed in the mirror B
View Solution