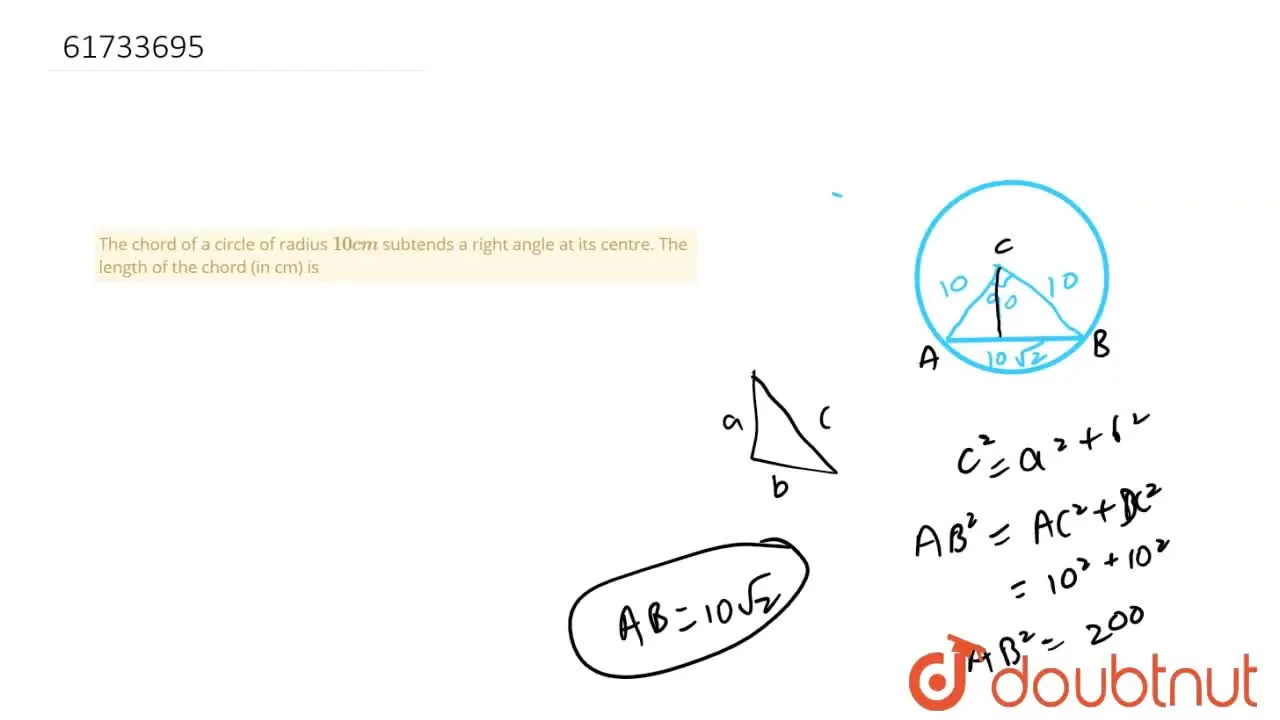
The chord of a circle of radius 10cm subtends a right angle at its centre. The length of the chord (in cm) is
5√2
5√2
10√2
10√3
The correct Answer is:C
AB=√OA2+OB2=√102+102=10√2cm.
Related Playlists
- BOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Multiple-choice questions (MCQ)39videos
CIRCLES
- BOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Assertion-and-Reason1videos
CIRCLES
- BOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Exercise 8B15videos
CIRCLES
- BOOK - RS AGGARWALCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Multiple Choice Questions (Mcq)30videos
ARITHMETIC PROGRESSION
- BOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - Test Yourself10videos
CONSTRUCTIONS
Similar Questions
A chord of a circle of radius 10cm subtends a right angle at the centre. The length of the chord (in cm) is 5√2 (b) 10√2 (c) 5√2 (d) 10√3
View SolutionThe chord of a circle of radius 8 cm subtends a right angle at its centre. Find the length of the chord.
View SolutionA chord of a circle of radius 10 cm subtends a right angle at the centre. Find the area of the corresponding :
(i) minor segment
(ii) major sector.View SolutionA chord of a circle of radius 10 cm subtends a right angle at the centre. Find the area of the corresponding :
Minor segmentView SolutionA chord of a circle of radius 10 cm subtends a right angle at the centre. Find the area of the corresponding sector.
View SolutionA chord of a circle of radius 10 cm subtends a right angle at the centre. Find the area of the corresponding :
Major sectorView SolutionA chord of a circle of radius 10cm subtends a right angle at the centre. The area of the minor segments (given π=3.14) is
View SolutionA chord of a circle of radius 14 cm subtends a right angle at the centre. The area of the minor segments (given π = 3.14 ) is
View SolutionA chord of a circle of radius 15 cm subtends an angle of 60∘ at the centre . Find the areas of the corresponding minor and major segments of the circle.
View SolutionA chord AB of a circle of radius 10cm subtends a right angle at the centre. Find: area of the minor sector area of the minor segment area of the major sector area of the major segment
View Solution