Show that the diagonals of a parallelogram divide it into four triangles of equal area.
Video Solution
Text Solution
Let E, E, F be the midpoints of BC, CA and AB.
Then, DE =12AB=AF,DF=12AC=AE.
∴△AFE≅△DEF [∵AF=DF,AE=DF,FE=EF].
Similarly, △DEF≅△FBDand△DEF≅△EDC.
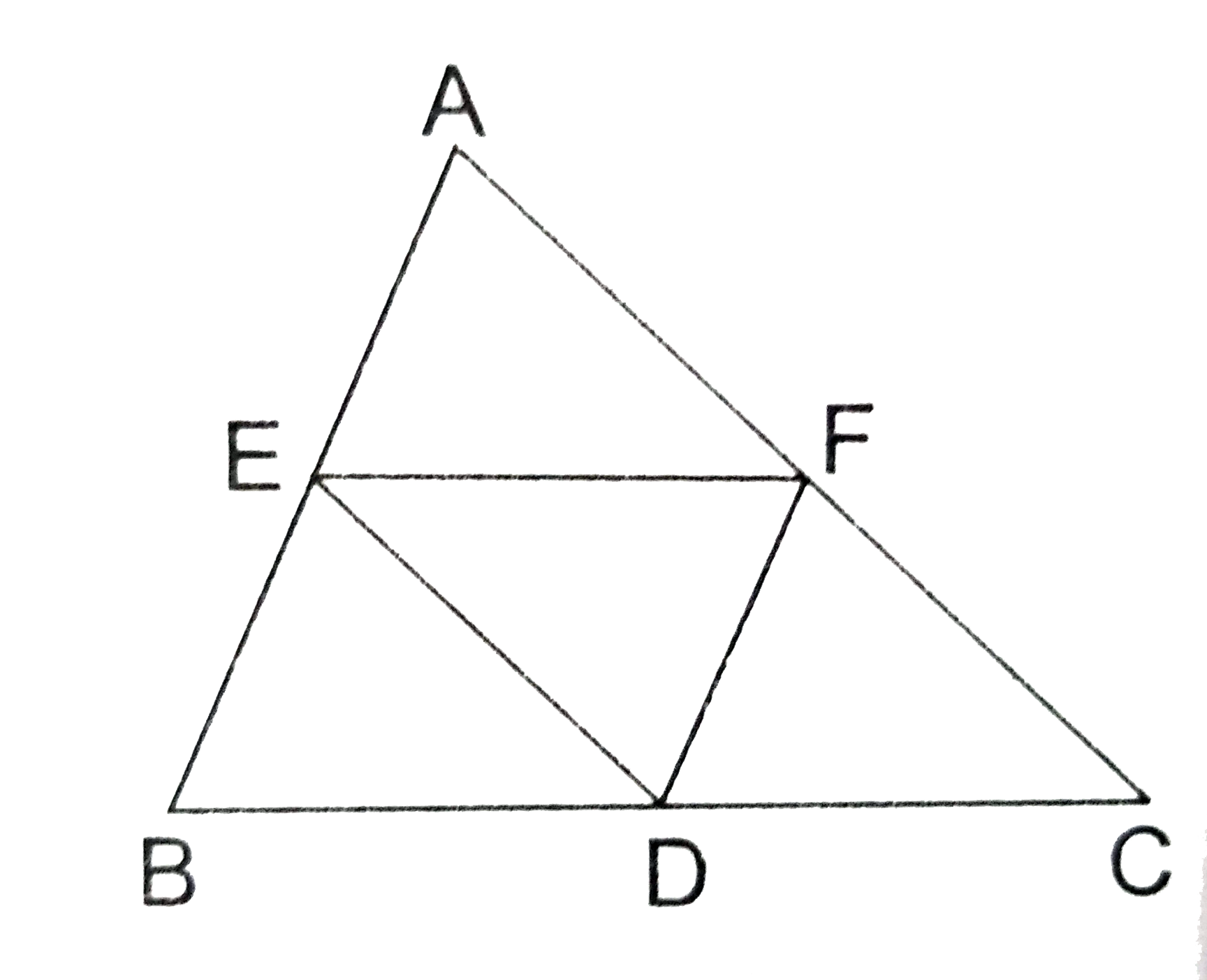
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
Show that each diagonal of a parallelogram divide it into two congruent triangles.
The following are the steps involved in showing the above result. Arrange them in sequential order.
A) In △ABC and △CDA, AB=DC and BC=AD (∴ opposite angles of parallelogram) AC=AC (common side).
B) Let ABCD be a parallelogram. Join AC.
C) By SSS congruence property, △ABC≅△CDA.
D) Similarly, BD divides the triangle into two congruent triangles.ABACDBBDACCBADCDBDCA - Question 2 - Select One
Asserion (A) : The diagonals of a ||gm divide it into four triangle of equal area.
Reason (R ) : A diagonal of a ||gm divides it into two triangle of equal area.ABoth Assertion (A) and Reason (R ) are true and Reason (R ) is a correct explansion of Assertion (A).BBoth Assertion (A) and Reason (R ) are true but Reason (R ) is not a correct explansion of Assertion (A).CAssertion (A) is true and Reason (R ) is false.DAssertion (A) is false and Reason (R ) is true.
A diagonal of a parallelogram divides it into two congruent triangles.
View SolutionA diagonal of parallelogram divides it into two congruent triangles.
View SolutionA diagonal of a parallelogram divides it into two triangles of equal area. GIVEN : A parallelogram ABCD in which BD is one of the diagonals. TO PROVE : ar (ABD)=ar(CDB)
View SolutionThe diagonals of a parallelograms are equal .
View SolutionShow that a median of a triangle divides it into two triangles of equal areas.
View SolutionShow that a median of a triangle divides it into two triangles of equal area.
View Solution

