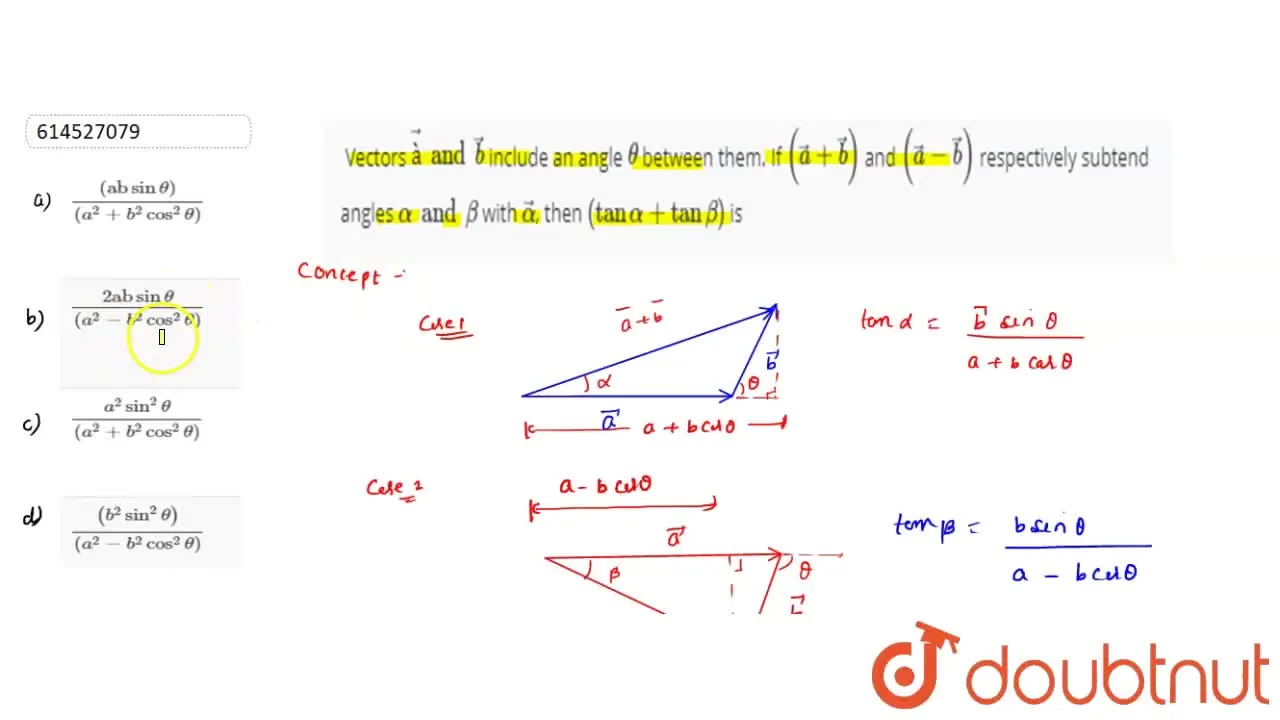
Vectors vecà and vecb include an angle $θ$ between them. If $(\to a + \to b)$ and $(\to a - \to b)$ respectively subtend angles $α and β$ with $\to a$ , then $(tan α + tan β)$ is

A

$\frac{(ab sin θ)}{(a^{2} + b^{2} {cos}^{2} θ)}$

B

$\frac{2 ab sin θ}{(a^{2} - b^{2} {cos}^{2} θ)}$

C

$\frac{a^{2} {sin}^{2} θ}{(a^{2} + b^{2} {cos}^{2} θ)}$

D

$\frac{(b^{2} {sin}^{2} θ)}{(a^{2} - b^{2} {cos}^{2} θ)}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:B

To solve the problem, we need to find the expression for $tan α + tan β$ where $α$ and $β$ are the angles that the resultant vectors $\to a + \to b$ and $\to a - \to b$ make with vector $\to a$ , respectively.
1. Understanding the Vectors:
- Let $\to a$ and $\to b$ be two vectors with an angle $θ$ between them.
- The resultant vector $_{1} = \to a + \to b$ makes an angle $α$ with $\to a$ .
- The resultant vector $_{2} = \to a - \to b$ makes an angle $β$ with $\to a$ .
2. Finding $tan α$ :
- The tangent of angle $α$ can be expressed as:
$tan α = \frac{| \to b | sin θ}{| \to a | + | \to b | cos θ}$
- Here, $| \to b | sin θ$ is the perpendicular height from $\to b$ to the resultant $_{1}$ , and $| \to a | + | \to b | cos θ$ is the base.
3. Finding $tan β$ :
- Similarly, for $tan β$ :
$tan β = \frac{| \to b | sin θ}{| \to a | - | \to b | cos θ}$
- Here, $| \to b | sin θ$ is the perpendicular height from $- \to b$ to the resultant $_{2}$ , and $| \to a | - | \to b | cos θ$ is the base.
4. Adding $tan α$ and $tan β$ :
- Now, we can add $tan α$ and $tan β$ :
$tan α + tan β = \frac{| \to b | sin θ}{| \to a | + | \to b | cos θ} + \frac{| \to b | sin θ}{| \to a | - | \to b | cos θ}$
- This can be simplified using a common denominator:
$= \frac{| \to b | sin θ (| \to a | - | \to b | cos θ) + | \to b | sin θ (| \to a | + | \to b | cos θ)}{(| \to a | + | \to b | cos θ) (| \to a | - | \to b | cos θ)}$
- Simplifying the numerator:
$= \frac{2 | \to b | | \to a | sin θ}{| \to a |^{2} - | \to b |^{2} {cos}^{2} θ}$
5. Final Result:
- Thus, we arrive at the final expression:
$tan α + tan β = \frac{2 | \to a | | \to b | sin θ}{| \to a |^{2} - | \to b |^{2} {cos}^{2} θ}$
Conclusion:
The value of $tan α + tan β$ is given by:
$\frac{2 | \to a | | \to b | sin θ}{| \to a |^{2} - | \to b |^{2} {cos}^{2} θ}$

Updated on:18/9/2024

|

Class 11 PHYSICS RECTILINEAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Vectors $\to A$ and $\to B$ include an angle $θ$ between them. If $(\to A + \to B)$ and $(\to A - \to B)$ respectively subtend angles $α and β$ with $\to A$ , then $(tan α + tan β)$ is
A $\frac{(A sin θ)}{(A^{2} + B^{2} {cos}^{2} θ)}$
B $\frac{(2 A B sin θ)}{(A^{2} - B^{2} {cos}^{2} θ)}$
C $\frac{(A^{2} {sin}^{2} θ)}{(A^{2} + B^{2} {cos}^{2} θ)}$
D $\frac{(B^{2} {sin}^{2} θ)}{(A^{2} - B^{2} {cos}^{2} θ)}$
If $\to a$ and $\to b$ are unit vectors inclined at an angle $θ$ , then the value of $∣ ∣ \to a - \to b ∣ ∣$ is
A $2 \frac{sin (θ)}{2}$
B $2 sin θ$
C $2 cos θ \frac{θ}{2}$
D $2 cos θ$
If $\to a and \to b$ are two vectors and angle between them is $θ$ , then
A ${∣ ∣ \to a \times \to b ∣ ∣}^{2} + {(\to a . \to b)}^{2} = {| \to a |}^{2} {∣ ∣ \to b ∣ ∣}^{2}$
B ${∣ ∣ \to a \times \to b ∣ ∣}^{2} + {(\to a . \to b)}^{2}, if θ = π / 4$
C $\to a \times \to b = (\to a . \to b) ˆ n$ ( where $ˆ n$ is a normal unit vector ) $if θ f = π / 4$
D $(\to a \times \to b) . (\to a + \to b) = 0$

MTG GUIDE-KINEMATICS -Topicwise Practice Questions (VECTORS)

Home
Ask Doubt
Profile