If X is a random variable with probability distribution P(X=k)=(k+1)C2k,K=0,1,2,…. then find C. Solution in Telugu
The correct Answer is:14
Step by step video, text & image solution for If X is a random variable with probability distribution P(X=k)=((k+1)C)/(2^(k)),K=0,1,2,…. then find C. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RANDOM VARIABLE BOOK - AAKASH SERIESCHAPTER - RANDOM VARIABLE EXERCISE - EXERCISE-3.2 (VERY SHORT ANSWER QUESTIONS)
16 Videos
- RANDOM VARIABLE BOOK - AAKASH SERIESCHAPTER - RANDOM VARIABLE EXERCISE - EXERCISE-3.3 (LONG ANSWER QUESTIONS)
10 Videos
- RANDOM VARIABLE BOOK - AAKASH SERIESCHAPTER - RANDOM VARIABLE EXERCISE - PRACTICE EXERCISE
34 Videos
- QUADRATIC EQUATIONS & EXPRESSIONSBOOK - AAKASH SERIESCHAPTER - QUADRATIC EQUATIONS & EXPRESSIONSEXERCISE - PRACTICE EXERCISE
88 Videos
- RANDOM VARIABLESBOOK - AAKASH SERIESCHAPTER - RANDOM VARIABLESEXERCISE - Exercise 4.4
9 Videos
Similar Questions
If X~B(10,0.6) then, find mean and variance of X.
View SolutionThe mean of a Poisson distribution is 2.25. The standard deviation is
View SolutionA random variable X ahs the following distribution
x1234P(X=x)c2c3c4c
The value of c is
View SolutionIf 3x+22x+3=4x+33x+4, then x =
View SolutionA random variable X takes four values with probabilities 1+3x4,(1−x),1+2x4 and 1−4x4. Find the set of values of x for the variable X to have probability distribution.
View Solutionएक यादृच्छिक चर X का निम्नलिखित प्रायिकता बंटन है :
X012345P(X)0.1k0.13k0.3k
k का मान ज्ञात करे
View Solution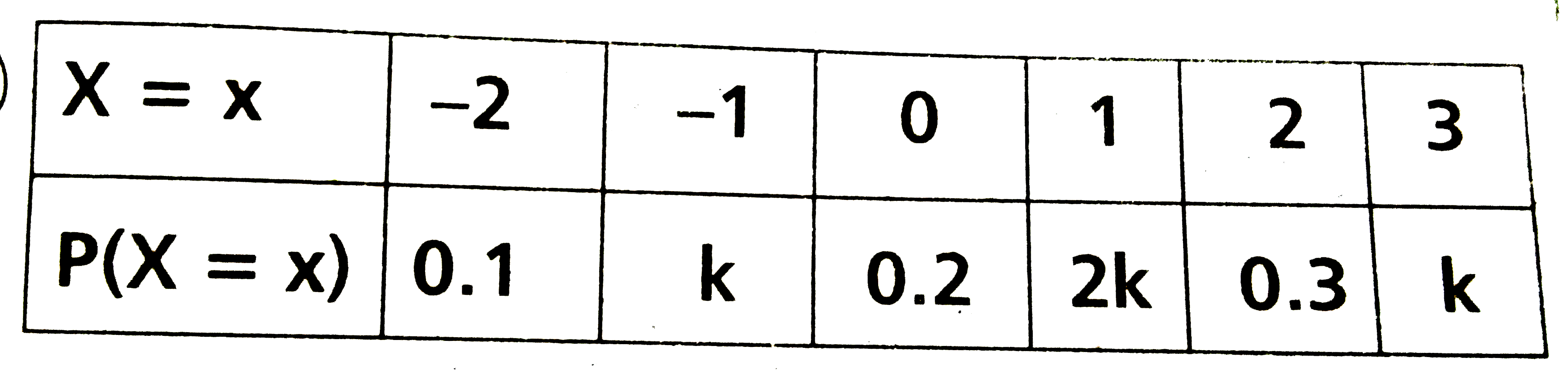
is the probability distribution of a random variable X. Find the value of K and the variance of X.
View SolutionIf the probability function of a random variable X is defined by P(X=k)=a(k+12k) for k=0,1,2,3,4,5 then the probability that X takes a prime value is
View SolutionThe probability distribution of a random variable X is given below, then k=
X=x1234P(X=x)k4k4kk
View SolutionThe range of a random variable X is 1,2,3,..., and P(X=k)=ckk! where k=1,2,3... .Find the value of P(0<X<3)
View SolutionIf the probability function of a random variable X is defined by P(X=k)=a(k+12k) for k=0,1,2,3,4,5, then the probability that X takes a prime value is
View SolutionThe range of random variable X={1,2,3,....∞} and the probabilities are given by P(x=k)=Ck⌊k. Then C=
View SolutionA random variable 'X' has a probability distribution P(X) of the following form (k is constant):

then k = __________ .
View SolutionThe range of random vairable x={1,2,3…..} and the probabilities are given by P(x=k)=ckk! then c =
View SolutionFor a random variable X if P(X=k) = 4(k+15k)a, for k=0,1,2,…then a= _____
View Solution
