A 2.0-kg projectile is fired with initial velocity components vox=30m/s and voy=40m/s from a point on the earth's surface. Neglect any effects due to air resistance.
How much work was done in firing the projectile ?
900J
2500J
1600J
4900J
The correct Answer is:C
To find the work done in firing a projectile, we can use the following steps:
Step 1: Understand the Problem
We have a projectile of mass m=2.0kg that is fired with initial velocity components v0x=30m/s and v0y=40m/s. We need to calculate the work done in firing this projectile until it reaches its highest point.
Step 2: Identify the Forces Acting on the Projectile
The only force acting on the projectile after it is fired is the gravitational force, which acts downward. The gravitational force Fg can be calculated as:
Fg=m⋅g
where g is the acceleration due to gravity (approximately 10m/s2).
Step 3: Calculate the Gravitational Force
Substituting the values:
Fg=2.0kg⋅10m/s2=20N
Step 4: Determine the Maximum Height
To find the maximum height H reached by the projectile, we can use the kinematic equation:
v2y=u2y+2ays
At the maximum height, the final vertical velocity vy=0. Thus, the equation simplifies to:
0=(40m/s)2−2⋅10m/s2⋅H
Rearranging gives:
H=(40m/s)22⋅10m/s2=160020=80m
Step 5: Calculate the Work Done
The work done W against gravity to raise the projectile to its maximum height is given by:
W=Fg⋅H
Substituting the values we have:
W=20N⋅80m=1600J
Conclusion
The work done in firing the projectile is 1600J.
---
We have a projectile of mass m=2.0kg that is fired with initial velocity components v0x=30m/s and v0y=40m/s. We need to calculate the work done in firing this projectile until it reaches its highest point.
The only force acting on the projectile after it is fired is the gravitational force, which acts downward. The gravitational force Fg can be calculated as:
Fg=m⋅g
where g is the acceleration due to gravity (approximately 10m/s2).
Substituting the values:
Fg=2.0kg⋅10m/s2=20N
To find the maximum height H reached by the projectile, we can use the kinematic equation:
v2y=u2y+2ays
At the maximum height, the final vertical velocity vy=0. Thus, the equation simplifies to:
0=(40m/s)2−2⋅10m/s2⋅H
Rearranging gives:
H=(40m/s)22⋅10m/s2=160020=80m
The work done W against gravity to raise the projectile to its maximum height is given by:
W=Fg⋅H
Substituting the values we have:
W=20N⋅80m=1600J
The work done in firing the projectile is 1600J.
Topper's Solved these Questions
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (Matrix-Match)
1 Video
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (Integer Type)
5 Videos
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (More than one choice correct type)
10 Videos
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Practice Questions (Integer Type)
4 Videos
Similar Questions
A projectile is projected in air with initial velocity →v=(3ˆi+4ˆj) m/s from the origin. The equation of trajectory of the projectile is given as (g=−10ˆjms2) (neglect air resistance )
View SolutionA projectile projected with initial velocity 20m/s and at and angle of 30∘ from horizontal. Find the total work done when it will hit the ground.
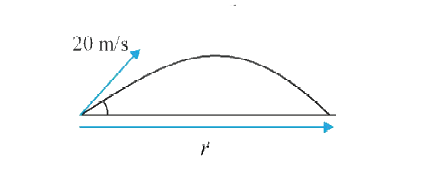
View Solution
Knowledge Check
A 2.0-kg projectile is fired with initial velocity components vox=30m/s and voy=40m/s from a point on the earth's surface. Neglect any effects due to air resistance.
What is the kinetic energy of the projectile when it reaches the highest point in its trajectory ?AzerojoulesB1600JC900JD2500JA projectile is fired with initial momentum p at an angle 45∘ from a point P as shown in figure. Neglecting air resistance, the magnitude of change in momentum between leaving P and arriving at Q is:
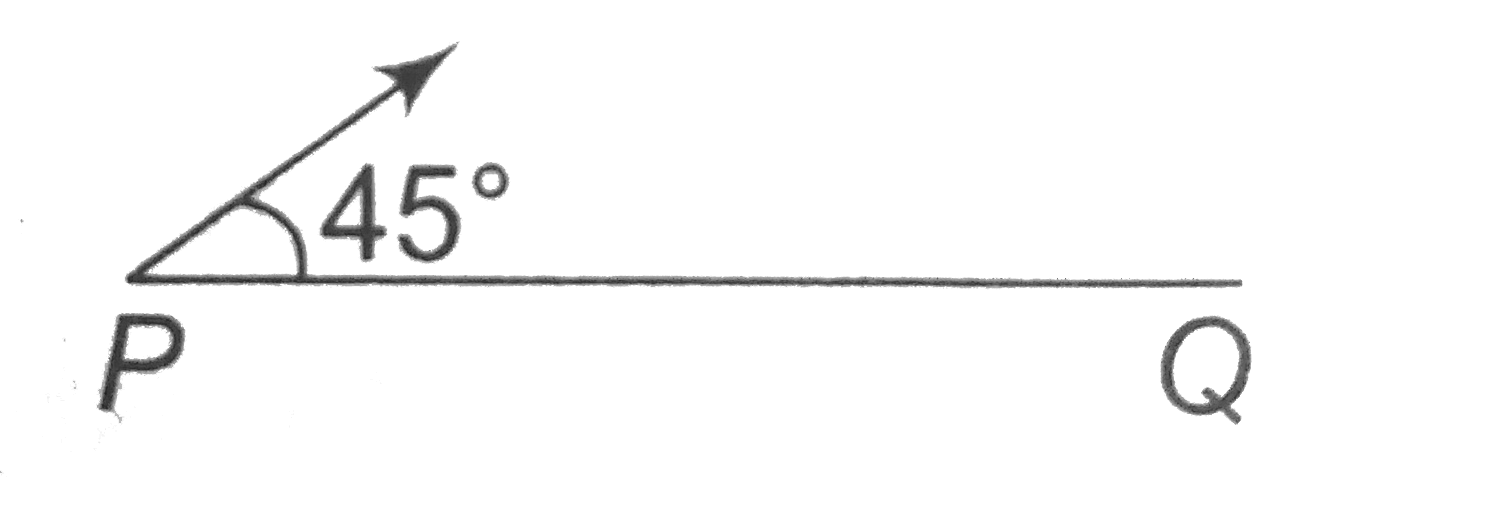 Ap/2Bp√2CpD2p
Ap/2Bp√2CpD2pA projectile is fired horizontally with an initial speed of 20 m/s/ its horzontal speed 3 sec later is:
A20m/sB6.67m/sC60m/sD29.4m/s
A 3.0 kg particle-like object moves in a plane with velocity components vx=30m/sandvy=60m/s as is passes through the point with (x, y) coordinates of (3.0, -4.0) m. Just then, in unit vector notation, what is its angular momentum relative to (a) the origin and (b) the point located at (-2.0, -2.0) m?
View SolutionA projectile is fired from the surface of earth of radius R with a velocity ηve where ve is the escape velocity and η<1. Neglecting air resistance, the orbital velocity of projectile is -
View SolutionA projectile fired from a gun has initial horizontal and vertical components of velocity equal to 30 m/s and 40 m/s, respectively.
What is the magnitude of the projectile's velocity just before it strikes the ground?
View SolutionA projectile fired from a gun has initial horizontal and vertical components of velocity equal to 30 m/s and 40 m/s, respectively.
Approximately how long does it take the projectile to reach the highest point in its trajectory?
View SolutionA projectile is fired from the origin with a velocity v0 at an angle θ with x-axis. The speed of the projectile at an altitude h is .
View Solution
