An outfielder throws a baseball with an initial speed of 83.2 mi/h. Just before an infielder catches the ball at the same level, the ball's speed is 110 ft/s. In foot-pounds, by how much is the mechanical energy of the ball-Earth system reduced of air drag ? (The weight of a baseball is 9.0 oz.)
The correct Answer is:25 ft.lb
To solve the problem, we need to calculate the change in mechanical energy of the ball-Earth system due to air drag. We will do this by calculating the initial and final kinetic energies of the baseball and then finding the difference.
Step 1: Convert the initial speed from miles per hour to feet per second
The initial speed of the baseball is given as 83.2 miles per hour. We need to convert this to feet per second.
1 mile = 5280 feet
1 hour = 3600 seconds
Initial speed (V_i)=83.2mi/h×5280ft1mi×1h3600s
Calculating this gives:
Vi=83.2×52803600≈122.0ft/s
Step 2: Calculate the mass of the baseball in pounds
The weight of the baseball is given as 9.0 oz. We need to convert this to pounds.
1 pound = 16 ounces
Weight in pounds=9.0oz16oz/lb=0.5625lb
Step 3: Calculate the initial kinetic energy (KE_initial)
The formula for kinetic energy is:
KE=12mv2
Where:
- m = mass in pounds
- v = speed in feet per second
Using the initial speed:
KEinitial=12×0.5625lb×(122.0ft/s)2
Calculating this gives:
KEinitial=12×0.5625×14884≈4184.25ft-lb
Step 4: Calculate the final kinetic energy (KE_final)
Using the final speed of 110 ft/s:
KEfinal=12×0.5625lb×(110.0ft/s)2
Calculating this gives:
KEfinal=12×0.5625×12100≈3403.125ft-lb
Step 5: Calculate the reduction in mechanical energy due to air drag
The reduction in mechanical energy is given by the difference between the initial and final kinetic energies:
Reduction in energy=KEinitial−KEfinal
Calculating this gives:
Reduction in energy=4184.25ft-lb−3403.125ft-lb≈781.125ft-lb
Final Answer
The mechanical energy of the ball-Earth system is reduced by approximately 781.125 foot-pounds due to air drag.
---
The initial speed of the baseball is given as 83.2 miles per hour. We need to convert this to feet per second.
1 hour = 3600 seconds
The weight of the baseball is given as 9.0 oz. We need to convert this to pounds.
The formula for kinetic energy is:
- m = mass in pounds
- v = speed in feet per second
Using the final speed of 110 ft/s:
The reduction in mechanical energy is given by the difference between the initial and final kinetic energies:
The mechanical energy of the ball-Earth system is reduced by approximately 781.125 foot-pounds due to air drag.
Topper's Solved these Questions
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (Single Correct Choice Type)
59 Videos
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (More than one choice correct type)
10 Videos
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - CHECKPOINT
6 Videos
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Practice Questions (Integer Type)
4 Videos
Similar Questions
A 60 kg skier leaves the end of a ski-jump ramp with a velocity of 27 m/s directed 25∘ above the horizontal. Suppose that as a result of air drag the skier returns to the ground with a speed of 22 m/s, landing 14 m vertically below the end of the ramp. From the launch to the return to the ground, by how much is the mechanical energy of the skier-Earth system reduced because of air drag ?
View SolutionIn Fig. 2-13 , a pitcher tosses a baseball up along a y axis, with an initial speed of 12 m/s.
(a) How long does the ball take to reach its maximum height ?
View Solution
Knowledge Check
The coach throws a baseball to a player with an initial speed at 20ms−1 at an angle of 45∘ with the horizontal.At the moment the ball is thrown, the player is 50m from coach.The speed and the direction that the player has to run to catch the ball at the same height at which it was released in ms−1 is
A5√2away from coachB5√2 towards from coachC√25towards the coachD√25away from the coachA basketball player is running at a constant speed of 2.5 m/s when a player tosses a basketball upward with a speed of 6.0 m/s. How far does the player run before he catches the ball? Ignore air resistance.
A3.1 mB4.5 mCThe ball cannot be caught because it will fall behind the player.D6.0 mA baseball is hit into the air at an initial speed of 36.6 m/s and an angle of 50.0∘ above the horizontal. At the same time, the center fielder starts running away from the batter, and he catches the ball 0.914 m above the level at which it was hit. If the center fielder is initially 1.10×102m from home plate, what must be his average speed?
A3.5 m/sB5.0 m/sC4.2 m/sD6.9 m/s
The coach throws a baseball to a player with an initial speed of 20m/s at an angle of 45∘ with the horizontal. At the moment the ball is thrown, the player is 50 m from the coach. At what speed and in what direction must the player run to catch the ball at the same height at which it was released? (g=10m/s2).
View SolutionA boy throws a ball vertically upward with an initial speed of 15.0m/s.The ball was released when it was at 2.00m above ground. The boy catches it at the same point as the point of projection
(a) What is maximum height reaches by the ball ?
How long is the ball in the air?
View SolutionA player throwsa a ball upwards with an initial speed of 29.4ms−1.
(i) What is the direction of acceleration during the upwared motion of the ball?
(ii) What are the velocity and acceleration of the ball at the highest point of its motion?
(iii) Choose the x=0 and t=0 to be the location and time of the ball at its highest point, vertically downward direction to be the positive direction of X-axis, and give the signs of positive, velocity and acceleration of the ball during its upward, and downward motion.
(iv) To what height does the ball rise and after how long does the ball return to the player's hand?( Take g=9.8ms−2, and neglect air resistance).
View SolutionA ball is projected vertically up from the floor of a room. The ball experiences air resistance that is proportional to speed of the ball. Just before hitting the ceiling the speed of the ball is 10 m/s and its retardation is 2g. The ball rebounds from the ceiling without any loss of speed and falls on the floor 2s after making impact with the ceiling. How high is the ceiling? Take g=10m/s2.
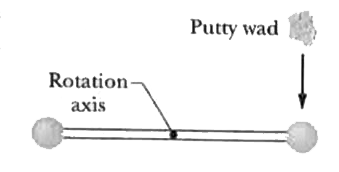
View SolutionTwo 2.00 kg balls are attached to the ends of a thin rod of length 50.0 cm and negligible mass. The rod is free to rotate in a vertical plane without friction about a horizontal axis through its center. With the rod initially horizontal (Fig. 10-87), a 50.0 g wad of wet putty drops onto one of the balls, hitting it with a speed of 3.00 m/s and then sticking to it. (a) What is the angular speed of the system just after the putty wad hits? (b) What is the ratio of the kinetic energy of the system after the collision to that of the putty wad just before? ( c) Through what angle will the system rotate before it momentarily stops?
View Solution
