Vector →A is 2 cm long and is 60° above the x - axis in the first quadrant, vector →B is 2cm long and is 60° below the x - axis in the fourth quadrant. Find →A+→B Solution in Telugu
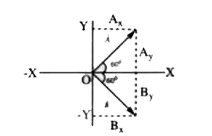
→R=→A+→B
→R=2cos60∘ˆi+2sin60ˆj+2cos60ˆi−2sin60ˆj
→R=4cos60ˆi=2ˆi
∴2cm, along x-axis.
Topper's Solved these Questions
- MOTION IN A PLANEBook:AAKASH SERIESChapter:MOTION IN A PLANEExercise:SHORT ANSWER TYPE QUESTIONS
Explore 6 Videos
- MOTION IN A PLANEBook:AAKASH SERIESChapter:MOTION IN A PLANEExercise:VERY SHORT ANSWER TYPE QUESTIONS
Explore 10 Videos
- MOTION IN A PLANEBook:AAKASH SERIESChapter:MOTION IN A PLANEExercise:EXERCISE-3 (Circular Motion)
Explore 7 Videos
- MOTION IN A STRAIGHT LINE Book:AAKASH SERIESChapter:MOTION IN A STRAIGHT LINE Exercise:very Short answer type question
Explore 15 Videos
Similar Questions
Show that the trajectory of an object thrown at certain angle with the horizontal is a parabola .
View SolutionWhat is the acceleration of a projectile at the top of its trajectory ?
View SolutionA particle ‘P is moving in a circle of radius "r" with uniform speed v. AB is the diameter of circle and 'C' is the centre. The angular velocity of P about A and C are in the ratio.
View SolutionGive two examples of the motion of big objects where the object can be treated as a particle and where it cannot be?
View SolutionVector vec(A) is 2 cm long and is 60^(@) above the x-axis in the first quadrant. Vactor vec(B) is 2 cm long and is 60^(@) below the x-axis in the fourth quadrant. The sum vec(A)+vec(B) is a vector of magnitudes
View SolutionIf ∣∣→a+→b∣∣=60,∣∣→a−→b∣∣=40and|→a|=22 then find ∣∣→b∣∣.
View SolutionLet →aand→b are two vectors inclined at an angle of 60∘,If|→a|=∣∣→b∣∣=2 ,the the angle between →aand→a+→b is
View Solutionvectors →aand→b are inclined at an angle θ=60∘. If |→a|=1,∣∣→b∣∣=2, then [(→a+3→b)×(3→a−→b)]2 is equal to
View Solutionसदिश →a तथा →b सरीख नहीं हैं| यह ज्ञात करें x के किस मान के लिए सदिश →c=(x−2)→a+→b तथा →d=(2x+1)→a−→b हैं|
View SolutionIf →a and →b are two vectors of magnitude 2 and inclined at an angle 60∘ , then the angle between →a and →a+→b is
View SolutionIf ∣∣→a+→b∣∣=60∣∣→a−→b∣∣=40and∣∣→b∣∣=46 find |→a|
View SolutionIf →aand→b are two vectors of magnitude 2 and inclined at an angle 60∘ then the angle between →aand→a+→b is
View SolutionVector →A is 2 cm long and is 600 above the x-axis in the first quadrant, vector B is 2cm long and is 600 below the x-axis in the fourth quadrant. Find →A+→B
View Solutionयदि →A=ˆi−ˆj+ˆk है (→A+→B) x- अक्ष के अनुदिश एकांक सदिश है तो →B होगा
View SolutionVector →A s 2cm long and is 60∘ above the x-axis in the first quadrant, vector →B is 2 cm long and is 60∘ below the x-axis is the fourth quadrant. Find →A+→B.
View Solution