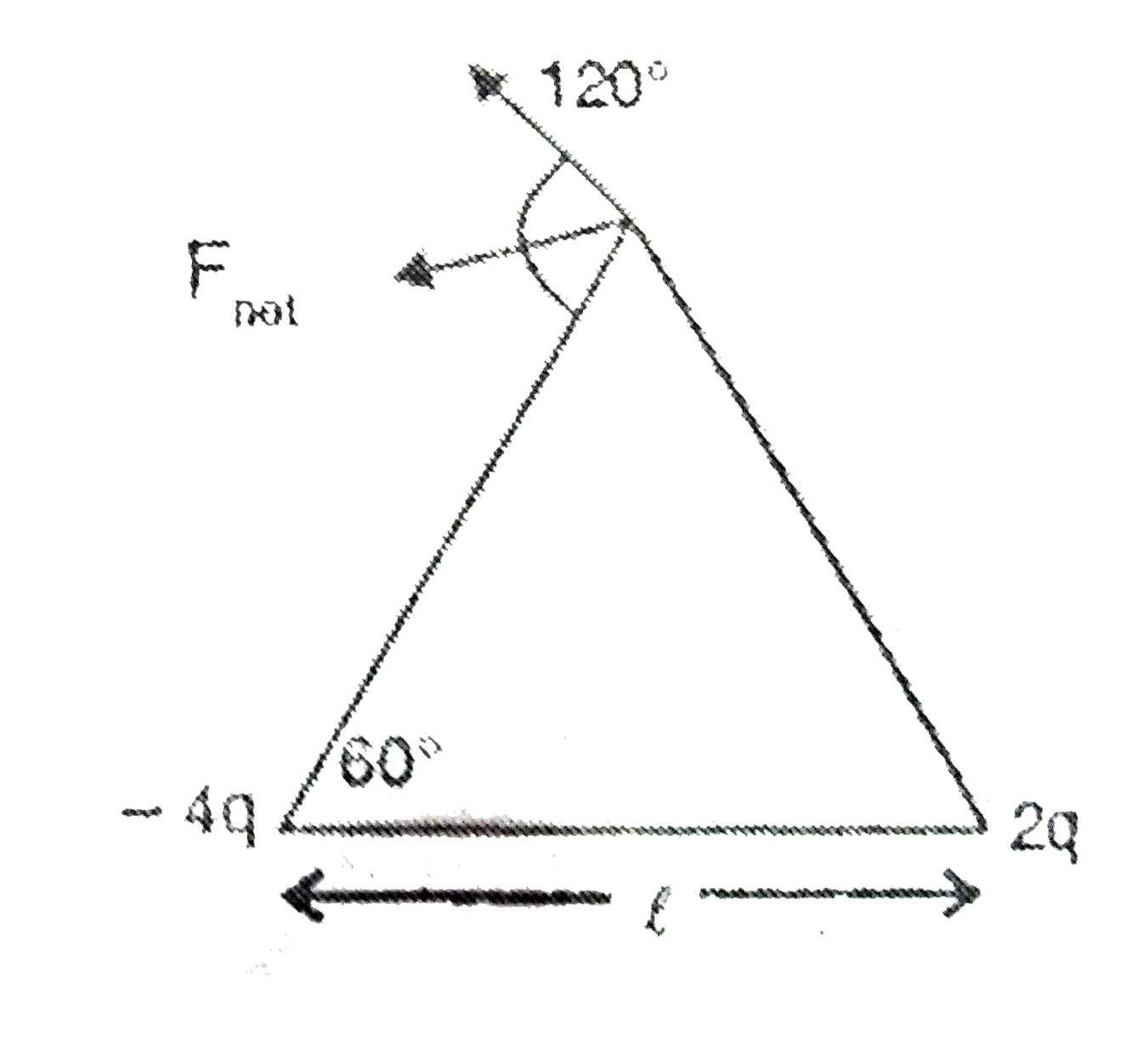
(a) Three point charges q,-4q and 2q are placed at the vertices of an equilateral triangle ABC of side 'l' as shown in the figure. Obtain the expression for the magnitude of the resultant electric force acting on the charge q.
(b) Find out the amount of the work 4one to separate the charges at infinite distance.

(a) F1=k2q2l2
F2=4q2l2
Fnet=√F21+F22+2F1F2cos120∘
Fnet=√F21−F22−F1F2
(b) Work done required to separate the charge at infinity equal to electric potential energy of system.
w1=kq2ql w2=k(−4q)−ql
w3=k(−4q)(2q)l w=w1+w2+w3
w=2kq2l−4kq2l−8kq2l w=−10kq2l
Topper's Solved these Questions
- XII BOARDSBook:XII BOARDS PREVIOUS YEARChapter:XII BOARDSExercise:SECTION - A
Explore 7 Videos
- XII BOARDSBook:XII BOARDS PREVIOUS YEARChapter:XII BOARDSExercise:SECTION - B
Explore 9 Videos
- XII BOARDSBook:XII BOARDS PREVIOUS YEARChapter:XII BOARDSExercise:SECTION - E
Explore 6 Videos
- SAMPLE PAPER 2019Book:XII BOARDS PREVIOUS YEARChapter:SAMPLE PAPER 2019Exercise:SECTION D
Explore 6 Videos
Similar Questions
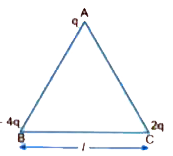
(a) Three point charges q,-4q and 2q are placed at the vertices of an equilateral triangle ABC of side 'l' as shown in the figure. Obtain the expression for the magnitude of the resultant electric force acting on the charge q.
(b) Find out the amount of the work done to separate the charges at infinite distance.

View SolutionThree point charges q,−4q and 2q are placed at the vertices of an equilateral triangle ABC of side 'l' as shown in the Fig. Obtain the experssion for the magnitude of the resultant electric force acting on the charge q.
View Solution
Knowledge Check
Three charges 2q,−q,and−q are located at the vertices of an equilateral triangle. At the center of the triangle,
Athe field is zero but potential is nonzero.Bthe field is nonzero but potential is zero.Cboth field and potential are zero.Dboth field and potential are nonzero.Three charges Q,(+q) and (+q) are placed at the vertices of an equilateral triangle of side l as shown in the figure. It the net electrostatic energy of the system is zero, then Q is equal to
 A(−q2)B(−q)C(+q)DZero
A(−q2)B(−q)C(+q)DZeroThree point charges q are placed at three vertices of an equilateral triangle of side a. Find magnitude of electric force on any charge due to the other two.
A(√34πε0)(qa)2B(√24πε0)(qa)2C(√34πε0)(q2a)2D(√24πε0)(2qa)2
Two point charges +q and -2qare placed at the vertices B and C of an equilateral triangle ABC of side 'a' as shown in Obtain the expression for
The magnitude ,
(b)
The direction of the resultant electric field at the vertex A due to these two charges.
(c)
View SolutionTwo point charges +q and -2q are placed at the vertices B and C of an equilateral triangle ABC of side 'a' as shown Obtain the expession for (i) the magnitude ,and (ii) the direction of the resultant electric field at the vertex A due to these two charges .
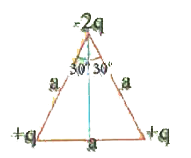
View SolutionThree points charges =q, -2q, +q are arranged on the vertices of an equilaterial triangle as shown in the figure. Find he dipole moment of the system.
View SolutionTwo point charges +6qand−8q are placed at the vertices B and C of an equilateral triangle ABC of side a. Obtain an expression for magnitude and direction of resultant electric field at the vertex A due to these two charges.
View SolutionConsider the charges q,q and -q placed at the vertices of an equilateral triangle of each side l. What is the force on each charge ?
View Solution